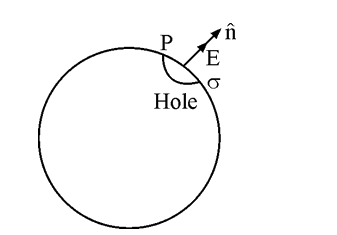
A hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the holes is (σ/2∈0 ˆn, where ˆn is the unit vector in the outward normal direction, and σ is the surface charge density near ther hole.
The correct Answer is:B
Surface charge density near the hole =σ . Unit vector n (normal directed outwards).
Let P be the point on the hole. The electric field at Point P closed to the surface of conductor, according to Gauss's theorem,
∫→E.¯¯¯¯ds=qe0
(∵=qds⇒q=0 ds, where ds= area) where, q is the charge near the hole. Edscosθ=adse0
∵ Angle between electric field and area vector is 0∘ ∴Eds=adse0=E=σe0⇒E=0e0n
This electric field is due to the filled up hole and the field due to the rest of the charged conductor. The two fields inside the conductor are equal and opposite. So, there is no electric field inside the conductor. Outside the conductor, the electric field at P due to each part =12E=σ2e0¯n
Similar Questions
A hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the holes is (σ/2∈0 ˆn, where ˆn is the unit vector in the outward normal direction, and σ is the surface charge density near ther hole.
View SolutionA hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the hole is (σ2∈0)ˆn , where ˆn is the unit vector in the outward normal direction, and σ is the surface charge density near the hole,
View Solution
Knowledge Check
- Question 1 - Select One
The electric intensity at a point near a charged conductor of surface charge density σ is
AE=σ/∈0kBE=σ∈0kCE=σ/2∈0kDE=σ2 - Question 2 - Select One
A spherical charged conductor has σ as the surface density of charge. The electric field on its surface is E. If the radius of the sphere is doubled keeping the surface density of charge unchanged, what will be the electric field on the surface of the new sphere -
AE4BE2CED2E
Show that the normal component of electrostatic field has a discontinuly form one side of a charged. Surface to another given by (−→E2−−→E1).ˆn=σ∈0
where ˆn is a unit vector normal to the surface at a point and σ at a point and σ is the surface charge density at that point. (The direction of ˆn is from side 1 to side 2). Hence show that justy outside a conductor, the electric field σˆn/∈0.
(b) Show that the tangential componet of electrostatic field is contionous from one side fo a charged surface to another.View SolutionShow that the normal component of electrostatic field has a discontinuity from one side of a charged surface to another given by
(→E2−→E1).Htan=σε0
where ˆn is a unit vector normal to the surface at a point and o is the surface charge density at that point. (The direction of în is from side 1 to side 2). Hence, show that just outside a conductor, the electric field is σε0ˆn.View SolutionShow that the electric field at the surface of a charged conductor is given by →E=σε0ˆn , where σ is the surface charge density and ˆn is a unit vector normal to the surface in the outward direction .
View SolutionWhat is the direction of the electric field at the surface of a charged conductor having charge density σ <0?
View SolutionShow that the electric field at the surface of a charged conductor is given by →E=σ60ˆn , where σ is the surface charge density and ˆn is a unit vector normal to the surface in the outward direction.
View SolutionThe surface charge density of a thin charged disc of radius R is σ. The value of the electric field at the centre of the disc is σ2∈o. With respect to the field at the centre, the electric field along the axis at a distance R from the centre of the disc reduces by
View Solution

