. In the given figure, two equal circles, with centres O and O', touch each other at X. OO' produced me the circle with centre O' at A. AC is tangent to the circle with centreO, at the point C. O'D is perpendicular to AC. Find the value of DO'CO.
∠ADO’=900( tangent to the circle is perpendicular to the radius through the point of contact )
In△ADO’and/_\ACO<br>/_ADO’ = /_ACO(⊥hare900)<br>/_DAO’=/_CAO(commonp∮)<br>:. /_AO’D=/_AOC<br>BY∀Acriterionof≅ruence /_\ADO’ and /_\ACOare≅ruent<br>:. (AO’)/(AO) = (DO’)/(CO)<br>And AO= AO’ + O’X + OX <br>:. AO= 3AO’ ( asAO’=O’X=OXare radius of the two equal circles)
∴AO’3(AO’)=DO’CO
∴13=DO’CO
∴DO’CO=13
Topper's Solved these Questions
Similar Questions
AC is tangent to O, O'D is ⊥ to AC, Find the value of DO'CO
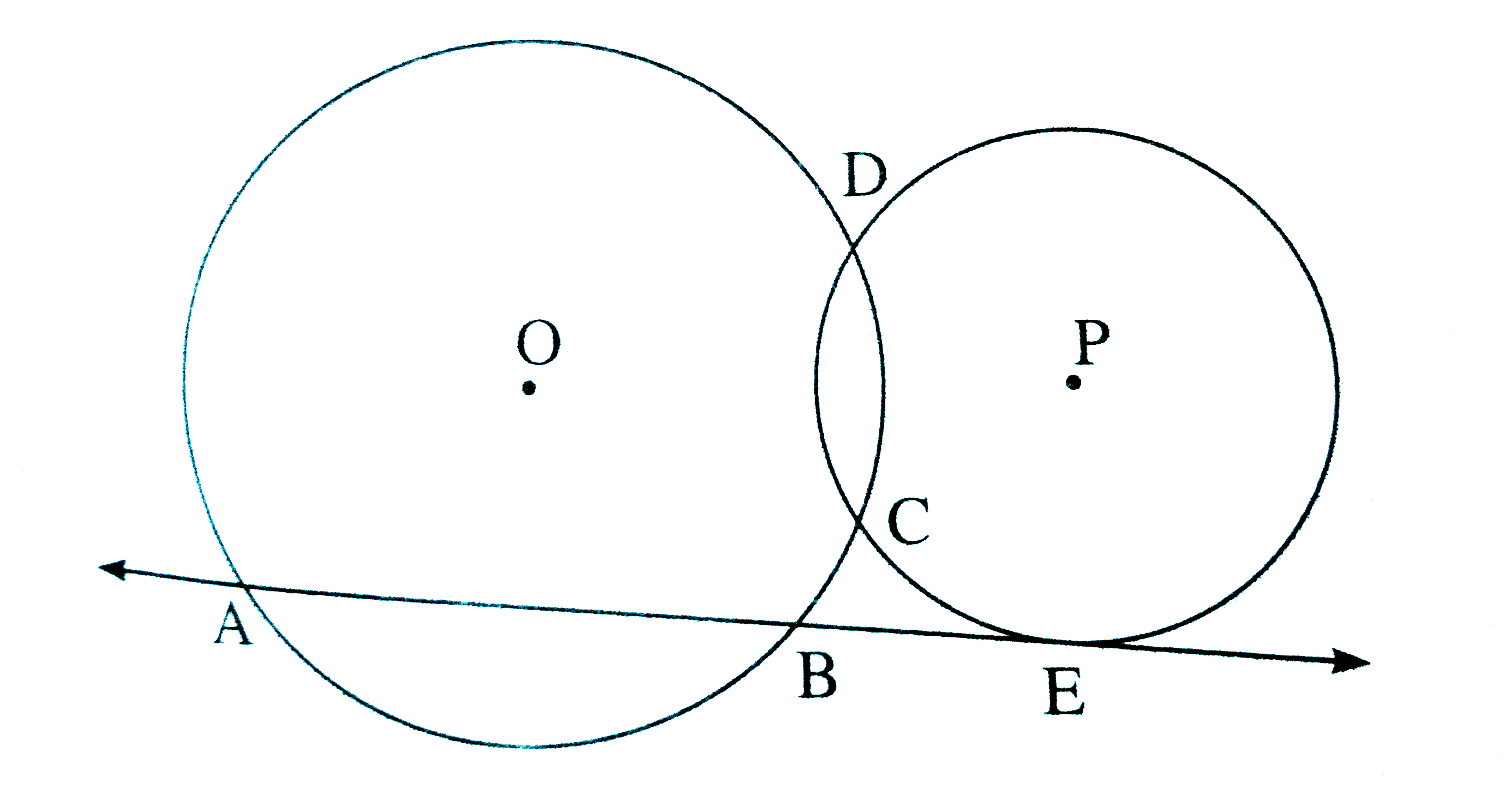
View SolutionIn the figure, two circles with centers O and P intersect each other at points D and C. Line AB intersects the circle with centre O at points A and B and touches the circle with centre P at point E. Prove ∠ADE+∠BCE=180∘.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
AB and AC are tangents to the circle with centre O. Then x equals.
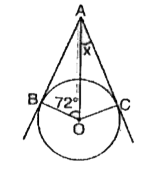 A22∘B18∘C20∘D36∘
A22∘B18∘C20∘D36∘ - Question 2 - Select One
In the given figure, AC is the diameter of the circle with centre O. Chord BD is perpendicular to AC. Express p in terms of x.
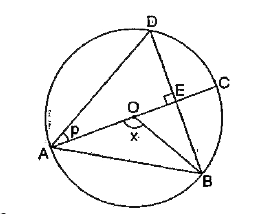 Ax/2B90∘+x/2C90∘−x/2D180∘−x
Ax/2B90∘+x/2C90∘−x/2D180∘−x - Question 3 - Select One
Two circles of same radius r centred at O and O' touch each other at P as shown in the figure, if OO' is produced to meet the circle C(O'r) at A and AT is a tangent to the circle C(O,r) such that O'Q ⊥ AT . Then AO : AO'=
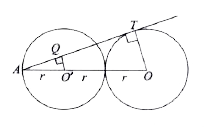 A32B2C3D14
A32B2C3D14
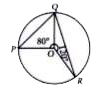
In the given figure, if O is centre of circle, then ∠PQR=
 View Solution
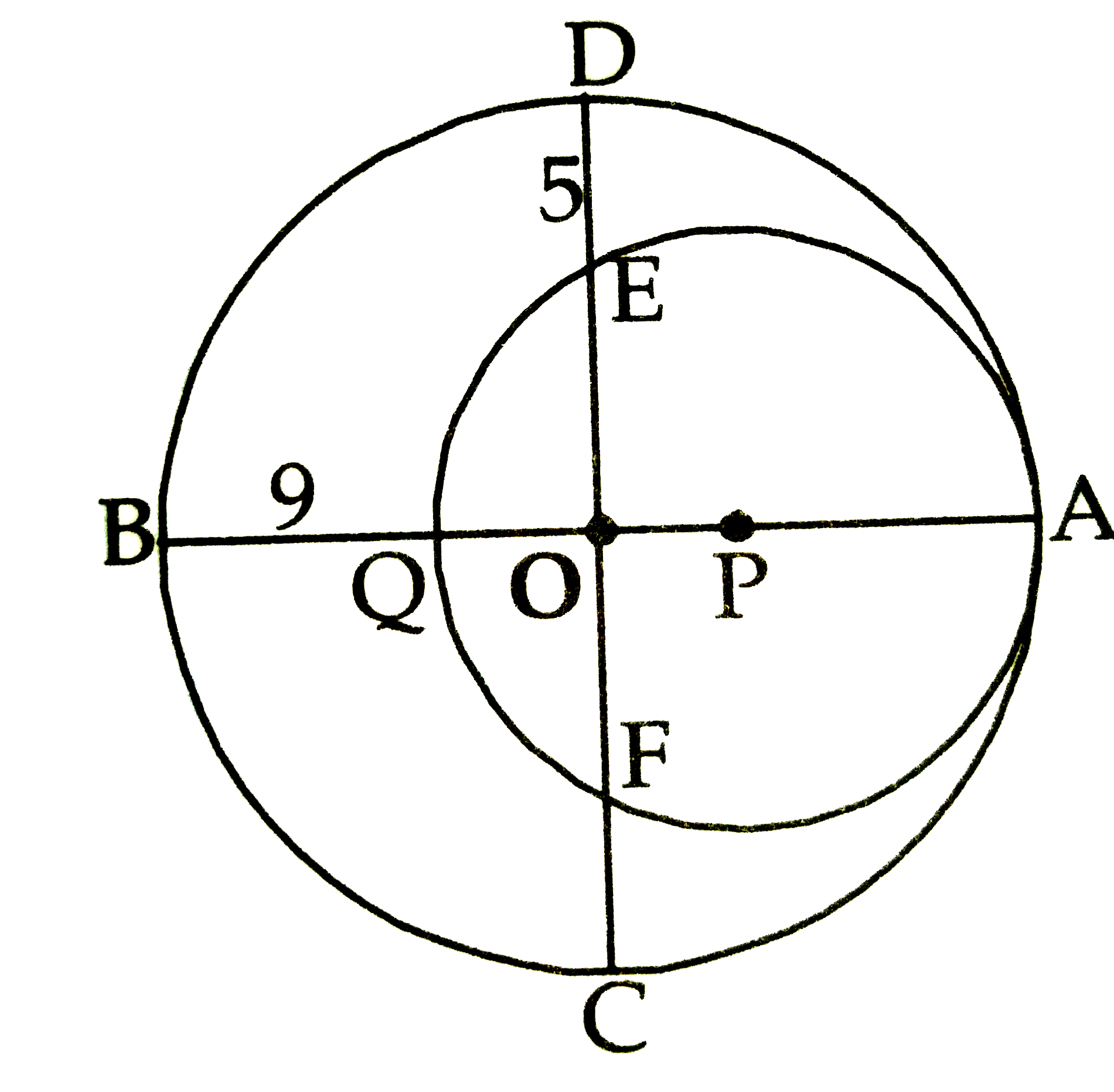
View SolutionIn the given figure, two circle with centres O and P are touching internally at point A.
If BQ= 9, DE= 5, complete the following activity to find the radii of the circle.
 View Solution
View SolutionIn the given figure, O is the centre of the circle.

Name all chords of the circle.View SolutionIf Fig , AOB is diameter of a circle with centre O and AC is a tangent to the circle at A . If ∠BOC=130∘ , then find ∠ACO .
 View Solution
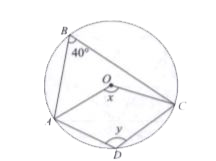
View SolutionIn the given figures, value of y (O is the centre of the circle) is
 View Solution
View Solution

