Question

Collision cross-section is an area of an imaginary sphere of radius $\sigma$ around the molecule within which the centre of another molecule cannot penetrate.
The volume swept by a single molecule in unit time is

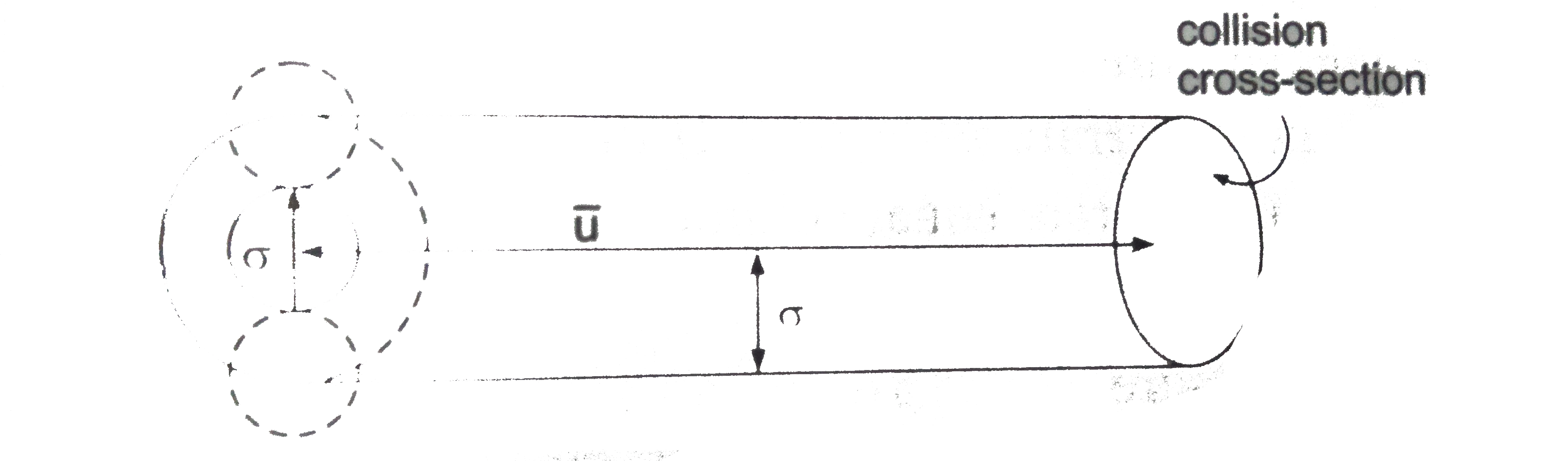
$V=\left(\pi {\sigma }^2\right)\overline{u}$ where $\overline{u}$ is the average speed
If $N^{\ast }$ is the number of molecules per unit volume, then the number of molecules within the volume V is
$N=V{N}^{\ast }=\left(\pi {\sigma }^2\overline{u}\right)N^{\ast }$
Hence, the number of collision made by a single molecule in unit time will be
$Z=N=\left(\pi {\sigma }^2\overline{u}\right)N^{\ast }$
In order to account for the movements of all molecules, we must consider the average velocity along the line of centres of two coliding molecules instead of the average velocity of a single molecule . If it is assumed that, on an average, molecules collide while approaching each other perpendicularly, then the average velocity along their centres is $\sqrt{2}\overline{u}$ as shown below.

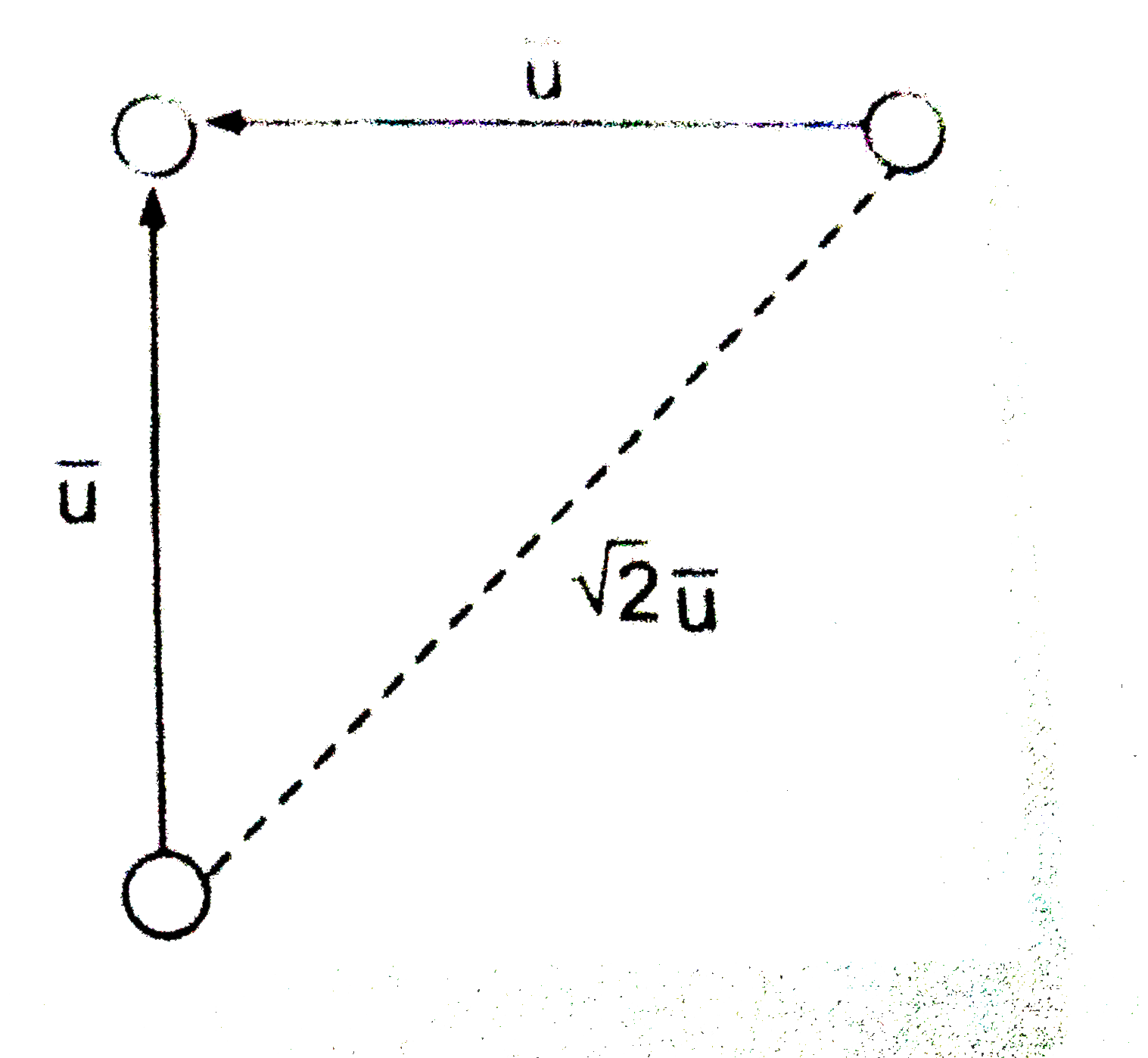
Number of collision made by a single molecule with other molecule per unit time is given by
$Z_1=\pi {\sigma }^2\left({\overline{u}}_{\text{rel}}\right)N^{\ast }=\sqrt{2}\pi {\sigma }^2\overline{u}{N}^{\ast }$
The total number of bimolecular collisions $Z_{11}$ per unit volume per unit time is given by
$Z_{11}=\frac{1}{2}\left(Z_1{N}^{\ast }\right)\text{or}{Z}_{11}=\frac{1}{2}\left(\sqrt{2}\pi {\sigma }^2\overline{u}{N}^{\ast }\right)N^{\ast }=\frac{1}{\sqrt{2}}\pi {\sigma }^2\overline{u}{N}^{\ast 2}$
If the collsion involve two unlike molecules then the number of collisions $Z_{12}$ per unit volume per unit time is given as
$Z_{12}=\pi {\sigma }_{12}^2\left(\sqrt{\frac{8kT}{\pi \mu }}\right)N_1{N}_2$
where $N_1$ and $N_2$ are the number of molecules per unit volume of the two types of molecules, ${\sigma }_{12}$ is the average diameter of the two molecules and $\mu$ is the reduced mass. The mean free path is the average distance travelled by a molecule between two successive collisions. We can express it as follows :
$\lambda =\frac{\text{Average distance travelled per unit time}}{\text{NO. of collisions made by a single molecule per unit time}}=\frac{\overline{u}}{Z_1}$
$\text{or}\lambda =\frac{\overline{u}}{\sqrt{2}\pi {\sigma }^2\overline{u}{N}^{\ast }}\Rightarrow \frac{1}{\sqrt{2}\pi {\sigma }^2\overline{u}{N}^{\ast }}$
For a given gas the mean free path at a particular pressure is :

Options

A. independent to temperature
B. decreases with rise in temperature
C. increases with rise in temperature
D. directly proportional to `T^(2)`

Answer

c