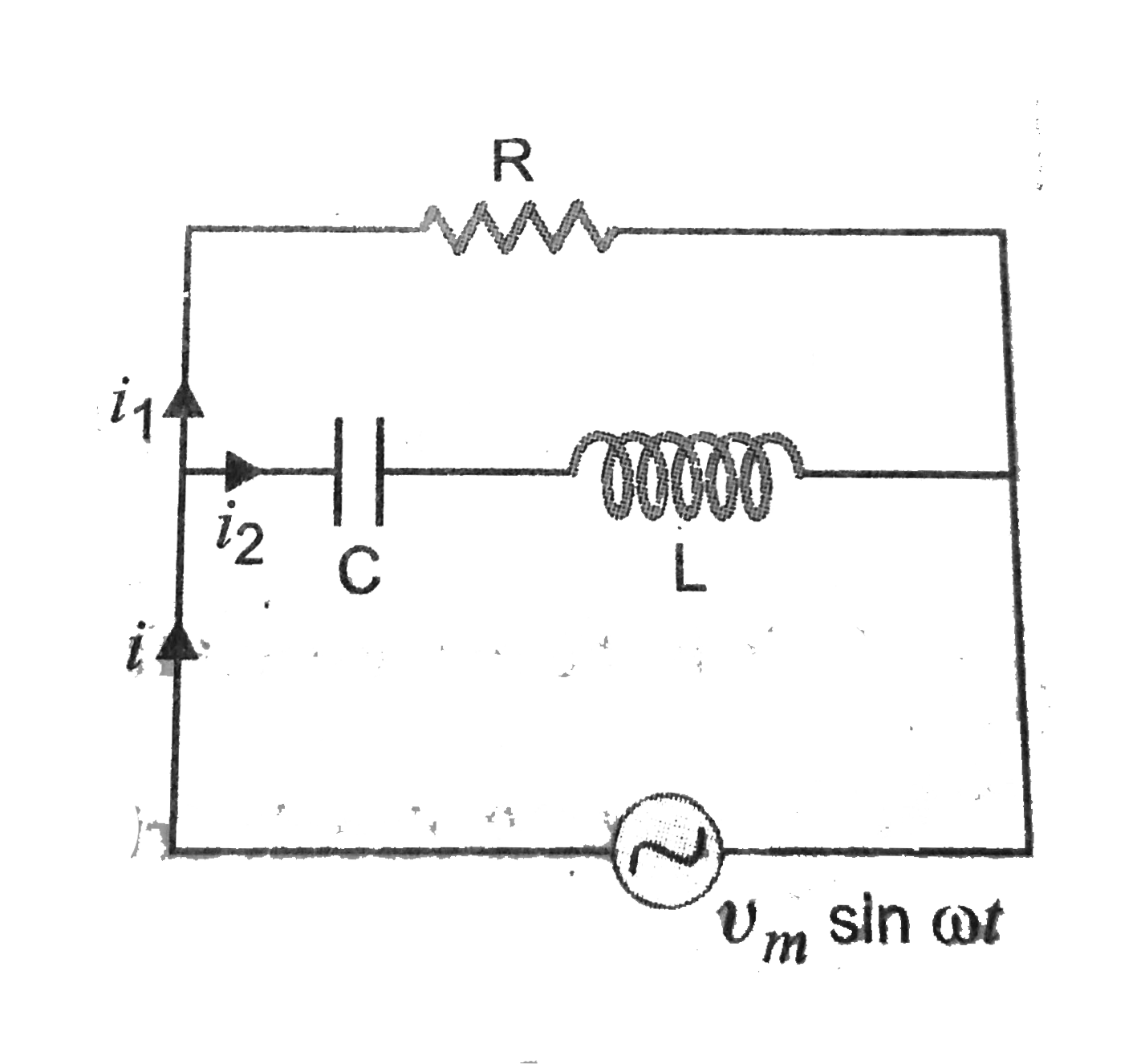
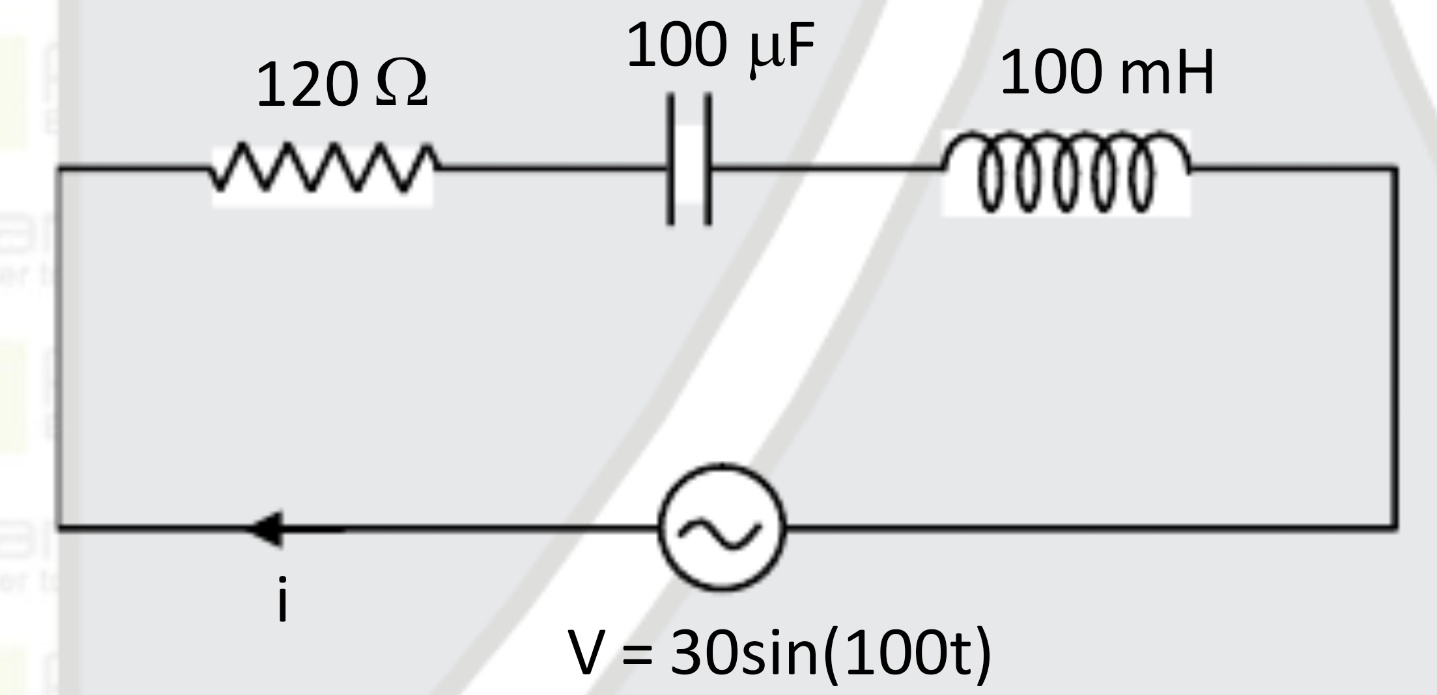
Consider the L-C-R circuit shown in the fiure. Find the net current I and the phase of i. Show that i=VZ. Find the impedance Z of this circuit.

Text Solution
The correct Answer is:A
In the given figure i is the total current from the source. It is divided into two parts, i1 through R and i2 throgh series combination of C and L.
So, we can write i=i1+i2
Vmsinωt=Ri1 (from the circuit diagram)
i1=VmsinωtR.............(i)
If q2 is charge on the capacitor at any time t, then for series combination of C and L.
Applying KVL inthe Lower circuit as shown.

q2C+Ldi2dt−Vmsinωt=0
⇒q2C+Ld2q2(dt)2=Vmsinωt [∴i2=dq2dt]......(ii)
Let q2=qmsin(ωt+ϕ)..............(iii)
dq2dt=qmcos(ωt+ϕ)
⇒d2q2dt2=−qmω2sin(ωt+ϕ)
Now putting the values in Eq. (ii), we get
qm[1C+l(−ω2)]sin(ωt+ϕ)=Vmsinωt
If ϕ=0 and (1C−Lω6(2))>0,
then qm=vm1C−Lω2..............(iv)
From Eq. (iii), i2=dq2dt=ωqmcos(ωt+ϕ)
Using Eq. (iv), i2=ωVmcos(ωt+ϕ)1C−Lω2
Taking ϕ=0,i2=Vmcos(ωt)1ωC−Lω
From Eqs. (i) and (v), we find that i1 and i2 are out of phase by ϕ2
Now, i1+i2=VmsinωtR+Vmcosωt1ωC−Lω
Put VmR=A=Ccosϕ and Vm1ωC−Lω=B=Csinϕ
∴i1+i2=Ccosϕsinωt+Csinϕcosωt
=C=√A2+B2
and ϕ=tan−1BAC=⎡⎣V2mR2+V2m1ωC−Lω⎤⎦1/2
and ϕ=tan−1=R1ωC−Lω
Hence, i=i1+i2=⎡⎢
⎢
⎢⎣V2mR2+V2m(1ωC−Lω)2⎤⎥
⎥
⎥⎦1/2sin(ωt+ϕ)
or iVm=1Z=⎡⎢
⎢
⎢⎣1R2+1(1ωC−Lω)2⎤⎥
⎥
⎥⎦1/2
This is the expression for impedance Z of the circuit.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
Find the current I in circuit
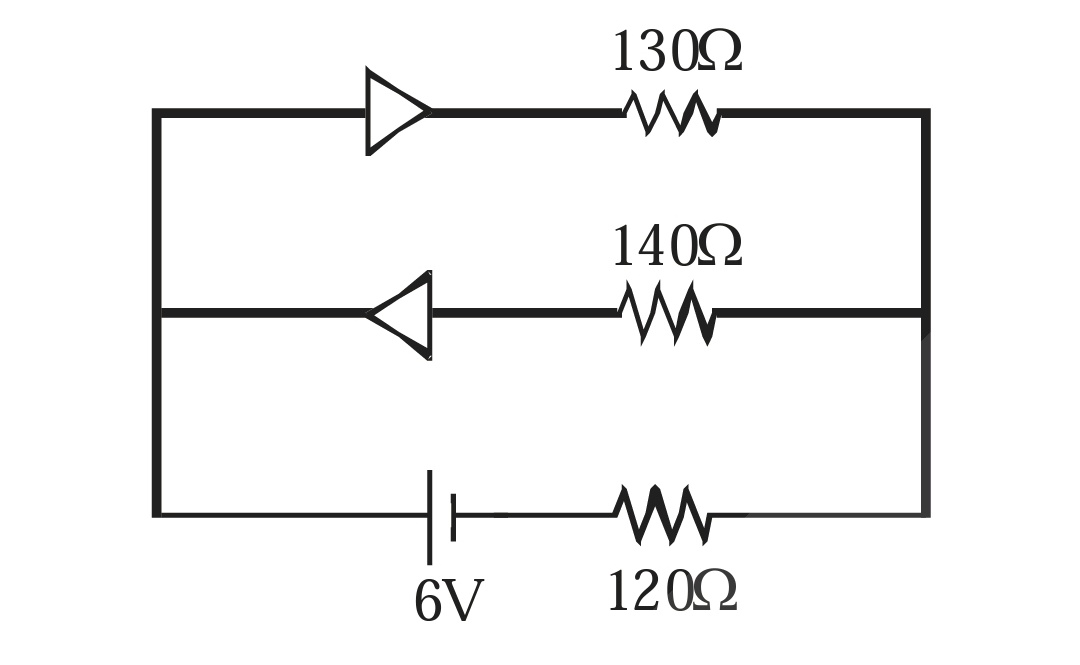 A20 mAB2 mAC0.1 mAD10 mA
A20 mAB2 mAC0.1 mAD10 mAFind the current I in the given circuit
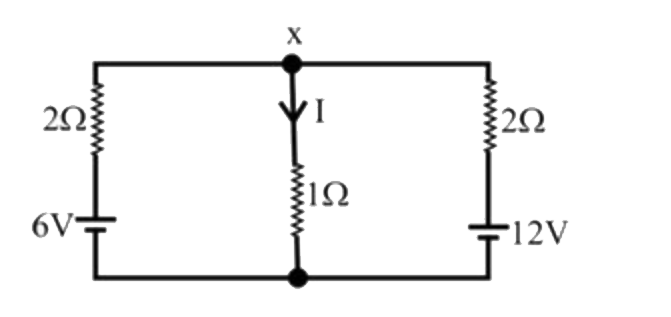 A2AB4. 5 AC6AD1.5 A
A2AB4. 5 AC6AD1.5 AFind current (i) in circuit shown in figure.
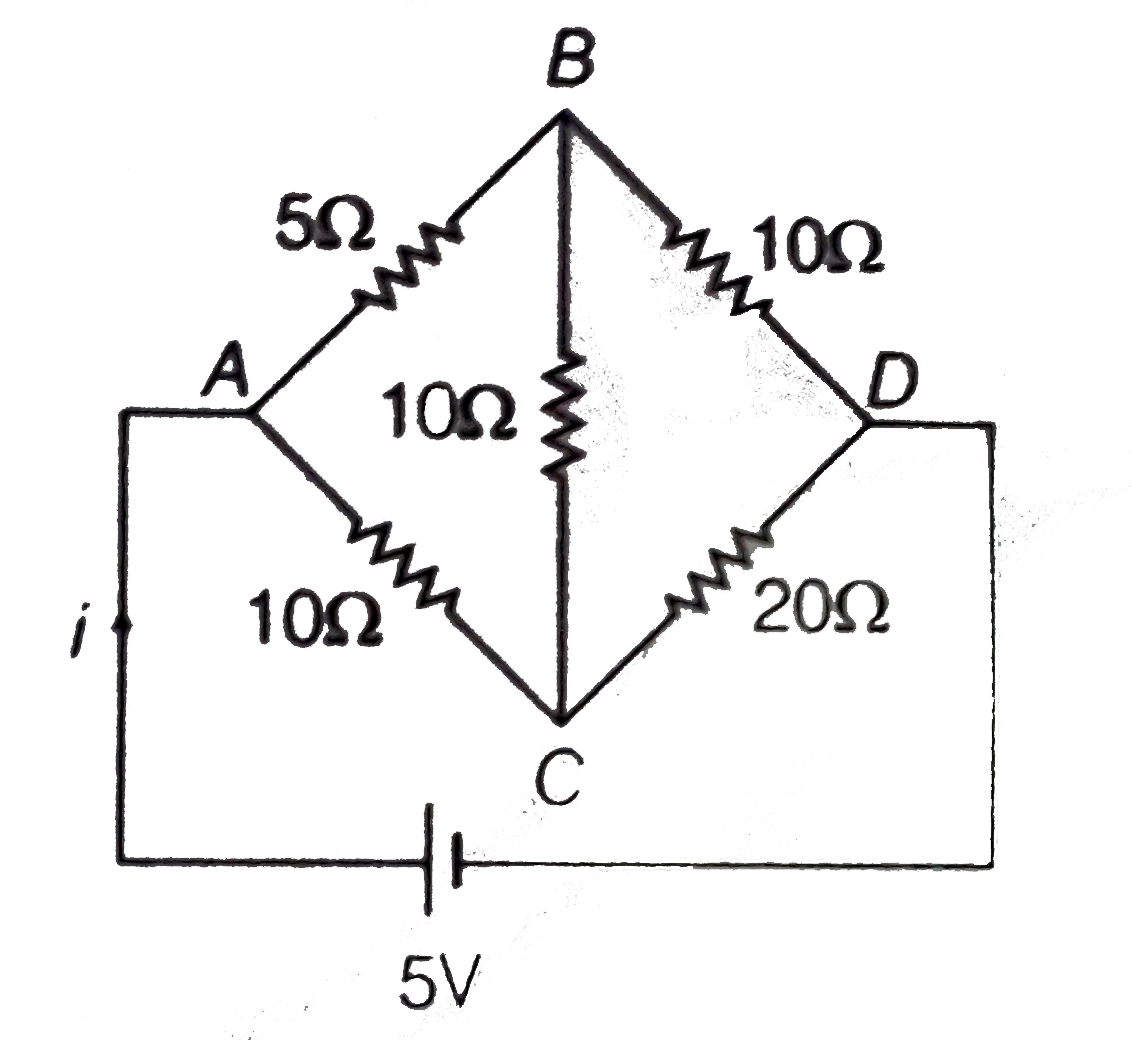 A0.5 AB0.2 AC0.041666666666667D0.083333333333333
A0.5 AB0.2 AC0.041666666666667D0.083333333333333
In the circuit shown in the figure,
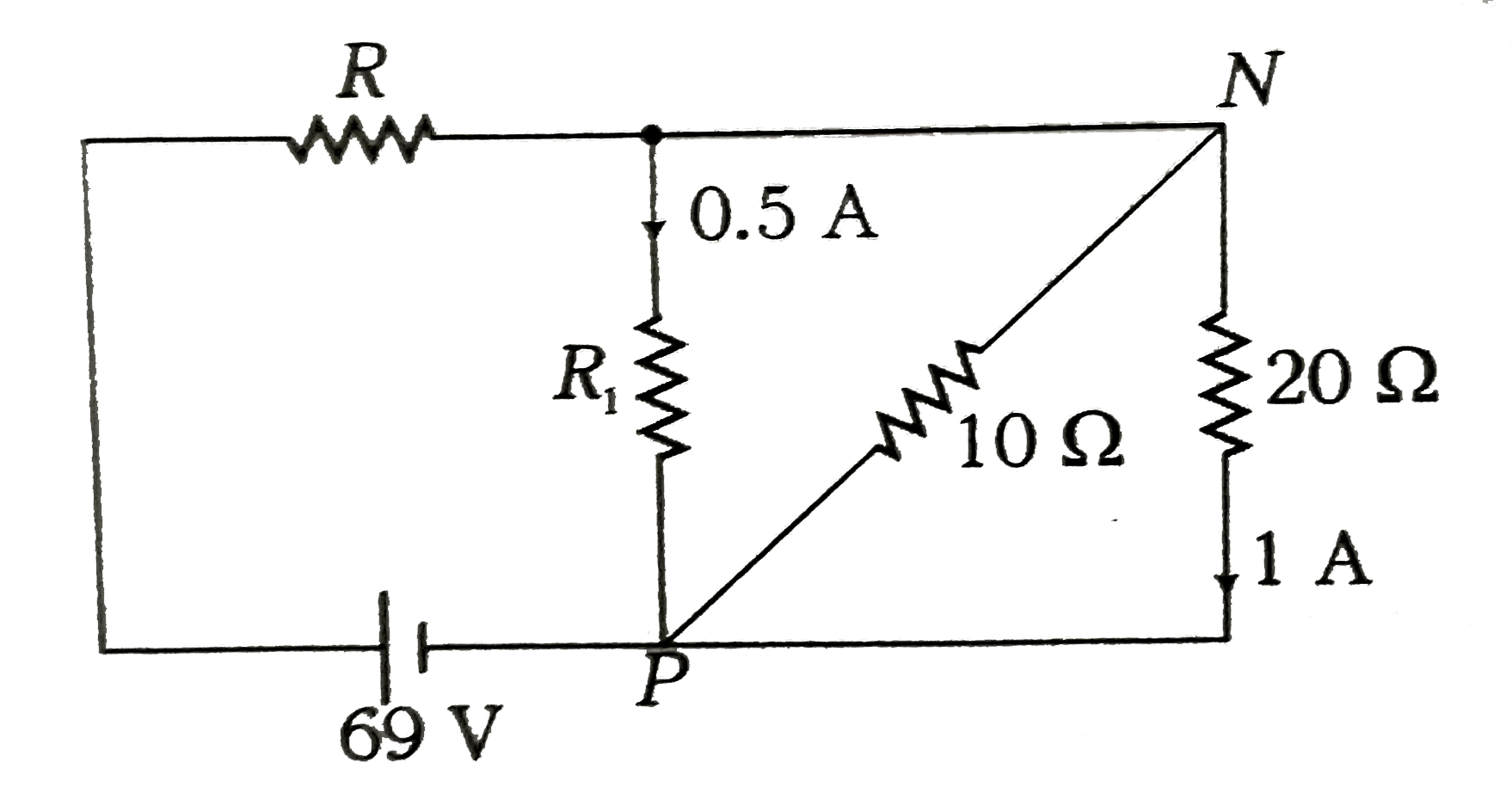
View SolutionR-L-C series AC circuit shown in fig. Find i_(max)
View SolutionIn the circuit shown in figure ,find the ratio of currents i1/i2.

View SolutionIn the circuit shown in figure ,find the ratio of currents i1/i2.

View SolutionFind the value of current I in the circuit
View Solution