A ΔPQR , given that QR=3cm, ∠PQR=45∘ and QP−PR=2cm.
Text Solution
Given, in ΔPQR, QR=3cm
∠PQR=45∘
and QP−PR = 2cm
To construct ΔPQR , use the following steps.
(i) Draw the base QR of length 3cm.
(ii) Make an angle XQR = 45∘ at point Q of base QR.
(iii) Cut the line segment QS = QP - PR = 2cm from the ray QX.
(iv) Join SR and draw the perpendicular bisector of SR say AB.
(v) Let bisector AB intersect QX at P. Join PR Thus, ΔPQR is the required triangle.
Justification
Base QR and ∠PQR are drawn as given. Since, the point P lies on the perpendicular bisector of SR.
∴ PS = PR
Now, QS = PQ-PS
= PQ - PR
Thus , our construction is justified.

|
Topper's Solved these Questions
Similar Questions
Knowledge Check
ΔPQR is right angled at point Q. If PQ=8cmand PR=(QR+2)cm. What is the value (in cm) of PR?
A17B15C19D18Tick the correct answer and justify : In ΔPQR, PQ = 6√3 cm , PR =12 cm and QR = 6 cm. The angle Q is :
A120∘B60∘C90∘D45∘
PQR is a triangle. S is a point on the side QR of ΔPQR such that ∠PSR=∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.

area of ΔPQRarea of ΔSPR
View SolutionPQR is a triangle. S is a point on the side QR of ΔPQR such that ∠PSR=∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.

Prove ΔPQR~ΔSPR.
View SolutionPQR is a triangle. S is a point on the side QR of ΔPQR such that ∠PSR=∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.

Find the lengths of QR and PS.
View Solution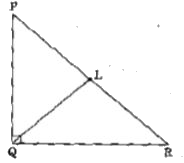
In the figure given above, ∠PQR=90∘ and QL is a median, PQ=5cmandQR=12cm, Then QL is equal to:
View SolutionConstruct a parallelogram PQRS, when PQ=3.7cm,QR=2.3cm,andPR=4.8cm.

View SolutionIn ΔPQR,∠R=∠PandQR=4cmandPR=5cm . Then find the value of length of PQ×QR×PR.
View Solution
