A thin non conducting disc of radius R is rotating clockwise (see figure) with an angular velocity ω about its central axis which is perpendicular to its plane Both its surfaces carry+ve charges of unifrom surface density Half the disc is in a region of a unifrom unidirectional magnetic field B parallel to the plane of the disc as shown Then
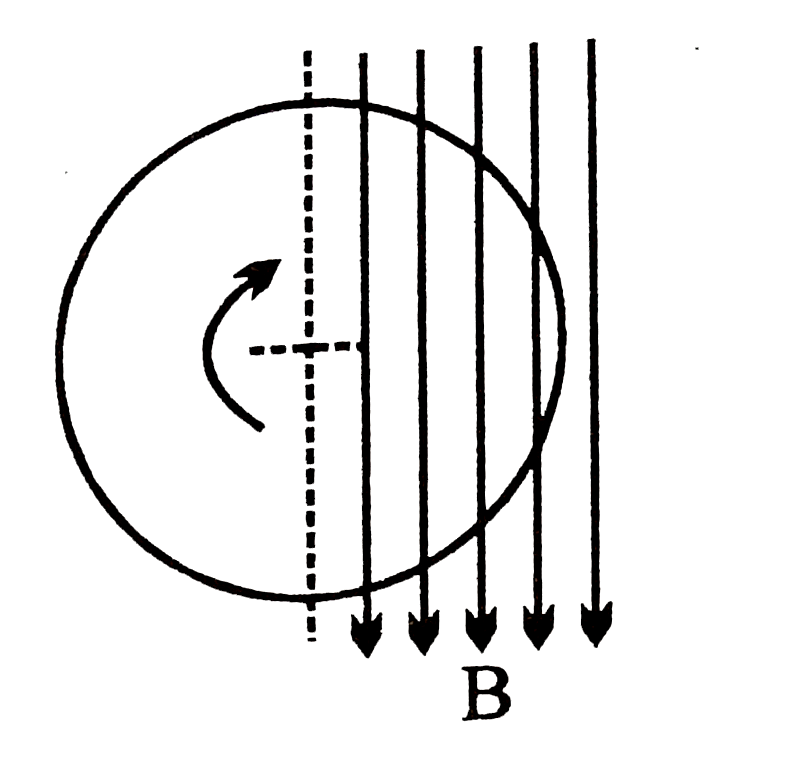 .
.
The net torque on the disc is zero
The net torque vector on the disc is directed leftwards
The net torque vector on the disc is directed
The net torque vector on the disc is parallel to B
The correct Answer is:B
Step by step video, text & image solution for A thin non conducting disc of radius R is rotating clockwise (see figure) with an angular velocity omega about its central axis which is perpendicular to its plane Both its surfaces carry+ve charges of unifrom surface density Half the disc is in a region of a unifrom unidirectional magnetic field B parallel to the plane of the disc as shown Then . by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MAGNETISM BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-2 (Level-I)
40 Videos
- MAGNETISM BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-2 (Level-II)
15 Videos
- MAGNETISM BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-4 (LEVEL-II)
40 Videos
- LOGIC GATESBOOK - MOTIONCHAPTER - LOGIC GATESEXERCISE - EXERCISE - 2
10 Videos
- MAGNETISM -1BOOK - MOTIONCHAPTER - MAGNETISM -1EXERCISE - Exercise - 3 SECTION -A
56 Videos
Similar Questions
A charge q is uniformly distributed on a non-conducting disc of radius R. It is rotated with an angular speed co about an axis passing through the centre of mass of the disc and perpendicular to its plane. Find the magnetic moment of the disc.
View SolutionA disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View Solution
Knowledge Check
A non conducting disc of radius R, charge q is rotating about an axis passing through its centre and perpendicular to its plane with an angular velocity ω charge q is uniformly distributed over its surface. The magnetic moment of the disc is
A14qωR2B12qωRCqωRD12qωR2A non-conducting disk of radius R is rotating about its own axis with constant angular velocity ω in a perpendicular uniform magnetic field B as shown in figure. The emf induced between centre and rim of disk is
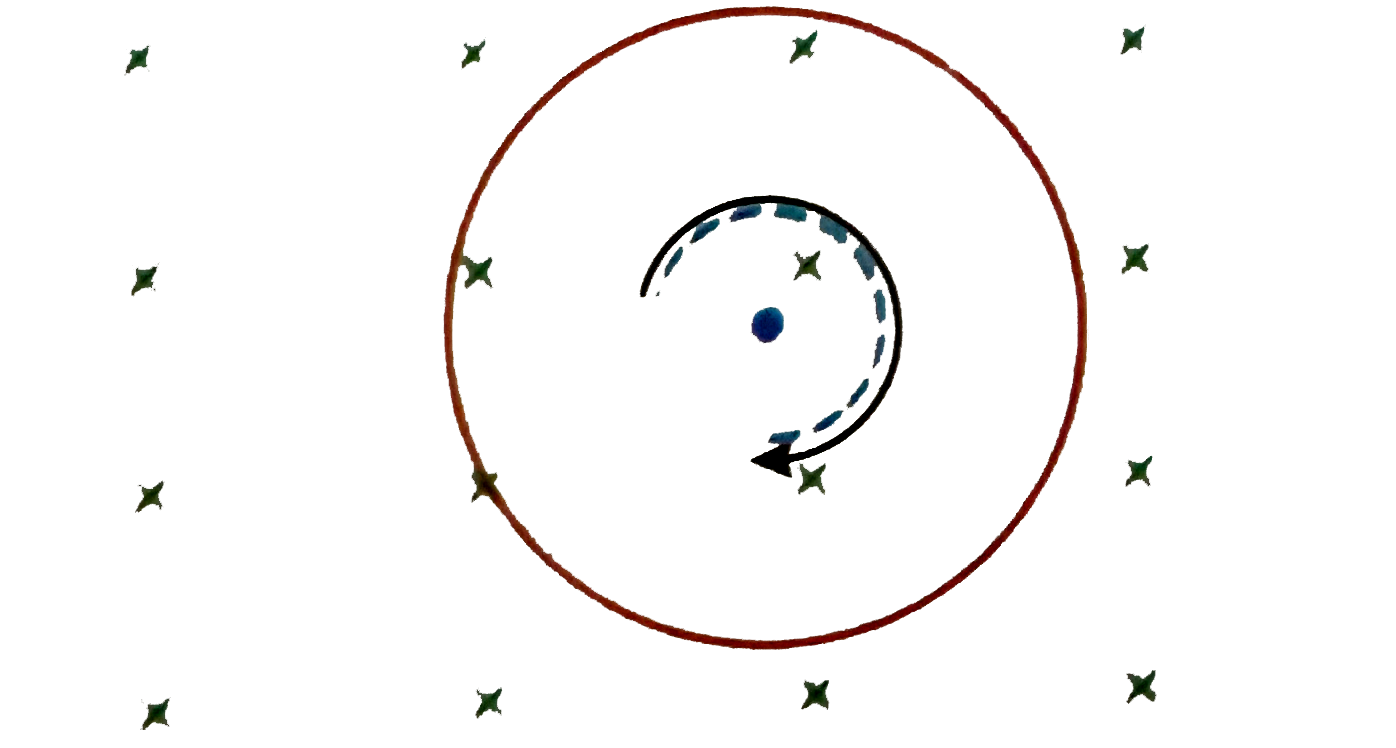 ABωR22BBωR2CZeroDBωR23
ABωR22BBωR2CZeroDBωR23A nonconducing disc of radius R is rotaing about axis passing through its cente and perpendicual its plance with an agular velocity ω . The magnt moment of this disc is .
A14qωR2B12qωRCqωRD12qωR2
A metal disc of radius R rotates with an angular velcoity ω about an axis perpendicular to its plane passing through its centre in a magnetic field B acting perpendicular to the plane of the disc. Calculate the induced emf between the rim and the axis of the disc.
View SolutionA thin metallic disc is rotating with constant angular velocity about a vertical axis that is perpendicular to its plane and passes through its centre. The rotation causes the free electrons in the disc to redistribute. Assume that there is no external electric or magnetic field. Then
View SolutionA heavy disc is rotating with uniform angular velocity ω about its own axis. A piece of wax sticks to it. The angular velocity of the disc will
View SolutionA non-conducting disc having unifrom positive charge Q, is rotating about its axis with unifrom angular velocity ω.The magnetic field at the centre of the disc is.

View Solutiona conducting disc of radius R roatates about its axis when an angular velocity ω.Then the potential difference between the centre of the disc and its edge is (no magnetic field is present ):
View Solution
