A proton beam passes without deviation through a region of space where there are uniform transverse mutually perpendicular electric and magnetic field with E and B Then the beam strikes a grounded target. Find the force imparted by the beam on the target if the beam current is equal to I .
mE1Be
Topper's Solved these Questions
- BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-3 (Level-II)23videos
MAGNETISM
- BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-4 (LEVEL-I)33videos
MAGNETISM
- BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-2 (Level-II)15videos
MAGNETISM
- BOOK - MOTIONCHAPTER - LOGIC GATESEXERCISE - EXERCISE - 210videos
LOGIC GATES
- BOOK - MOTIONCHAPTER - MAGNETISM -1EXERCISE - Exercise - 3 SECTION -A56videos
MAGNETISM -1
Similar Questions
A non-relativistic proton beam passes without deviation through a region of space where there are uniform transverse mutually perpendicular electric and magnetic fields with E=120kVm−1andB=50mT. Then the beam strikes a grounded target. Find the force which the beam acts on the target if the beam current is equal to i=0.8mA.
Mass of protons =1.67×10−27kg.View SolutionA non-relativistic protons beam passes without diviation through the region of space where there are unifrom transverse mutually perpendicular electric and magnetic fields with E=120kV/m and B=50mT. Then the beam strikes a grounded target. Find the force with which the beam acts on the target if the beam current is equal to I=0.80mA.
View Solution
Knowledge Check
- Question 1 - Select One
A beam of electrons passes undeflected through mutually perpendicular electric and magnetic fields. If the electric field is switched off, and the same magnetic field is maintained, the electrons move
Ain an elliptical orbitBin a circular orbitCalong a parabolic pathDalong a straight line - Question 2 - Select One
A beam of electrons passes undeflected through mutually perpendicular electric and magnetic fields. If the electric field is switched off, and the same magnetic field is maintained, the electrons move
Ain an elliptical orbitBin a circular orbitCalong a parabolic pathDalong a straight line - Question 3 - Select One
A beam of electrons passes underfected throgh unifromly perpendicular electric and magnetic fields. If the electric fiedl is swiched off, and the same magnetic field is mainted fiedl is maintetained the electrons move:
Ain a eliptical orbitBin a circular orbitCalong a parabolic pathDalong a straight line
A beam of non-relativistic charged particles moves without deviation through the region of space A where there are transverse mutually perpendicular electric and magnetic fields with strength E and induction B. When the magnetic field is switched off, the trace of the beam on the screen S shifts by Δx. Knowing the distance a and b, find the specific charge q/m of the particles.
View SolutionA beam of non-relatitivistic chagred particles moves without deviation through the region of space A (fig) where there are transerve mutually perpendicular electric and magnetic fields with streght E and induction B. When the magnetic field is swichted off, the trace of the beam on the screen S shifts by Δπ. Knowing the distances a and b, find the spefic charge q/m of the particles.
 View Solution
View SolutionProtons move rectilinearly in the region of space where there are uniform mutually perpendicular electric and magnetic fields E and B. The trajectory of protons lies in the plane xz as shown in the figure and forms an angle 9 with x-axis. Find the pitch of the helical trajectory along which the protons will move after the electric field is switched off.
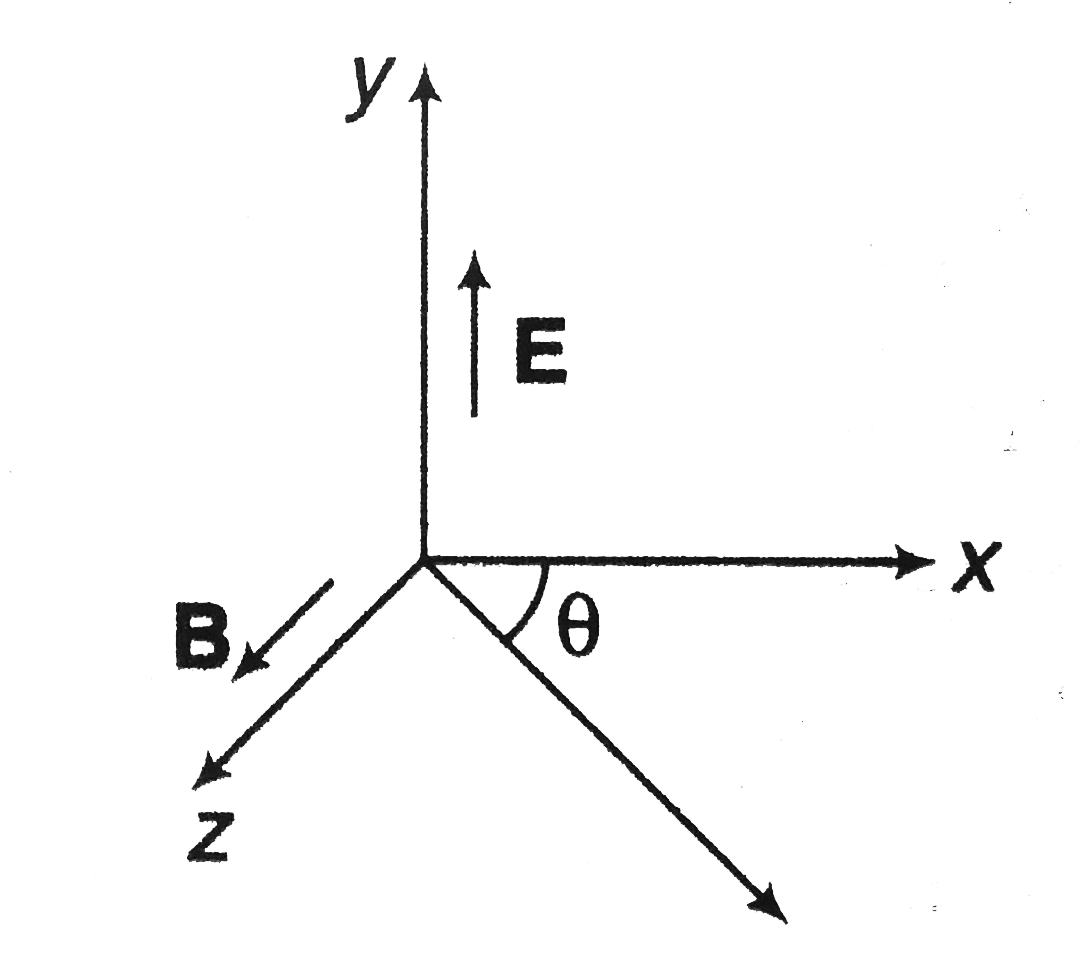 View Solution
View SolutionA narrow beam of identical ions with specific charge q/m, possessing different velocities, enters the region of space, where there are uniform parallel electric and magnetic fields with strengths EandB, at the point O. The beam direction coincides with the x-axis at the point O. A plane screen oriented at right angles to the x-axis is located at a distance I from O. Find the equation of trace that the ion leaves on the screen. Demonostrate that at z<<l, it is the equation of a parabola.
View SolutionAn electron enters a region where magnetic field (B) and electric field (E ) are mutually perpendicular, then
View Solution

