A particle of massM and positive charge Q, moving with a constant velocity →u1=4ˆims−1, enters a region of uniform static magnetic field , normal to the x−y plane. The region of the magnetic field extends from x=0 to x=L for all values of y. After passing through this region, the particle emerges on the other side after 10milliseconds with a velocity →u2=2(√3ˆi+ˆj)ms−1 . The correct statement(s) is (are)
the direction of the magnetic field is –z direction.
the direction of the magnetic field is +z direction.
the magnitude of the magnetic field is 50πM3Q units.
the magnitude of the magnetic field is 100πM3Q units.
The correct Answer is:A, C
Step by step video, text & image solution for A particle of massM and positive charge Q, moving with a constant velocity vec(u_(1)) = 4 hat(i) ms^(-1), enters a region of uniform static magnetic field , normal to the x-y plane. The region of the magnetic field extends from x= 0 to x = L for all values of y. After passing through this region, the particle emerges on the other side after 10 milliseconds with a velocity vec(u_(2)) = 2 (sqrt(3 hat(i) + hat(j))) ms^(-1) . The correct statement(s) is (are) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
A charged particle of mass m and charge q travels on a circular path of radius r that is perpendicular to a magnetic field B. The time takeen by the particle to complete one revolution is
12:24View SolutionOut of the following, universal gate is :-
09:23View SolutionAn electron moves in a circular orbit with a uniform speed v.It produces a magnetic field B at the centre of the circle. The radius of the circle is proportional to
05:41View SolutionA particle of massM and positive charge Q, moving with a constant velocity →u1=4ˆims−1, enters a region of uniform static magnetic field , normal to the x−y plane. The region of the magnetic field extends from x=0 to x=L for all values of y. After passing through this region, the particle emerges on the other side after 10milliseconds with a velocity →u2=2(√3ˆi+ˆj))ms−1 . The correct statement(s) is (are)
09:05View SolutionThe region between x=o and x = L is filled with uniform, steady magnetic field B_(0) hat(k) . A particle of mass m , positive charge q and velocity v_(0) hat(i) travels along x-axis and enters the region of the magnetic field. Neglect gravity throughout the question . (a) Find the value of L if the particle emerges from the region of magnetic field with its final velocity at angle 30^(@) to its initial velocity. (b) Find the velocity of the particle and the time spent by it in the magnetic field, if the magnetic field now extends upto 2.1L .
08:45View SolutionThe region between x=0 and x=L is filled with uniform steady magnetic field B0ˆk. A particle of mass m, positive charge q and velocity v0ˆi travels along x-axis and enters the region of the magnetic field. Neglect the gravity throughout the question.
a. Find the value of l if the particle emerges from the region of magnetic field with its final velocilty at an angel 30∘ to its initial velocity.
b. Find the final velocity of the particle and the time spent by it in the magnetic field, if themagnetic field now extends upto 2.1L.07:55View SolutionThe region between x=0 and x=L is filled with uniform constant magnetic field 25(T) hatk . A particle of mass m=50g having positive charge q=1C and velocity vecV_0=50sqrt3hati+50hatjms^-1 enters the region of the magnetic field. Neglect gravity throughout the question. The value of L if the particle emerges from the region of magnetic field with its velocity 100(ms^-1)hati is
03:46View SolutionIn region x gt 0 a uniform and constant magnetic field vec(B)_(1) = 2 B_(0) hat(k) exists. Another uniform and constant magnetic field vec(B)_(2) = B_(0) hat(k) exists in region x lt 0 A positively charged particle of mass m and charge q is crossing origin at time t =0 with a velocity bar(u) = u_(0) hat(i) . The particle comes back to its initial position after a time : ( B_(0),u_(0) are positive constants)
03:46View SolutionA particle of mass m and charge q enters a region of magnetic field (as shown) with speed v at t = 0. There is a region in which the magnetic field is absent as shown. The particle after entering the region collide elastically with a rigid wall. Time t after which the velocity of particle become antiparallel to its initial velocity is -
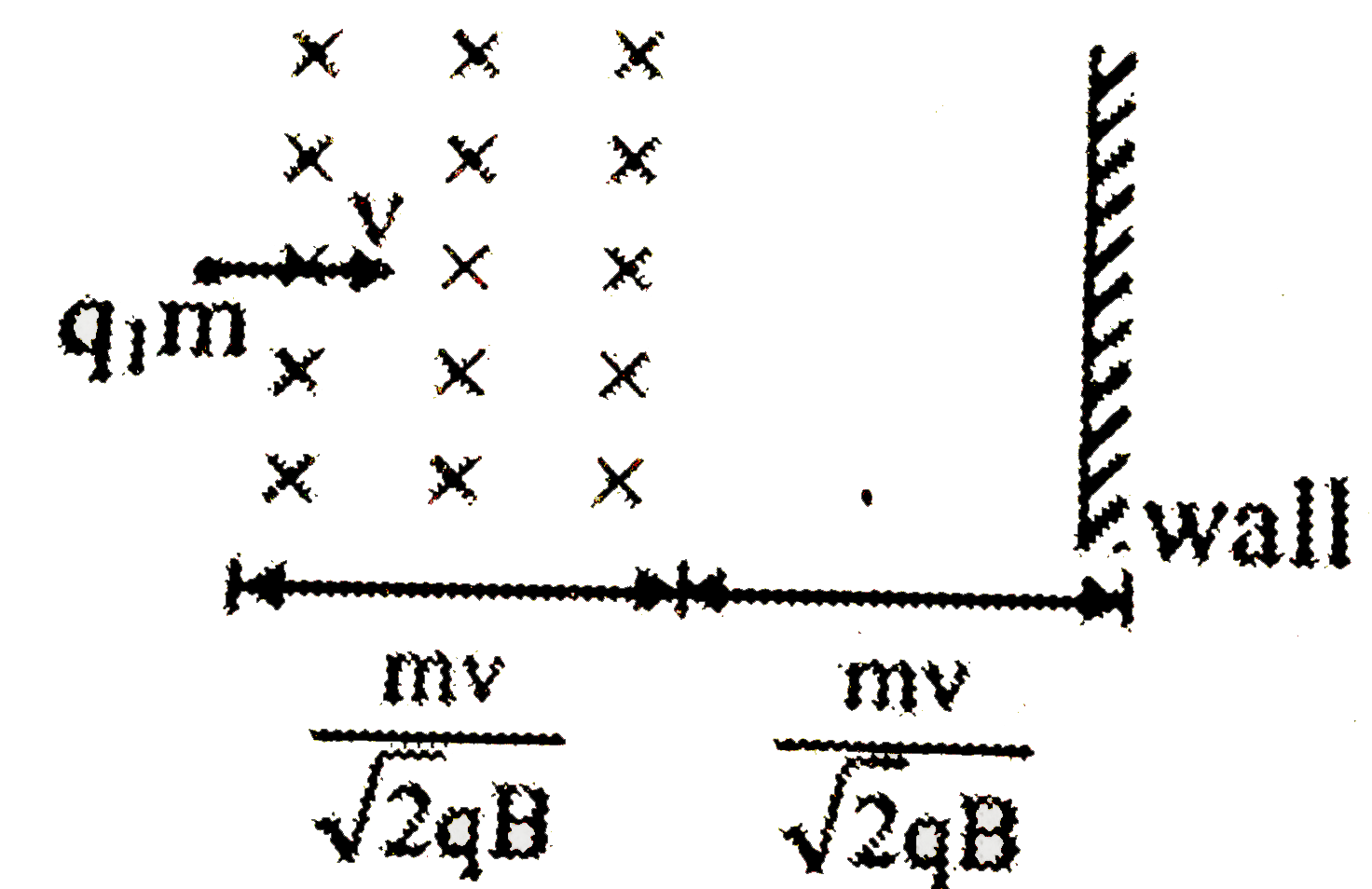 View Solution
View SolutionA charge particle enters into a region containing uniform electric field (E) and uniform magnetic field (B) along x- axis and y-axis respectively.If it passes the region undeviated the velocity of charge particle is given by
09:25View SolutionA charge particle enters into a region containing uniform electric field (E) and uniform magnetic field (B) along x-axis and y-axis respectively.If it passes the region undeviated the velocity of charge particle is given by
07:24View Solutionएक M द्रव्यमान तथा O धनावेश का कण जो →u1=4ˆims−1 के एकसमान वेग से गतिशील से है , एक समान स्थिर चुम्बकीय क्षेत्र मे x-y तल के अभिलंबवत है तथा इसका विस्तार क्षेत्र x = 0 से x= L तक प्रत्येक y के मान के लिए है। इस चुम्बकीय क्षेत्र का यह कण 10 मिली सेकंड मे पार कर दूसरी ओर →u2=2(√3ˆi+ˆj)ms−1 वेग से प्रकट होता है। सही प्रकथन है /है -
View SolutionOne M mass and Q particle of positive charge, which vecu_1=4hati ms^(-1) K is moving with uniform velocity, enters a uniform constant magnetic field. This magnetic field is perpendicular to the xy plane and its expansion field is from x = 0 to x = L for every y value. This particle is wired to this magnetic field in 10 milli-seconds and on the other side vecu_2=2(sqrt3hati+hatj) ms^(-1) Appears with velocity. Correct statement है//हैं
06:30View SolutionThe region between x=0 and x=L is filled with uniform steady magnetic field B0ˆk. A particle of mass m, positive charge q and velocity v0ˆi travels along x-axis and enters the region of the magnetic field. Neglect the gravity throughout the question.
a. Find the value of l if the particle emerges from the region of magnetic field with its final velocilty at an angel 30∘ to its initial velocity.
b. Find the final velocity of the particle and the time spent by it in the magnetic field, if themagnetic field now extends upto 2.1L.04:33View Solutionमाना एक समरूप तथा नियत चुंबकीय क्षेत्र Bˆk, स्थान y>0 में उपस्थित है | एक आवेशित कण जिसका q तथा द्रव्यमान m है, y-अक्ष के अनुदिश चलता हुआ v0 चाल से, मूल बिंदु पर चुंबकीय क्षेत्र में प्रवेश करता है | इस क्षेत्र में कण पर, एक अतिरिक्त घर्षण बल →F=−kˆv भी उपस्थित है | यह मानिये कि कण पूर्ण में y>0 क्षेत्र में ही उपस्थित है तो उस बिंदु के निर्देशांक, जिस पर कण अन्त में रुकेगा, (x, y) है तो
03:07View SolutionThe region between x=0 and x=L is filled with uniform constant magnetic field 25(T) hatk . A particle of mass m=50g having positive charge q=1C and velocity vecV_0=50sqrt3hati+50hatjms^-1 enters the region of the magnetic field. Neglect gravity throughout the question. The value of L if the particle emerges from the region of magnetic field with its velocity 100(ms^-1)hati is
05:59View Solution

