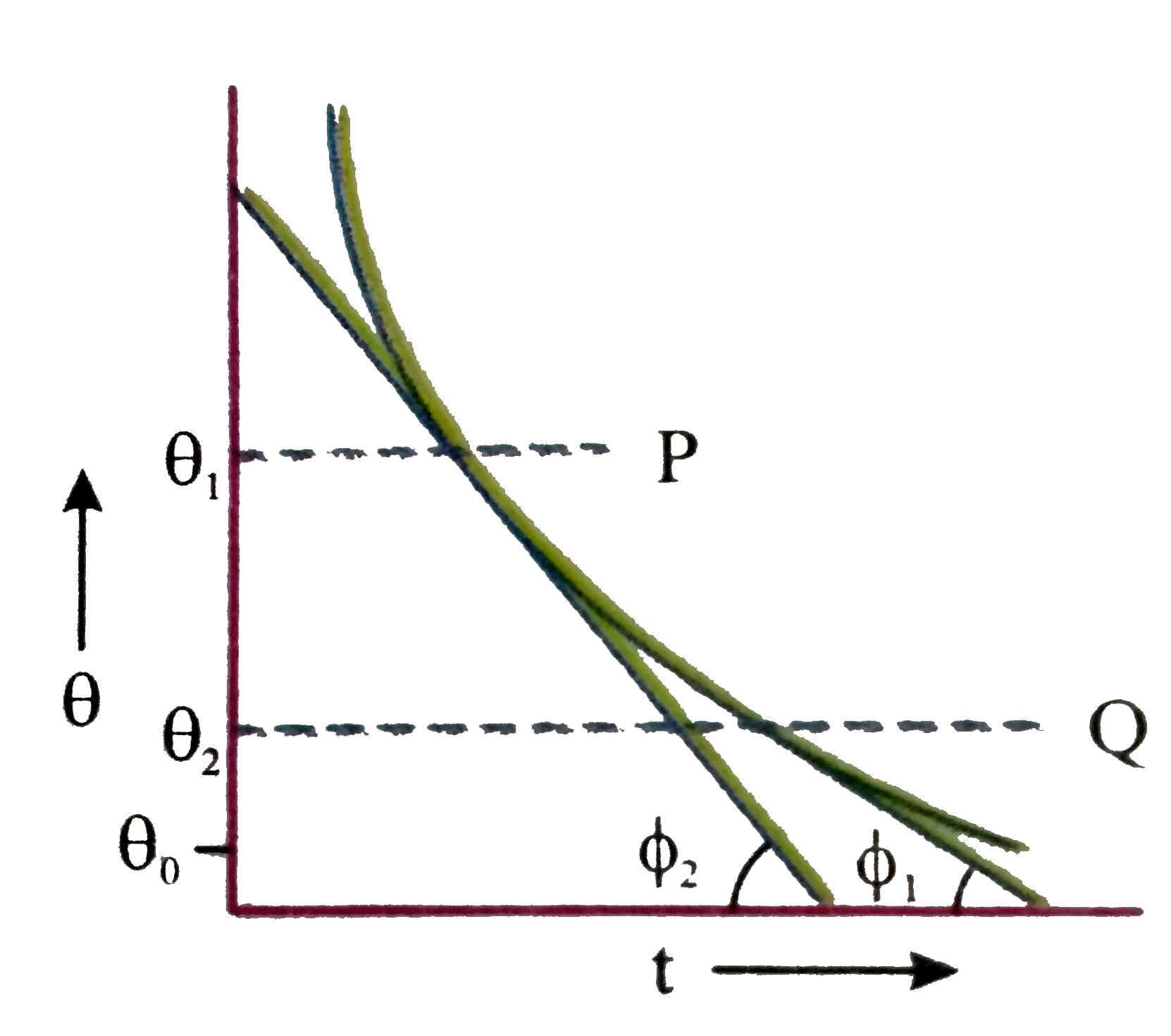
A body cools in a surrounding which is at a constant temperature of $θ_{0}$ Assume that it obeys Newton's law of cooling Its temperature $θ$ is plotted against time t Tangents are drawn to the curve at the points $P (θ = θ_{1})$ and $Q (θ = θ_{2})$ These tangents meet the time axis at angle of $ϕ_{2}$ and $ϕ_{1}$ as shown
.

Video Solution

Text Solution

Verified by Experts

Temperature as a function of time is given as
$T_{1} - T_{0}) e^{- k t}$
Slope of curve is
$\frac{d T}{d t} = - k (T_{1} - T_{0}) e^{- k t} = - k (T - T_{0}$
if at $T = T_{1}$ slope is ${tan θ}_{1}$ , we use
${tan θ}_{1} = - k (T_{1} - T_{0})$ …(1)
and at $T = T_{2}$ slope is ${tan θ}_{2}$ , we use
${tan θ}_{2} = - k (T_{2} - T_{0})$ ...(2)
$\frac{(2)}{(1)}$ gives
$\frac{{tan θ}_{2}}{{tan θ}_{1}} = \frac{T_{2} - T_{0}}{T_{1} - T_{0}}$

Updated on:21/07/2023

Class 12 PHYSICS HEAT TRANSFER

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
A body cools in a surrounding which is at constant temperature of $θ_{0}$ . Assume that it obeys Newyon's law of colling. Its temperature $θ$ is plotted against time $t$ . Tangents are drawn to the curve at the points $P (θ = θ_{t})$ and $Q (θ = θ_{2})$ . These tangents meet the time axis at angles of $ϕ_{2}$ and $ϕ_{1}$ , as shown

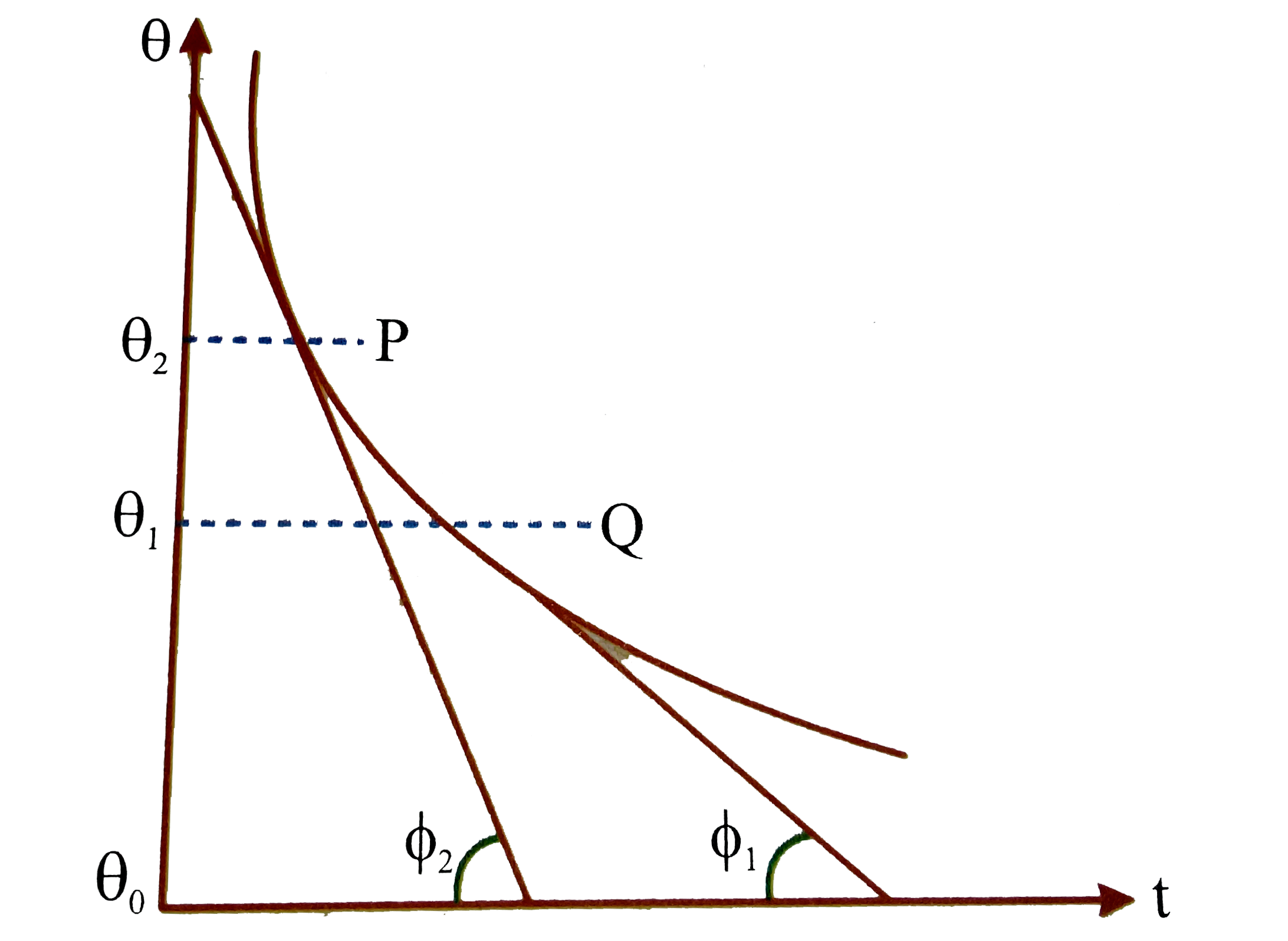A $\frac{{tan ϕ}_{2}}{{tan ϕ}_{1}} = \frac{θ_{1} - θ_{0}}{θ_{2} - θ_{0}}$
B $\frac{{tan ϕ}_{2}}{{tan ϕ}_{1}} = \frac{θ_{2} - θ_{0}}{θ_{1} - θ_{0}}$
C $\frac{{tan ϕ}_{1}}{{tan ϕ}_{2}} = \frac{θ_{1}}{θ_{2}}$
D $\frac{{tan ϕ}_{1}}{{tan ϕ}_{2}} = \frac{θ_{2}}{θ_{1}}$
Question 2 - Select One
A body obeying Newton's law of cooling cools in a surrounding which is at a constant temperature Its temperature $θ$ is plotted against time There are two points on the curve with temperatures $θ_{2}$ and $θ_{1} (θ_{2} > θ_{1})$ such that tangents on these points make angles of $2 ϕ$ and half of its with time axis respectively Find the temperature of the surrounding .
A $θ_{1} cos 2 ϕ - θ_{2} (1 + cos 2 ϕ)$
B $θ_{1} (1 + cos 2 ϕ) - θ_{2} cos 2 ϕ$
C $(θ_{2} - θ_{1}) cos 2 ϕ$
D $\frac{2 θ_{1} + θ_{2} (1 + {tan}^{2} ϕ)}{(1 - {tan}^{2} ϕ)}$
Question 3 - Select One
A body with an initial temperature $θ_{1}$ is allowed to cool in a surrounding which is at a constant temperature of $θ_{0} (θ < θ_{1})$ Assume that Newton's law of cooling is obeyed Let $k =$ constant The temperature of the body after time t is best experssed by .
A $(θ_{i} - θ_{0}) e^{- k t}$
B $(θ_{i} - θ_{0}) I n (k t)$
C $θ_{0} + (θ_{i} - θ_{0}) e^{- k t}$
D $θ_{i} e^{- k t} - θ_{0}$

PHYSICS GALAXY - ASHISH ARORA-HEAT TRANSFER -Practice Exercise 4.3

Home
Ask Doubt
Profile

A body cools in a surrounding which is at a constant temperature of θ0 Assume that it obeys Newton's law of cooling Its temperature θ is plotted against time t Tangents are drawn to the curve at the points P(θ=θ1) and Q(θ=θ2) These tangents meet the time axis at angle of ϕ2 andϕ1 as shown .

Temperature as a function of time is given as T1−T0)e−kt Slope of curve is dTdt=−k(T1−T0)e−kt=−k(T−T0 if at T=T1 slope is tanθ1, we use tanθ1=−k(T1−T0) …(1) and at T=T2 slope is tanθ2, we use tanθ2=−k(T2−T0) ...(2) (2)(1) gives tanθ2tanθ1=T2−T0T1−T0

Topper's Solved these Questions

Similar Questions

Knowledge Check

A body with an initial temperature θ1 is allowed to cool in a surrounding which is at a constant temperature of θ0(θ<θ1) Assume that Newton's law of cooling is obeyed Let k= constant The temperature of the body after time t is best experssed by .

PHYSICS GALAXY - ASHISH ARORA-HEAT TRANSFER -Practice Exercise 4.3

A body with an initial temperature $θ_{1}$ is allowed to cool in a surrounding which is at a constant temperature of $θ_{0} (θ < θ_{1})$ Assume that Newton's law of cooling is obeyed Let $k =$ constant The temperature of the body after time t is best experssed by .