If a loop of chain is spunat high speed, it will roll like a hoop without collapsing. Consider a chain oflinear mass density p that μ is rollingwithoutslipping at a high speed v0
(a) Show that the tension in the chain is F=μv20
The correct Answer is:B
Topper's Solved these Questions
- WAVES BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WAVES EXERCISE - Advance MCQs with One or More Options Correct
30 Videos
- WAVE OPTICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WAVE OPTICSEXERCISE - Unsolved Numerical Problems
28 Videos
- X-RAYSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - X-RAYSEXERCISE - Unsolved Numerica Problem for Preparation
23 Videos
Similar Questions
If a loop of chain is spunat high speed, it will roll like a hoop without collapsing. Consider a chain oflinear mass density p that μ is rollingwithoutslipping at a high speed v0
(c)How far around the loop (in degrees) will a transverse pulse travelin the time the hoop rolls through one complete revolution ?
View SolutionIf a loop of chain is spunat high speed, it will roll like a hoop without collapsing. Consider a chain oflinear mass density p that μ is rollingwithoutslipping at a high speed v0
(b) If the chain rolls over a small hump, a transverse wave pulse will be generated in the chain. At whatspeedwill it travel alongthe chain ?
View Solution
Knowledge Check
A 10 m long iron chain of linear mass density 0.8 kg m−1 is hanging freely from a rigid support .If g=10 ms−2 ,then the power required to left the chain upto the point of support in 10 second
A10 WB20WC30WD40WA uniform chain has a mass M and length L. It is placed on a frictionless table with length l0 hanging over the edge. The chain begins to slide down. Ten, the speed v with which the end slides down from the edge is given by
Av=√gL(L+l0)Bv=√gL(L−l0)Cv=√gL(L2−l20)Dv=√2g(L-l_(0)))A solid sphere at the top of an inclined plane 0.6 m high is released and rolls down the incline without slipping and without loss of energy due to friction. Its linear speed at the bottom is about
A2.9m/sB2.42m/sC3.87m/sD1.53m/s
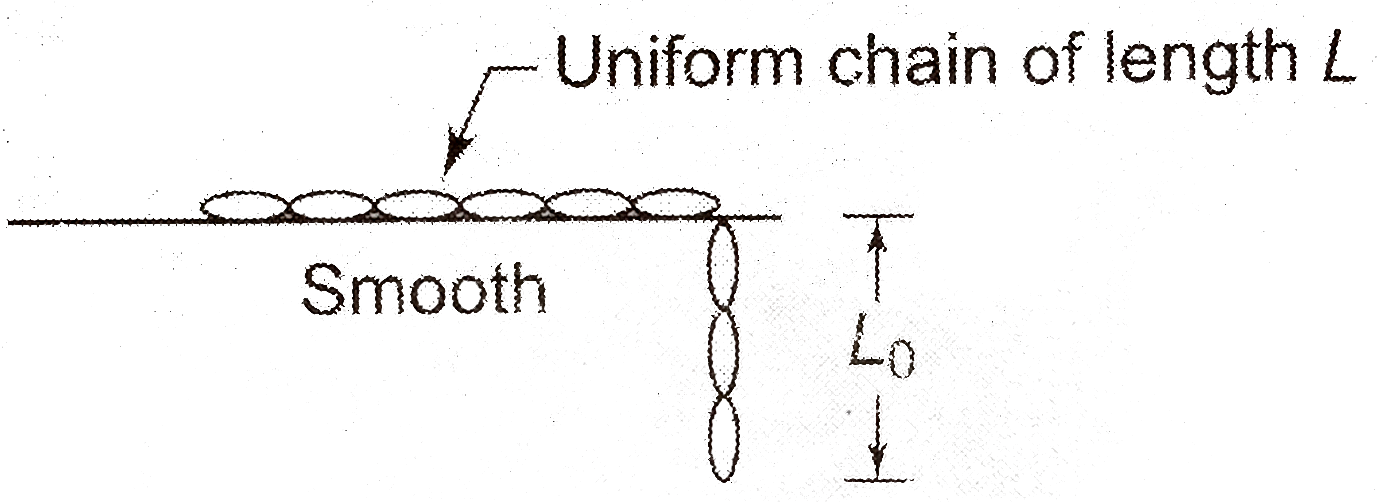
Consider a uniform chain of mass m and length L, the chain is released when hanging length is L0. Find the speed with which the chain leaves the table.
View Solution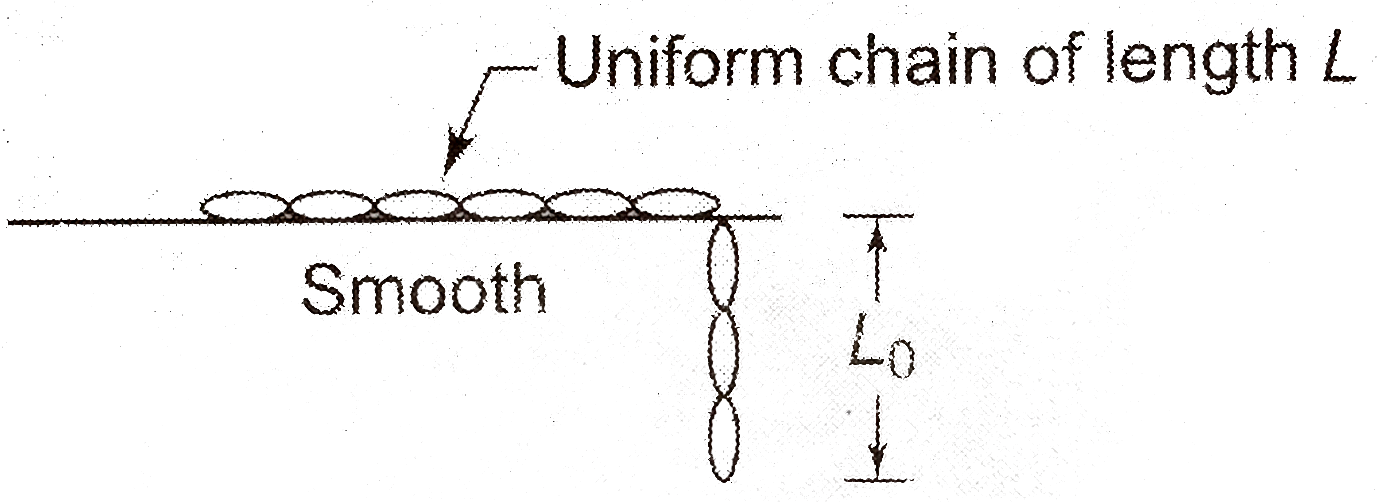
Consider a uniform chain of mass m and length L, the chain is released when hanging length is L0. Find the speed with which the chain leaves the table.
View SolutionA chain of mass m and radius R placed on a smooth table is revolving with a speed v about a vertical axis coinciding with the symmetry axis of the chain. Find the tension in the chain.
View SolutionA chain (A) of length L is coiled up on the edge of a table. Another identical chain (B) is placed straight on the table as shown. A very small length of both the chains is pushed off the edge and it starts falling under gravity. There is no friction
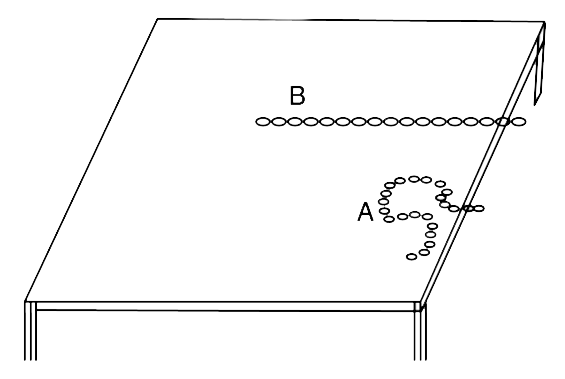
(a) Find the acceleration of the chain B at the instant L2 length of it is hanging. Assume no kinks in the chain so that the entire chain moves with same speed.
(b) For chain A assume that velocity of each element remains zero until it is jerked into motion with a velocity equal to that of the falling section. Find acceleration of the hanging section at the instant a length l0 has slipped off the table and its speed is known to be v0 at the instant.
View SolutionA uniform chain has a mass M and length L. It is placed on a frictionless table with length l0 hanging over the edge. The chain begins to slide down. Ten, the speed v with which the end slides down from the edge is given by
View Solution
