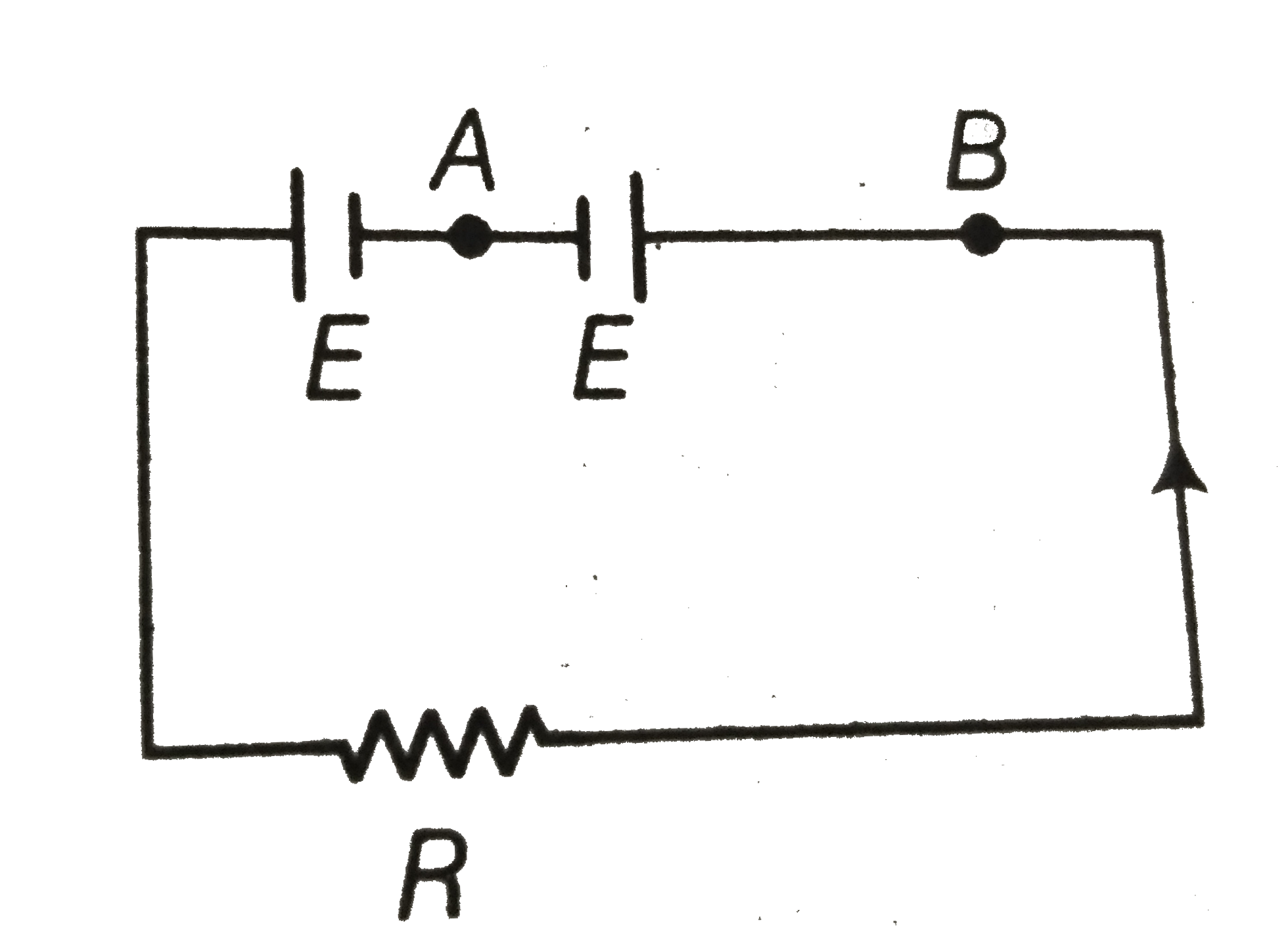
Two cells of same emf E but internal resistance r1andr2 are connected in series to an external resistor R(figure). What should be the value of R so that the potential difference across the terminals of the first cell becomes zero?
Applying Ohm's law
Effective resistance =R+r1+r2 and effective emf of two cells =E+E=2E, so the electric current is given by
I=E+EE+r1+r2
The potential difference across the terminals of the first cell and putting it equal to zero.
V1=E−Ir1=E−2Er1+r2+Rr1=0
or E=2Er1r1+r2+R⇒1=2r1r1+r2+R
r1+r2+R=2r1⇒R=r1−r2
this is the required relation.
Topper's Solved these Questions
Similar Questions
Two cells of same emf ε but internal resistances r1 and r2 are connected in series to an external resistor R. What should be the value of R so that the potential difference across the terminals of the first cell becomes zero.

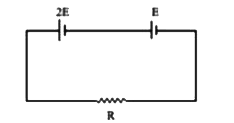
View SolutionTwo cells of emf 2E and E with internal resistance r1 and r2 respectively are connected in series to an external resistor R (see figure). The value of R, at which the potential difference across the terminals of the first cell becomes zero is
View Solution
Knowledge Check
Tow cells with the same emf E and different internal resistances r1 and r2 are connected in series to an external resistances R . The value of R so that the potential difference across the first cell be zero is
A√r1r2Br1+r2Cr1−r2Dr1+r22Two cell with the same e.m.f. E and difference internal resistance r1andr2 are connected in series to an external resistance R a value of R be selected such that the potential difference as the flow cell E should be zero when
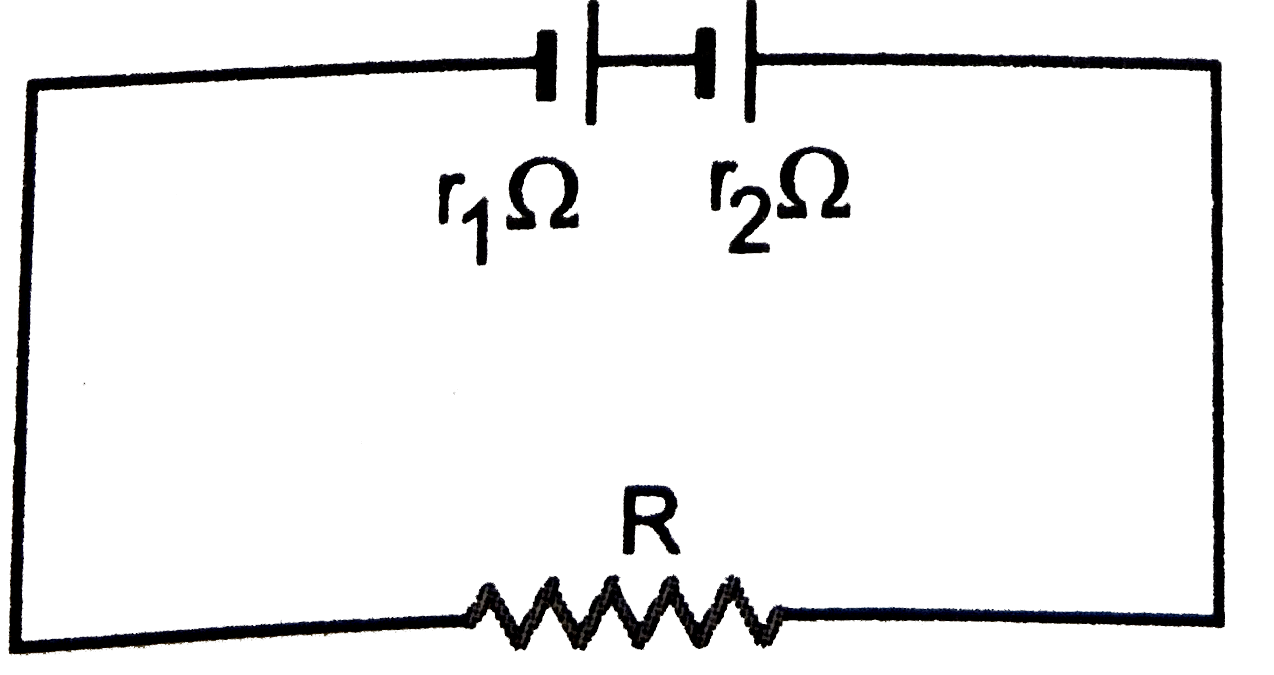 AR=r1+r2BR=r1−r2CR=r1/r2DR=r1=r2
AR=r1+r2BR=r1−r2CR=r1/r2DR=r1=r2Two cells of the same e.mf. e but different internal resistances r1andr2 are connected in series with an external resistance 'R'. The potential drop across the first cell is found to be zero. The external resistance Ris
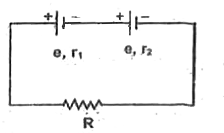 Ar1−r2Br1r2Cr1r2Dr1+r2
Ar1−r2Br1r2Cr1r2Dr1+r2
Two cells of the same e.mf. e but different internal resistances r1andr2 are connected in series with an external resistance 'R'. The potential drop across the first cell is found to be zero. The external resistance Ris
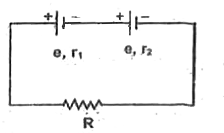
View SolutionA circuit consists of two cells, each of e.m.f. E and internal resistances r1 and r2 are connected in series through an external resistance X. If the potential difference between the ends of the first cell is zero, the value of X in terms of r1 and r2 will be
View SolutionA cell of emf ε and internal resistance (r) is connected in series with an external resistance (nr) then the ratio of the terminal potential difference to emf is:
View SolutionA cell of emf E and internal resistance r is connected in series with an external resistance nr. Than what will be the ratio of the terminal potential difference to emf, if n=9.
View SolutionTwo cells each of emf E and internal resistacne r1 and r2 respectively are conneted in series with an external resistance R. The potential defference between the terminats of the first cell will be zero when R equal to
View Solution
