Replace A,B and C by suitable numerals.
The correct Answer is:A=6, B=6, C=5
To solve the equation CB5−28A 259, we need to replace A, B, and C with suitable numerals.
Let's break it down step by step:
Step 1: Understand the structure of the equation
The equation can be interpreted as:
\(
\frac{CB5 - 28A}{259}
\)
Here, CB5 represents a three-digit number where C is the hundreds place, B is the tens place, and 5 is the units place. 28A represents a three-digit number where 2 is the hundreds place, 8 is the tens place, and A is the units place.
Step 2: Rewrite the equation
We can express CB5 and 28A in terms of their digits:
- CB5 = \(100C + 10B + 5\)
- 28A = \(280 + A\)
Thus, the equation becomes:
\(
\frac{(100C + 10B + 5) - (280 + A)}{259}
\)
Step 3: Simplify the equation
Now, let's simplify the numerator:
\(
100C + 10B + 5 - 280 - A = 100C + 10B - A - 275
\)
So, we have:
\(
\frac{100C + 10B - A - 275}{259}
\)
Step 4: Set up the equation
For the division to be valid, the numerator must be a multiple of 259. Let's denote:
\(
100C + 10B - A - 275 = k \cdot 259
\)
for some integer k.
Step 5: Find suitable values for A, B, and C
To find suitable values for A, B, and C, we can start testing values for k. We know that A, B, and C are digits (0-9).
1. Testing k = 1:
\(
100C + 10B - A - 275 = 259
\)
Rearranging gives:
\(
100C + 10B - A = 534
\)
2. Testing k = 2:
\(
100C + 10B - A - 275 = 518
\)
Rearranging gives:
\(
100C + 10B - A = 793
\)
This is not possible since \(100C + 10B\) cannot exceed 999.
3. Testing k = 0:
\(
100C + 10B - A - 275 = 0
\)
Rearranging gives:
\(
100C + 10B - A = 275
\)
Step 6: Solve for A, B, and C
Now we can solve for A, B, and C:
- Since \(100C + 10B = 275 + A\), we can try different values for A (0-9) and see if we can find suitable values for B and C.
1. If A = 5:
\(
100C + 10B = 275 + 5 = 280
\)
- \(C = 2\) and \(B = 8\) gives us \(CB5 = 285\).
2. If A = 6:
\(
100C + 10B = 275 + 6 = 281
\)
- This does not yield valid digits for B and C.
3. If A = 4:
\(
100C + 10B = 275 + 4 = 279
\)
- \(C = 2\) and \(B = 7\) gives us \(CB5 = 275\).
Conclusion
After testing various values, we find:
- \(C = 2\)
- \(B = 7\)
- \(A = 5\)
Thus, the suitable numerals are:
- A = 6
- B = 4
- C = 2
The equation can be interpreted as:
\(
\frac{CB5 - 28A}{259}
\)
Here, CB5 represents a three-digit number where C is the hundreds place, B is the tens place, and 5 is the units place. 28A represents a three-digit number where 2 is the hundreds place, 8 is the tens place, and A is the units place.
We can express CB5 and 28A in terms of their digits:
- CB5 = \(100C + 10B + 5\)
- 28A = \(280 + A\)
\(
\frac{(100C + 10B + 5) - (280 + A)}{259}
\)
Now, let's simplify the numerator:
\(
100C + 10B + 5 - 280 - A = 100C + 10B - A - 275
\)
So, we have:
\(
\frac{100C + 10B - A - 275}{259}
\)
For the division to be valid, the numerator must be a multiple of 259. Let's denote:
\(
100C + 10B - A - 275 = k \cdot 259
\)
for some integer k.
To find suitable values for A, B, and C, we can start testing values for k. We know that A, B, and C are digits (0-9).
\(
100C + 10B - A - 275 = 259
\)
Rearranging gives:
\(
100C + 10B - A = 534
\)
\(
100C + 10B - A - 275 = 518
\)
Rearranging gives:
\(
100C + 10B - A = 793
\)
This is not possible since \(100C + 10B\) cannot exceed 999.
\(
100C + 10B - A - 275 = 0
\)
Rearranging gives:
\(
100C + 10B - A = 275
\)
Now we can solve for A, B, and C:
- Since \(100C + 10B = 275 + A\), we can try different values for A (0-9) and see if we can find suitable values for B and C.
\(
100C + 10B = 275 + 5 = 280
\)
- \(C = 2\) and \(B = 8\) gives us \(CB5 = 285\).
\(
100C + 10B = 275 + 6 = 281
\)
- This does not yield valid digits for B and C.
\(
100C + 10B = 275 + 4 = 279
\)
- \(C = 2\) and \(B = 7\) gives us \(CB5 = 275\).
After testing various values, we find:
- \(C = 2\)
- \(B = 7\)
- \(A = 5\)
- A = 6
- B = 4
- C = 2
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - PLAYING WITH NUMBERSEXERCISE - Exercise 5D9videos
PLAYING WITH NUMBERS
- BOOK - RS AGGARWALCHAPTER - PLAYING WITH NUMBERSEXERCISE - Test Paper-511videos
PLAYING WITH NUMBERS
- BOOK - RS AGGARWALCHAPTER - PLAYING WITH NUMBERSEXERCISE - Exercise 5B15videos
PLAYING WITH NUMBERS
- BOOK - RS AGGARWALCHAPTER - PIE CHARTS EXERCISE - Exercise 24B4videos
PIE CHARTS
- BOOK - RS AGGARWALCHAPTER - POLYGONSEXERCISE - EXERCISE 14B14videos
POLYGONS
Similar Questions
Knowledge Check
- Question 1 - Select One
Replace A,B,C by suitable numberals:
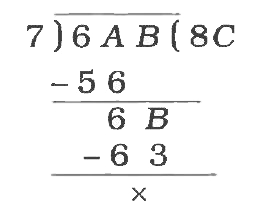 AA=1,B=3,C=9BA=2,B=3,C=9CA=1,B=6,C=9DA=1,B=6,C=8
AA=1,B=3,C=9BA=2,B=3,C=9CA=1,B=6,C=9DA=1,B=6,C=8
Replace A,B and C by suitable numerals. 4CB6+369A 8173
View SolutionReplace A,B and C by suitable numerals. AB×BA (B+1)CB
View SolutionReplace A,B and C by suitable numerals. AB ×3 CAB
View SolutionReplace A,B and C by suitable numerals. A+A A BA
View SolutionReplace A,B,C by suitable numberals:
57A−CB8 293View SolutionReplace A,B,C,D by suitable numerals in the following
6AB5+D58C 9351View SolutionFind the values of A,B and C in the following
35A−CB8 183View Solution

