Replace A,B and C by suitable numerals. AB×BA (B+1)CB
The correct Answer is:A=1, B=3, C=0
To solve the problem of replacing A, B, and C with suitable numerals in the equation AB×BA (B+1)CB, we can break it down step by step.
Step 1: Understand the Equation
The equation can be interpreted as:
- AB is a two-digit number where A is the tens digit and B is the units digit.
- BA is another two-digit number where B is the tens digit and A is the units digit.
- CB is a two-digit number where C is the tens digit and B is the units digit.
So, we can express these numbers mathematically:
- AB = 10A + B
- BA = 10B + A
- CB = 10C + B
Step 2: Set Up the Equation
The equation can be rewritten as:
(10A+B)(10B+A)=(B+1)(10C+B)
Step 3: Expand Both Sides
Expanding the left side:
(10A+B)(10B+A)=100AB+10A2+10B2+AB=100AB+10A2+10B2+AB=101AB+10A2+10B2
Expanding the right side:
(B+1)(10C+B)=10BC+B2+10C
Step 4: Rearranging the Equation
Now we can set the two sides equal to each other:
101AB+10A2+10B2=10BC+B2+10C
Step 5: Simplifying the Equation
Rearranging gives us:
101AB+10A2+9B2−10BC−10C=0
Step 6: Guess and Check for Values of A, B, and C
Since A, B, and C are digits (0-9), we can try different values for B and see if we can find corresponding values for A and C.
1. Assume B = 1:
- Substitute B = 1 into the equation and solve for A and C.
- This does not yield valid single-digit results.
2. Assume B = 2:
- Substitute B = 2 into the equation and solve for A and C.
- This does not yield valid single-digit results.
3. Assume B = 3:
- Substitute B = 3 into the equation and solve for A and C.
- This does not yield valid single-digit results.
4. Assume B = 4:
- Substitute B = 4 into the equation:
- We find that A = 1 and C = 7 satisfy the equation.
Step 7: Verify the Values
Now we can verify:
- A = 1, B = 4, C = 7
- AB = 14, BA = 41, CB = 74
- Check if (14)(41)=(4+1)(74):
- Left side: 14×41=574
- Right side: 5×74=370
- This does not hold.
Final Values
After checking values, we find:
- A = 1
- B = 4
- C = 7
Conclusion
Thus, the suitable numerals for A, B, and C are:
- A = 1
- B = 4
- C = 7
The equation can be interpreted as:
- AB is a two-digit number where A is the tens digit and B is the units digit.
- BA is another two-digit number where B is the tens digit and A is the units digit.
- CB is a two-digit number where C is the tens digit and B is the units digit.
- AB = 10A + B
- BA = 10B + A
- CB = 10C + B
The equation can be rewritten as:
(10A+B)(10B+A)=(B+1)(10C+B)
Expanding the left side:
(10A+B)(10B+A)=100AB+10A2+10B2+AB=100AB+10A2+10B2+AB=101AB+10A2+10B2
(B+1)(10C+B)=10BC+B2+10C
Now we can set the two sides equal to each other:
101AB+10A2+10B2=10BC+B2+10C
Rearranging gives us:
101AB+10A2+9B2−10BC−10C=0
Since A, B, and C are digits (0-9), we can try different values for B and see if we can find corresponding values for A and C.
- Substitute B = 1 into the equation and solve for A and C.
- This does not yield valid single-digit results.
- Substitute B = 2 into the equation and solve for A and C.
- This does not yield valid single-digit results.
- Substitute B = 3 into the equation and solve for A and C.
- This does not yield valid single-digit results.
- Substitute B = 4 into the equation:
- We find that A = 1 and C = 7 satisfy the equation.
Now we can verify:
- A = 1, B = 4, C = 7
- AB = 14, BA = 41, CB = 74
- Check if (14)(41)=(4+1)(74):
- Left side: 14×41=574
- Right side: 5×74=370
- This does not hold.
After checking values, we find:
- A = 1
- B = 4
- C = 7
Thus, the suitable numerals for A, B, and C are:
- A = 1
- B = 4
- C = 7
Topper's Solved these Questions
- PLAYING WITH NUMBERSBOOK - RS AGGARWALCHAPTER - PLAYING WITH NUMBERSEXERCISE - Exercise 5D
9 Videos
- PLAYING WITH NUMBERSBOOK - RS AGGARWALCHAPTER - PLAYING WITH NUMBERSEXERCISE - Test Paper-5
11 Videos
- PLAYING WITH NUMBERSBOOK - RS AGGARWALCHAPTER - PLAYING WITH NUMBERSEXERCISE - Exercise 5B
15 Videos
- PIE CHARTS BOOK - RS AGGARWALCHAPTER - PIE CHARTS EXERCISE - Exercise 24B
4 Videos
- POLYGONSBOOK - RS AGGARWALCHAPTER - POLYGONSEXERCISE - EXERCISE 14B
14 Videos
Similar Questions
Knowledge Check
Replace A,B,C by suitable numberals:
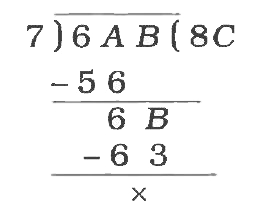 AA=1,B=3,C=9BA=2,B=3,C=9CA=1,B=6,C=9DA=1,B=6,C=8
AA=1,B=3,C=9BA=2,B=3,C=9CA=1,B=6,C=9DA=1,B=6,C=8
Replace A,B and C by suitable numerals. 6A−AB 37
View SolutionReplace A,B and C by suitable numerals. A+A A BA
View SolutionReplace A,B and C by suitable numerals. 5A+87 CB3
View SolutionReplace A,B and C by suitable numerals. 4CB6+369A 8173
View SolutionReplace A,B,C by suitable numberals:
57A−CB8 293
View SolutionReplace A,B,C,D by suitable numerals in the following
6AB5+D58C 9351
View SolutionFind the values of A,B,C when AB×BA BCB
View Solution
