For a uniformly charged sphere electric field inside the sphere is given as , where is volume charge density and is the position vector of the point with respective to centre For a given charge distribtution if the electric field at a point (P) is , and now some part of charge distribution is removed to form a cavity, electric field at same point (P) only due to removed part is . Then finally electric field at point P is .
There is a uniformly charged sphere of volume charge density and radius R with a cavity of radius as shown in the diagram.

A

B

C

D

Text Solution
See it carefully that electric field is same throughout the volume of cavity and equal to that at centre
|
Topper's Solved these Questions
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - (PROBLEM SOLUTION) COMPREHENSION - II (COMPREHENSION BASED TYPE)
2 Videos
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - ASSERTION REASONING TYPE
1 Videos
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - COMPREHENSION BASED (COMPREHENSION - II)
3 Videos
- ELECTROMAGNETIC WAVE & WAVE OPTICSBOOK - FIITJEECHAPTER - ELECTROMAGNETIC WAVE & WAVE OPTICSEXERCISE - ASSIGNMENT PROBLEMS Objective : Level-II (MULTI CHOICE SINGLE CORRECT)
21 Videos
- ELECTROSTATICSBOOK - FIITJEECHAPTER - ELECTROSTATICSEXERCISE - Example
14 Videos
Similar Questions
Knowledge Check
The electric field due to a uniformly charged sphere is maximum at :
Acentre of sphereBinside the surfaceCat the surfaceDat infinite distance from the centreA hollow sphere of copper is positively charged. Then the electric field inside the sphere is
Athe same as the field at the surfaceBgreater than the field at the surfaceCess than the field at the surface but not zeroDzeroElectric field intensity at point P due to charge distributed over a sphere is:
 AE=14πε0qrBE=σR2ε0r2CE=14πε0qrDE=0
AE=14πε0qrBE=σR2ε0r2CE=14πε0qrDE=0
Electric field intensity at point P due to charge distributed over a sphere is:
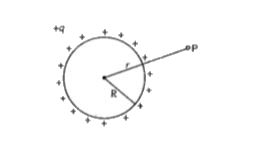
View SolutionFind the electric field at an arbitrary point of a sphere carrying a charge uniformly distributed over its volume.
View SolutionFind the electric field at an arbitrary point of a sphere carrying a charge uniformly distributed over its volume.
View SolutionElectric field in cavity due to sphere ,cylinder and plate||Charge distribution on conductors
View SolutionIntensity of electric field inside a uniformly charged hollow sphere is
View Solution

_PHY_E05_288_Q01.png)