Sides of two similar triangles are in the ratio 4 : 9 Areas of these triangles are in the ratio Solution in Kannada
2:3
4:9
81:16
16:81
The correct Answer is:D
Step by step video, text & image solution for Sides of two similar triangles are in the ratio 4 : 9 Areas of these triangles are in the ratio by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - TRIANGLESEXERCISE - Exercise 2.5
17 Videos
- TRIANGLESBOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - TRIANGLESEXERCISE - Exercise 2.6 (Optional)
8 Videos
- TRIANGLESBOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - TRIANGLESEXERCISE - Exercise 2.3
16 Videos
- SURFACE AREAS AND VOLUMES BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMES EXERCISE - EXERCISE 15.4
5 Videos
Similar Questions
Sides of triangles are given below. Determine which of them are right triangles.
In case of a right triangle , write the length of its hypotenuse.
3cm, 24 cm, 25 cm
View SolutionIn Fig . PS is the bisecto of ∠QPRofΔPQR . Prove that
QSSR=PQPR
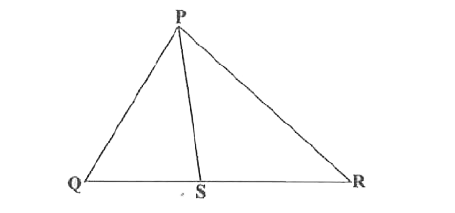
View SolutionState which pairs of triangles in Fig are similar. Write the similarity criterion used by you for answering the question also write the pairs of similar triangles in the symbolic form:
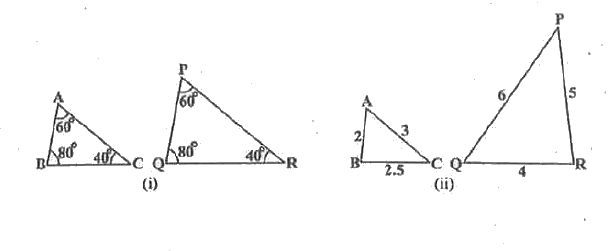
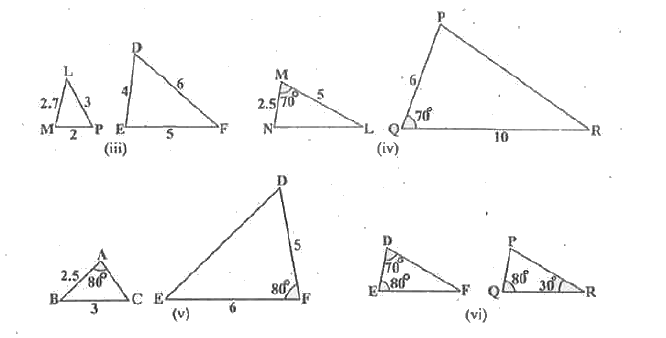
View SolutionA drinking glass is in the shape of a frustum of a cone of height 14cm . The diameters of its two circular ends. are 4cm and 2cm . Find the capacity of the glass.
View SolutionIf the ratio of the sides of two similar triangles be 4:9, then ratio of areas will be
View SolutionThe sides of two similar triangles are 4 : 9 . What is the ratio of their area?
View SolutionThe perimeter of two similar triangles are in 4:9 ratio, the ratio of their corresponding sides is…………..
View SolutionThe ratio of the areas of two similar triangles is 1:4 then the ratio of their corresponding sides………….
View SolutionSides of two similar triangles are in the ratio 4 : 9 Areas of these triangles are in the ratio
View SolutionSides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio
View SolutionThe side of two similar triangles are 4:9. What is the ratio of their area?
View Solutionਦੋ ਸਮਰੂਪ ਤ੍ਰਿਭੁਜਾਂ ਦੀਆਂ ਭੁਜਾਵਾਂ 4:9 ਦੇ ਅਨੁਪਾਤ ਵਿਚ ਹਨ। ਇਨ੍ਹਾਂ ਤ੍ਰਿਭੁਜਾਂ ਦੇ ਖੇਤਰਫਲਾਂ ਦਾ ਅਨੁਪਾਤ ਹੈ:
View SolutionTick the correct answer and justify : Sides of two similar triangles are in the ratio 4:9. Areas of these triangles are in the ratio
View SolutionSides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio___
View SolutionTick the correct answer and justify : Sides of two similar triangles are in the ratio 4:9. Areas of these triangles are in the ratio
View SolutionCalculate the ratio of the area of two similar triangles if the sides of the triangles are in the ratio of 9:4.
View Solution
