A block of mass 8 kg is sliding on a surface inclined at an angle of $45^{\circ}$ with the horizontal. Calculate the acceleration of the block. The coefficient of friction between the block and surface is 0.6. (Take $g = 10 m / s^{2}$ )

Video Solution

Text Solution

Verified by Experts

Here $m = 8 k g, θ = 45^{\circ}, a = ?, μ = 0.6$
Here, $tan θ = {tan 45}^{\circ} = 1 > μ (= 0.6)$

So, body starts moving and acceleration of the body.
$a = g (sin θ - μ cos θ)$
$∴ a = 10 ({sin 45}^{\circ} - 0.6 {cos 45}^{\circ})$
$= 10 [0.707 - 0.6 \times 0.707]$
$= 10 \times 0.707 (1 - 0.6)$
$= 10 \times 0.707 \times 0.4$
$= 2.828 m / s^{2}$

|

Updated on:21/07/2023

Class 12 PHYSICS LAWS OF MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A block of mass 20 kg is acted upon by a force $F = 30 N$ at an angle $53^{\circ}$ with horizontal in downward direction as shown. The coefficient of friction between the block and the forizontal surface is $0.2$ . The friction force acting on the block by the ground is $(g = 10 \frac{m}{s^{2}}$ )
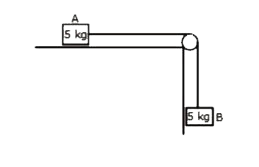
A40.0 N
B30.0 N
C18.0 N
D44.8 N
Question 2 - Select One
A block of mass 5 kg resting on a horizontal surface is connected by a cord, passing over a light frictionless pulley to a hanging block of mass 5 kg. The coefficient of kinetic friction between the block and surface is 0.5. Tension in the cord is (take, $g = 9.8 m s^{- 2}$ )

A49 N
Bzero
C36.75 N
D12.75 N
Question 3 - Select One
A block of mass 1 kg is pushed up a surface inclined to horizontal at an angle of $30^{\circ}$ by a force of 10 N parallel to the inclined surface as shown in the figure.
The coefficient of friction between block and the incline is 0.1. If the block is pushed up by 10 m along the incline, then the work against gravity is (Take $g = 10 m s^{- 2}$ )

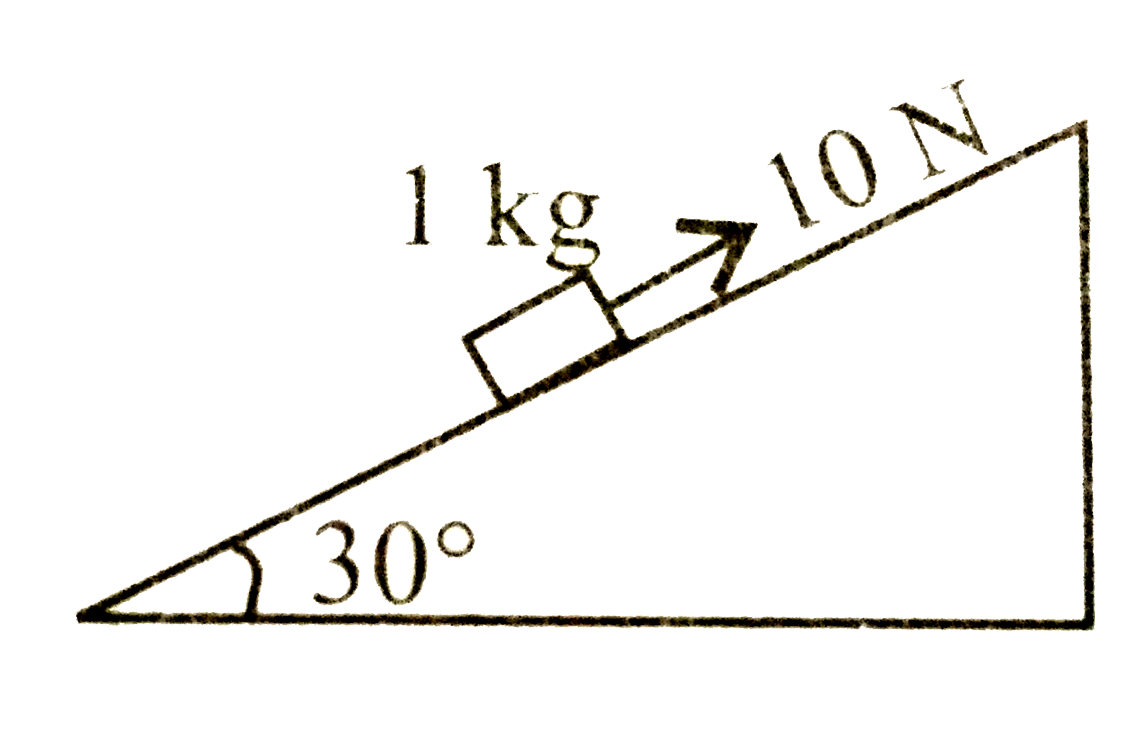A10 J
B50 J
C100 J
D150 J

AAKASH INSTITUTE-LAWS OF MOTION-Example

Home
Ask Doubt
Profile