A 2 kg mass is suspended by a rubber cord 2 m long and of cross-section 0.5 cm. It is made to describe a horizontal circle of radius 50cm in 4 times a second. Find the extension of the cord.(Young's modulus $Y = 7 \times 10^{8} N m^{- 2}$ )

Text Solution

Verified by Experts

Given, m=2kg, $ω = 2 π f = 2 π \times 4 = 8 π {rads}^{- 1}, r = 0.5$ m

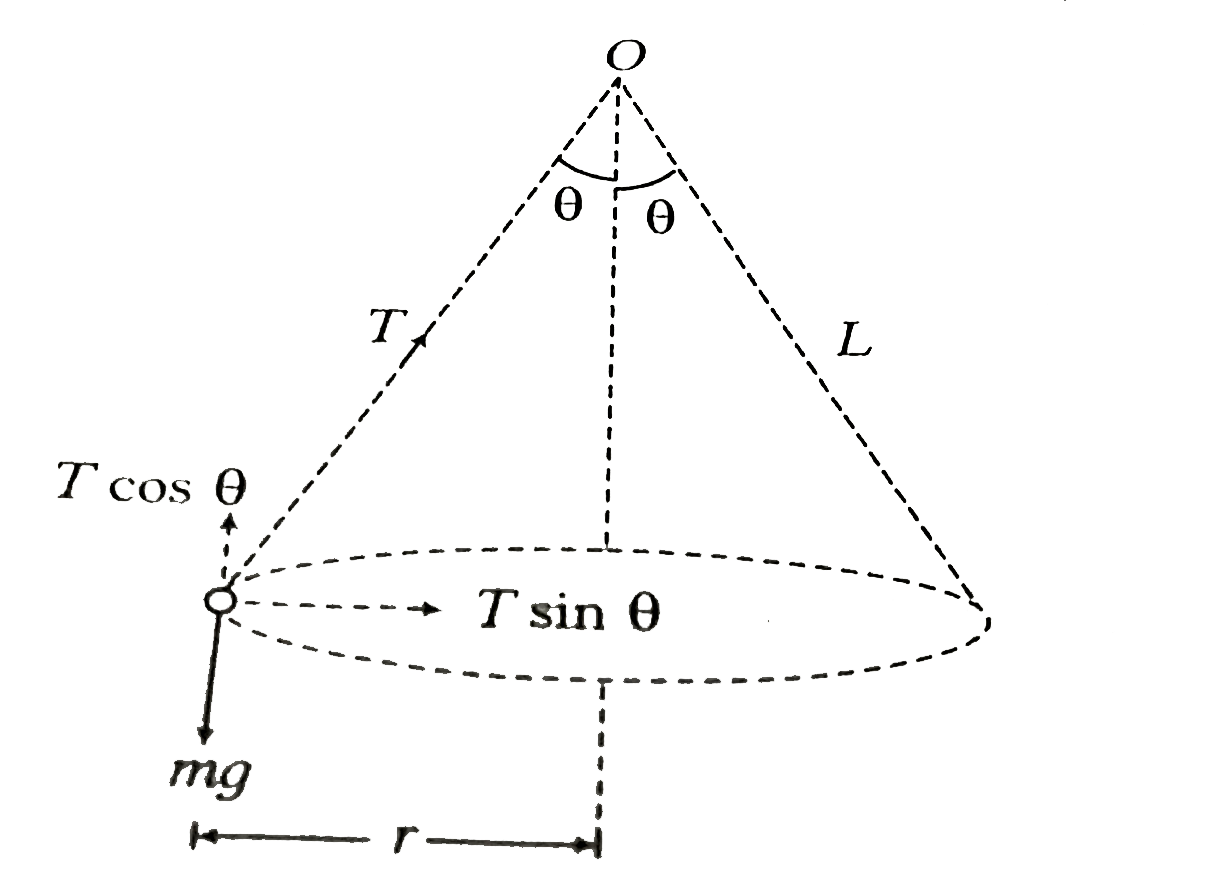
From the figure,
$T = cos θ = m g$ and $T sin θ = \frac{m v^{2}}{r} = m ω^{2}$
Tension in cord
$T = \sqrt{{(T sin θ)}^{2} + {(T cos θ)}^{2}}$
$= \sqrt{{(m ω^{2} r)}^{2} + {(m g)}^{2}}$
$T = \sqrt{{[2 \times {(8 π)}^{2} \times 0.5]}^{2} + {[2 \times 10]}^{2}} = 631 N$
The extension of cord,
$Δ L = \frac{T L}{A Y} = \frac{631 \times 2}{0.5 \times 10^{- 4} \times 10^{8}}$
$= 360 \times 10^{- 4} m = 3.60 c m$

|

Updated on:21/07/2023

Class 11 PHYSICS PROPERTIES OF SOLIDS AND FLUIDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A body of mass 1 kg is fastened to one end of a steel wire of cross- section area $3 \times 10^{- 6} m^{2}$ and is rotated in horizantal circle of radius 20 cm with a constant speed $2 m / s$ . Find the elongation of the wire $(Y = 2 \times 10^{11} N / m^{2})$
A $0.33 \times 10^{- 5} m$
B $0.67 \times 10^{- 5} m$
C $2 \times 10^{- 5} m$
D $4 \times 10^{- 5}$
Question 2 - Select One
A rubber cord of cross-sectional area 2 $c m^{2}$ has a length of 1 m. When a tensile force of 10 N is applied, the length of the cord increases by 1 cm. What is the Young's modulus of rubber ?
A $2 \times 10^{6} N / m^{2}$
B $5 \times 10^{6} N / m^{2}$
C $0.5 \times 10^{6} N / m^{2}$
D $0.2 \times 10^{6} N / m^{2}$

DC PANDEY-ELASTICITY-Example

Home
Ask Doubt
Profile