(Diet problem) A dietician has to develop a special diet using two foods and . Each packet (containing ) of food contains 12 units of calcium, 4 units of iron, 6 units of cholesterol and 6 units of vitamin A. Each packet of the same quantity of food contains 3 units of calcium, 20 units of iron, 4 units of cholesterol and 3 units of vitamin A. The diet requires atleast 240 units of calciums atleast 460 units of iron and at most 300 units of cholesterol. How many packets of each food should be used to minimise the amount of vitamin A in the diet? What is the minimum amount of vitamin A? How many packets of each food should be used to maximise the amount of vitamin A in the diet? What is the maximum amount of vitamin A in the diet?
Let the dietician used packets of food and packets of food . Then
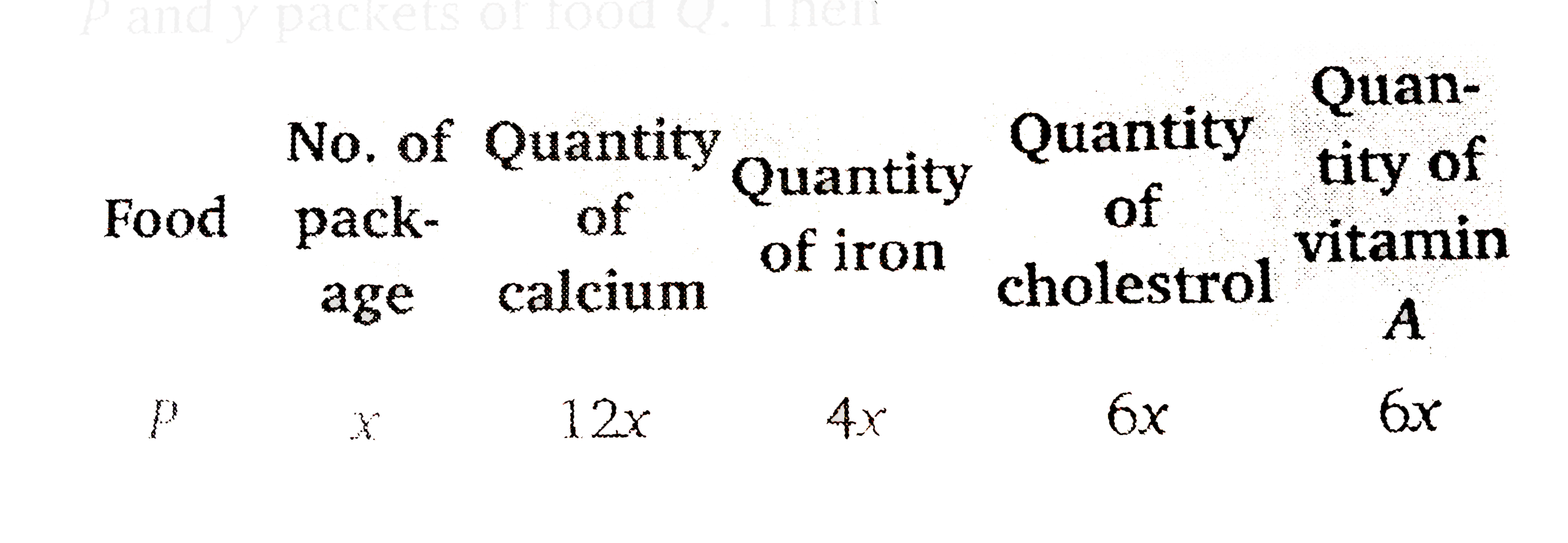
Minimise …………………1
and constraints
………………2
……………….3
..................4
....................5
First draw the graph of the line .
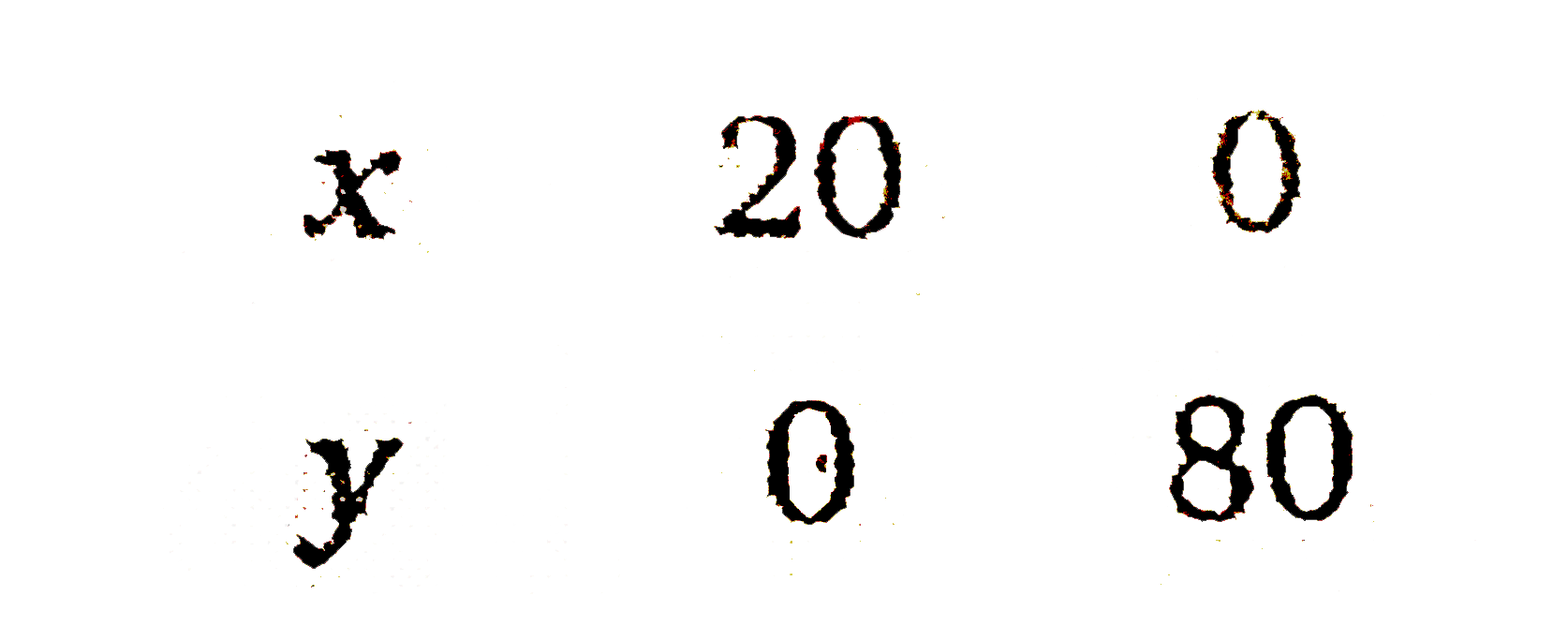
Put in the inequation ,
(False)
Thus, the half plane does not contains origin.
Now, draw the graph of the line
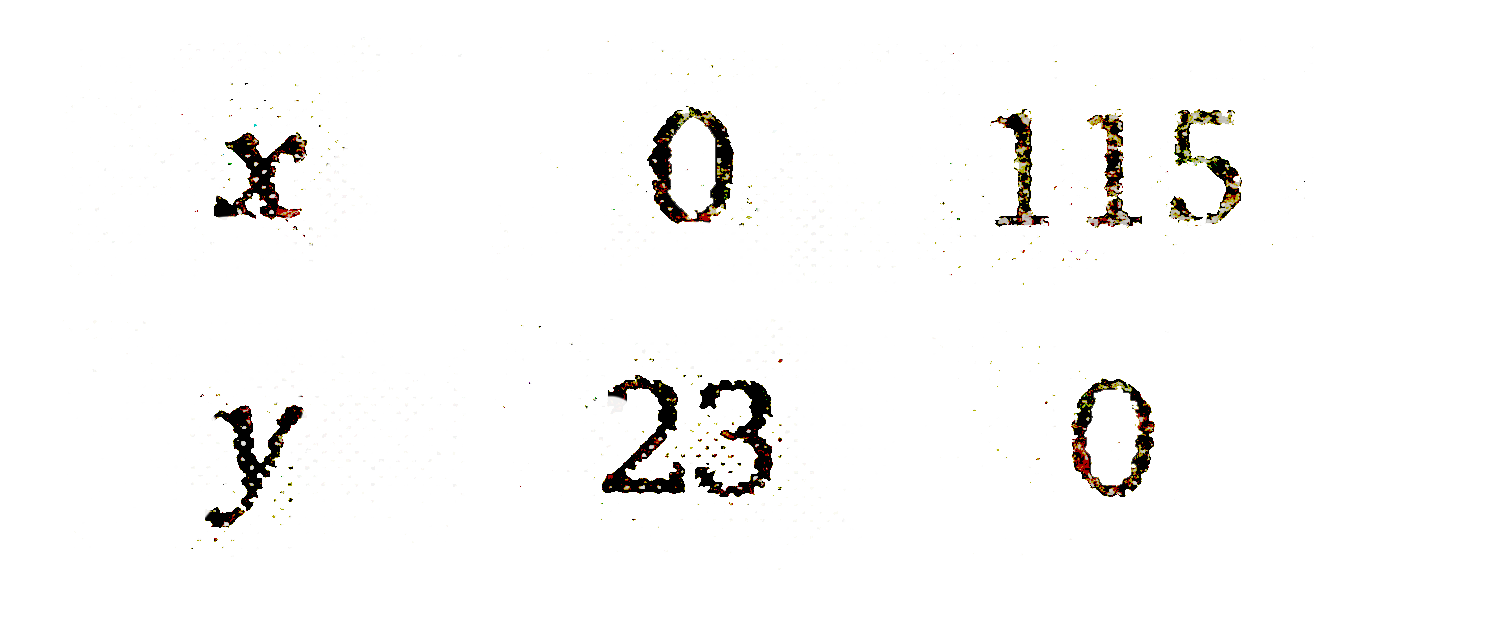
Put in the equation ,
(False)
Thus, the half plane does not contain the origin.
Now draw the graph of the line

Put in the inequation
(True)
Therefore, half plane contains the origin. Since, , so the feasible region lies in first quadrant.
From equations and . The point of intersection is .
Similarly from equations and the point of intersection is . Thus the feasible region is ABCA.
Its vertices are and
We find the value of at these vertices.
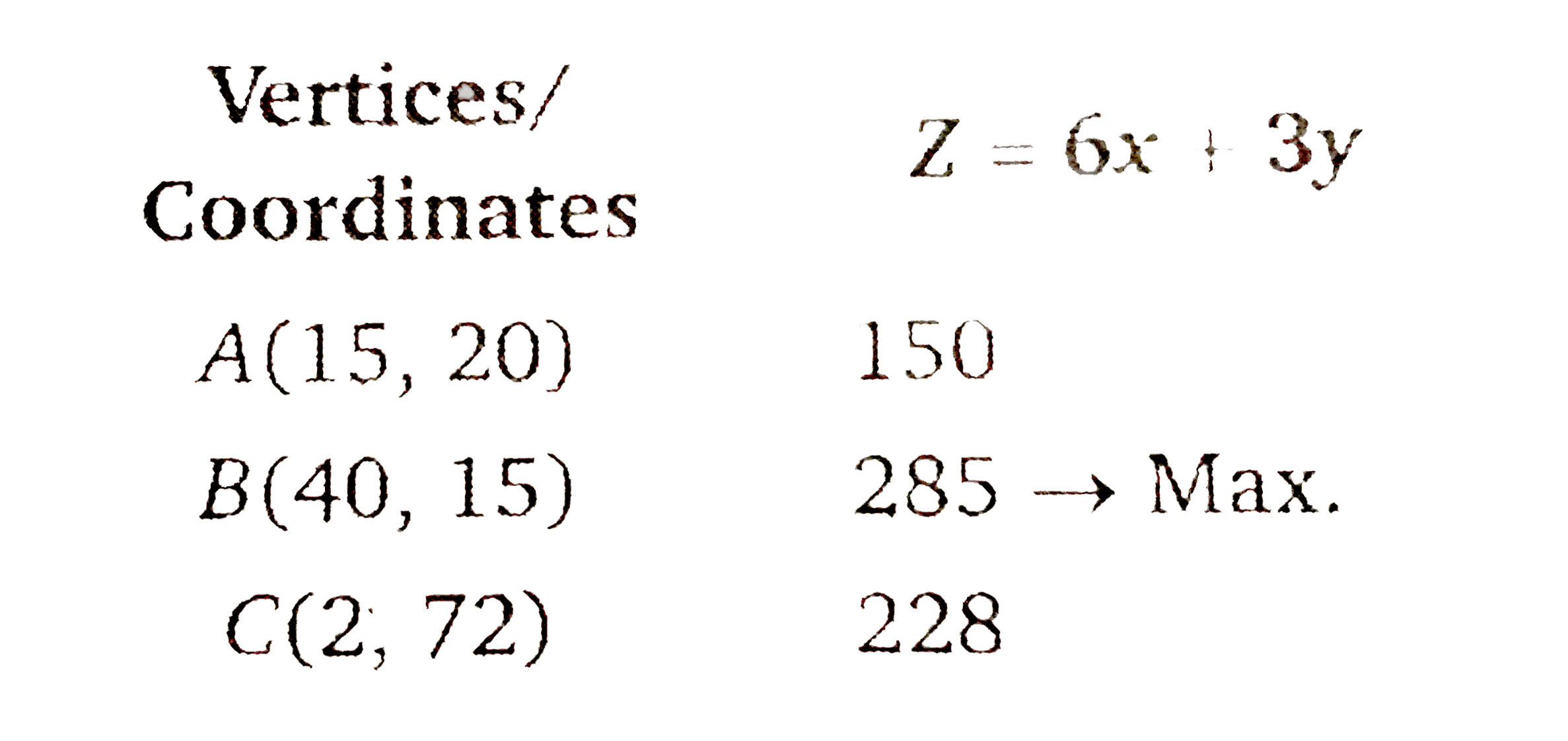
Thus, the maximum value of is 285 at point . Therefore, to obtain the maximum vitamin A, 40 packages of food and 15 packages of food should be produced. THus the maximum quantity of vitamin is 285.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exercise 12.211videos
LINEAR PROGRAMMING
- BOOK - NAGEEN PRAKASHANCHAPTER - INVERES TRIGONOMETRIC FUNCTIONSEXERCISE - Miscellaneous Exercise (prove That )9videos
INVERES TRIGONOMETRIC FUNCTIONS
- BOOK - NAGEEN PRAKASHANCHAPTER - MATRICESEXERCISE - Miscellaneous Exerice15videos
MATRICES
Similar Questions
(Diet problem) A dietician has to develop a special diet using two foods P and Q. Each packet (containing 30 g) of food P contains 12 units of calcium, 4 units of iron, 6 units of cholesterol and 6 units of vitamin A. Each packet of the same quantity of food Q contains 3 units of calcium, 20 units of iron, 4 units of cholesterol and 3 units of vitamin A. The diet requires atleast 240 units of calcium, atleast 460 units of iron and at most 300 units of cholesterol. How many packets of each food should be used to minimise the amount of vitamin Am the diet? What is the minimum amount of vitamin A?
View SolutionRefer to Example 9. How many packets of each food should be used to maximise the amount of vitamin A in the diet? What is the maximum amount of vitamin A in the diet?
View Solution
Knowledge Check
- Question 1 - Select One
Structural unit of vitamin-A is
A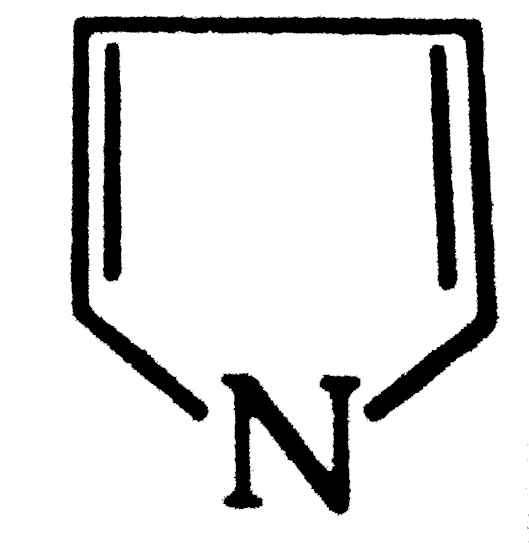 B
B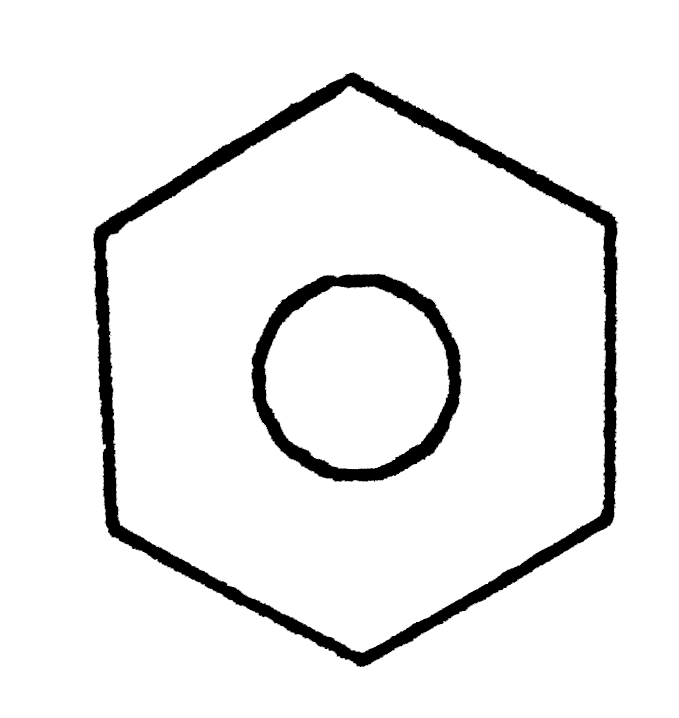 C
C D
D
- Question 2 - Select One
Food X contains 4 units of vitamin A per gram and 7 units of vitamin B per gram and cost 15 paise per gram . Food Y contains 6 units of vitamin A per gram and 11 units of vitamin B per gram and cost 22 paise per gram . The daily minimum requirement of vitamin A and B are 90 units and 130 units respectively . The formulation of LPP to minimize the cost is
Az = 15x +22y, subject to constraints 4x+6y≥90,7x+11y≥130,x≥0y≥0Bz = 6x +5y, subject to constraints 4x+3y≥90,7x+11y≥130,x≥0y≥0Cz = 15x +62y, subject to constraints 4x+6y≥90,7x+11y≥450,x≥0y≥0Dz = 15x +22y, subject to constraints 4x+6y≥90,7x+45y≥260,x≥0y≥0 - Question 3 - Select One
Funtion units of food absorption are
AVilliBBrunner's glandsCCrypts of LieberkuhnDPeyer's patches
A diet for a sick person must contain at least 4000 units of vitamins, 50 units of minerals and 1400 units of calories. Two foods A and B are available at a cost of Rs. 5 and Rs. 4 per unit respectively. One unit of food A contains 200 units of vitamins, 1 unit of minerals and 40 units of calories whereas one unit of food B contains 100 units of vitamins, 2 units of minerals and 40 units of calories. Find what combination of the food A and B should be used to have least cost but it must satisfy the requirements of the sick person.
View SolutionFood F1 contains 5 units of vitamin A and 6 units of vitamin B per gram and costs 20 p/gm. Food F2 contains 8 units of vitamin A and 10 units of vitamin B per gram and costs 30 p/gm. The daily requirements of A and B are at least 80 and 100 units respectively. Formulate the problem as a linear programming problem to minimize cost.
View SolutionA farmer mixes two brands P and Q of cattle feed. Brand P, costing Rs 250 per bag, contains 3 units of nutritional element A, 2.5 units of element B and 2 units of element C. Brand Q costing Rs 200 per bag contains 1.5 units of nutritional element A, 11.25 units of element B, and 3 units of element C. The minimum requirements of nutrients A, B and C are 18 units, 45 units and 24 units respectively. Determine the number of bags of each brand which should be mixed in order to produce a mixture having a minimum cost per bag? What is the minimum cost of the mixture per bag?
View SolutionA farmer mixes two brands P and Q of cattle feed. Brand P costing Rs. 250 per bag, contains 3 units of nutritional element A, 2.5 units of element B and 2 units of element C. Brand Q costing Rs. 200 per bag contains 1.5 units of nutritonal element A, 11.25 units of element B and 3 units of element C. The minimum requirements of nutrients A,B and C are 18 units, 45 units and 24 units respectively. Determine the number of bags of each brand which should be mixed in order to produce a mixture having a minimum cost per bag? What is the minimum cost of the mixture per bag?
View SolutionFood X contains 6 units of vitamin A per gm and 7 units of vitamin B per gm and costs ₹ 2.00 per gm.Food Y contains 8 units of Vitamin A per gm and 12 units of vitamin B per gm and costs ₹ 2.50 per gm.The daily minimum requirement of vitamin A and vitamin V are 100 units and 120 units respectively.Formulate the above as a linear programming problem to minimize the cost.
View Solution

