Derive an expression for electrical conductivity.Solution in Kannada
Let .n. be the number density of electrons. Let .L. be the length of the conductor, .A. be the area of cross-section of the conductor.
Let vd be the drift velocity of the electrons. Let Δx be a small length.
Electrons drift in a direction opposite to the electric field. Number of electrons in.Δx. and area of cross-section .A. is equal to (AΔx)n.
Charge on these electrons = (nAΔx)e
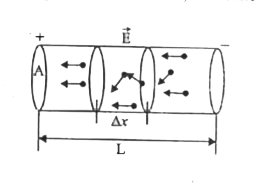
If Δt is the time taken for effective displacement of electrons then rate of flow charge = nAe(ΔxΔt). By definition, electric current I = rate of flow of charge.
i.e., I=nAevd ......(1)
where, average velocity of electrons with which it drifts against the direction of electric field is known as drift velocity (vd).
Let .a. be the acceleration of electrons. Let .E. be the electric field intensity. Force on electrons,
F=ma
i.e., eE=ma
but= a= vτt where .τ. is the relaxation time. Relaxation time represents the average time taken for two successive collisions of electrons and ions in the lattice.
Hence, eE=mvdτ or vd=eEmτ ...(2)
We also know that electric potential difference between the ends of the conductor V = EL
vd=eVmτL substituting this in the expression (1)
We write,
I=nAe(eVmτL)
i.e., I=(nAe2mτL)V
R=mτLmAe2 is called the electric resistance of a conductor.
and K=(nAe2mτL) is called the electric conductance of a conductor.
the expression σ=nc2mτ is called the electrical conductivity and 1σ=mτ≠2 is called electrical resistivity.
Topper's Solved these Questions
- BOOK - SUBHASH PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - NUMERICAL PROBLEMS AND ANSWERS29videos
CURRENT ELECTRICITY
- BOOK - SUBHASH PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - THREE MARK QUESTIONS AND ANSWERS11videos
CURRENT ELECTRICITY
- BOOK - SUBHASH PUBLICATIONCHAPTER - COMMUNICATION SYSTEMS EXERCISE - THREE MARKS QUESTIONS WITH ANSWERS4videos
COMMUNICATION SYSTEMS
- BOOK - SUBHASH PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - NUMERICALS WITH SOLUTIONS21videos
DUAL NATURE OF RADIATION AND MATTER
Similar Questions
In the given circuit, calculate the effective resistance between A and B.
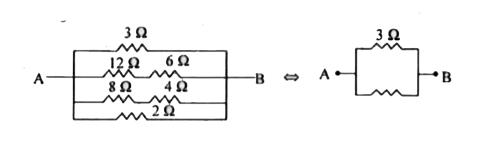 04:06View Solution
04:06View SolutionA uniform wire of resistance 9Ω is bent to form an equilateral triangle. What is the effective resistance between any two corners of the triangle.
05:06View SolutionOutline the production of an Amplitude Modulated Wave.
04:07View SolutionA photo has a wavelength of 5000 Å. Calculate its momentum.
View SolutionDerive an expression for the electric field intensity at a point outside a charged conducting sphere.
05:25View SolutionDerive an expression for the electric potential due to a point charge.
03:17View SolutionDefine relaxation time . Derive the expression for electrical conductcity of material in terms of relaxation time .
05:25View SolutionDerive an expression for electrostatic potential due to an electric dipole.
06:31View SolutionDerive an expression for electrostatic potential due to an electric dipole.
03:56View SolutionDerive the expression for power P=Vi in electrical circuit.
07:11View SolutionDerive an expression for electrostatic potential due to an electric dipole.
05:17View SolutionDerive an expression for electric potential due to a point charge
04:53View SolutionDerive an expression for coefficient of thermal conductivity of a material.
04:16View SolutionDerive the expression for electric intensity at a point on the axis of a dipole
View SolutionDerive an expression for torque on a dipole placed in an electric field.
View Solution

