Derive an expression for electrostatic potential due to an electric dipole. Solution in Tamil

Video Solution

Text Solution

Verified by Experts

Electrostatic potential at a point due to an electric dipole: Consider two equal and opposite charges separated by a small distance 2a. The point P is located at a distance r from the midpoint of the dipole . Let $θ$ be the angle between the line OP and dipole axis AB. Let $r_{1}$ be the distance of point P from +q and $r_{2}$ be the distance of point P from -q.
Potential at P due to charge +q = $\frac{1}{4 π ε_{0}} \frac{q}{r_{1}}$
Potential at P due to charge $- q = - \frac{1}{4 π ε_{0}} \frac{q}{r_{2}}$
Total potential at the point P,
$V = \frac{1}{4 π ε_{0}} q (\frac{1}{r_{1}} - \frac{1}{r_{2}})$
Suppose if the point P is far away from the dipole such that $r > > a$ then equation can be expressed in terms of r. By the cosine law for triangle BOP,
$r_{1}^{2} = r^{2} + a^{2} - 2 r a cos θ = r^{2} (1 + \frac{a^{2}}{r^{2}} - \frac{2 a}{r} cos θ)$
m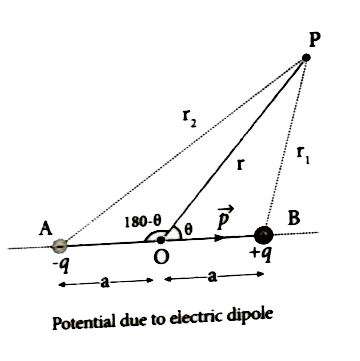
Since the point P is very far from dipole then $r > > a$ . As a result the term $\frac{a^{2}}{r^{2}}$ is very small and can be neglected. Therefore
$r_{1}^{2} = r^{2} (1 - 2 a \frac{cos θ}{r}) (or) r_{1} = r \frac{{(1 - \frac{2 a}{r} cos θ)}^{1}}{2} (or)$
$\frac{1}{r_{1}} = \frac{1}{r} \frac{{(1 - \frac{2 a}{r} cos θ)}^{1}}{2}$
since $\frac{a}{r} < < 1$ , we can use binominal theorem and retain the terms up to first order
$\frac{1}{r_{1}} = \frac{1}{r} (1 + \frac{a}{r} cos θ)$
Similarly applying the cosine law for triangle AOP
$r_{2}^{2} = r^{2} + a^{2} - 2 r a cos (180 - θ) Since cos (180 - θ) = - cos θ$ we get
$r_{2}^{2} = r^{2} + a^{2} + 2 r a cos θ$
Neglecting the term $\frac{a^{2}}{r^{2}}$ ( because $r > > a$ )
$r_{2}^{2} r^{2} (1 + \frac{2 a cos θ}{r}) (or) r_{2} = r \frac{{(1 + \frac{2 a cos θ}{r})}^{1}}{2}$
Using Binomial theorem , we get
$\frac{1}{r_{2}} = \frac{1}{r} \frac{1 - a cos θ}{r})$
Substituting equations (3) and (2) in equation (1)
$V = \frac{1}{4 π ε_{0}} q [\frac{1}{r} (1 + a \frac{cos θ}{r}) - \frac{1}{r} (1 - a (cos θ)] = \frac{q}{4 π ε_{0}} [\frac{1}{r} (1 + a \frac{cos θ}{r} - 1 + a \frac{cos θ}{r})]$
$V = \frac{1}{4 π ε_{0}} \frac{2 a q}{r^{2}} cos θ$
But the electric dipole moment p = 2qa and we get,
$V = \frac{1}{4 π ε} (\frac{p cos θ}{r^{2}})$
Now we can write p cos $θ = \to p . \to r$ where $ˆ r$ is the unit vector from the point O to point P . Hence the electric potential at a point P due to an electric dipole is given by
$V = \frac{1}{4 π ε_{0}} \frac{\to . \to r}{r^{2}} (r > > a)$
Equation ( 4) is valid for distances very large compared to the size of the dipole . But for a point dipole the equation ( 4) is valid for any distance.
Special cases:
Case (i) If the point P lies on the axial line of the dipole on the side of $+ q$ then theta =0 . Then the electric potential becomes
$V = \frac{1}{4 π ε_{0}} \frac{P}{r^{2}}$
Case (ii) IF the point P lies on the axial line of the dipole on the side of -q then $θ 180^{\circ}$ then $V = \frac{1}{4 π ε_{0}} \frac{p}{r^{2}}$
Case (iii) If the point P lies on the equatorial line of the dipole then $θ = 90^{\circ}$ . Hence V= 0 .

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

FULL MARKS-ELECTROSTATICS -Textual Evaluation Solved ( III. Long Answer Questions: )

Home
Ask Doubt
Profile