The perpendicular from A on side BC of a ABC intersects BC at D such that DB = 3 CD. Prove that $2 A B^{2} = 2 A C^{2} + B C^{2}$ .

Video Solution

Text Solution

Verified by Experts

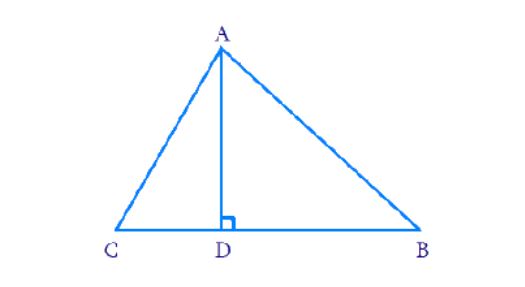
In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
In $Δ A B C$ , $A D ⊥ B C$ and $B D = 3 (C D)$
$\Rightarrow B D + C D = B C$
$\Rightarrow 3 C D + C D = B C$
$\Rightarrow 4 C D = B C$
$\Rightarrow C D = (\frac{1}{4}) B C$ ...... (1)
and, $B D = (\frac{3}{4}) B C$ ..... (2)
In $Δ A D C, ∠ A D C = 90 °$
$\Rightarrow {(A C)}^{2} = {(A D)}^{2} + {(C D)}^{2}$ [Using Pythagoras theorem]
$\Rightarrow {(A D)}^{2} = {(A C)}^{2} - {(C D)}^{2} ... . . (3)$
In $Δ A D B, ∠ A D B = 90 °$
$\Rightarrow {(A B)}^{2} = {(A D)}^{2} + {(B D)}^{2}$ [Using Pythagoras theorem ]
$\Rightarrow {(A B)}^{2} = {(A C)}^{2} - {(C D)}^{2} + B D^{2}$ [from equation(3)]
$\Rightarrow {(A B)}^{2} = {(A C)}^{2} + {(\frac{3}{4} B C)}^{2} - {(\frac{1}{4} B C)}^{2}$ [from equations(1) and (2)]
$\Rightarrow {(A B)}^{2} = {(A C)}^{2} + \frac{9 B C^{2} - B C^{2}}{16}$
$\Rightarrow {(A B)}^{2} = {(A C)}^{2} + 8 \frac{{(B C)}^{2}}{16}$
$\Rightarrow {(A B)}^{2} = {(A C)}^{2} + \frac{1}{2} {(B C)}^{2}$
Thus, ${(2 A B)}^{2} = {(2 A C)}^{2} + {(B C)}^{2}$

Updated on:21/07/2023

|

Class 10 MATHS TRIANGLES

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile