A container having base area A0. Contains mercury upto a height l0. At its bottom a thin tube of length 4l0 and cross-section area A(A<<A0) having lower end closed is attached . Initially the length of mercury in tube is 3l0. in reamining part 2 mole of a gas at temperature T is closed as shown in figure . Determine the work done (in joule) by gas if all mercury is displaced from tube by heating slowly the gas in the rear end of the tube by means of a heater. (Given : density of mercury = ρ, atmospheric pressure P0=2l0ρg,Cv of gas = 3/2R,A=(3/ρ)m2,l0=(1/9)m , all units is S.I.)
_S01_127_Q01.png)
The correct Answer is:5
If x is length of mercury in tube then pressure of gas
P'=P0+ρgl0+ρgx=3ρgl0+ρgx⇒W=−∫03/0(3ρgl0+ρgx)Adx=13.5ρgAl20=5
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -01
65 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -02
82 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
427 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
A glass tube of length l0 and of uniform cross-section is to be filled with mercury so that the length of the tube unoccoupied by mercury remains same at all temperatures. If γg=3α , calculate length of mercury column.
View SolutionA vertical tube of length 100 cm contains a mercury pallet of length 5 cm as shown in the figure. The length of the tube above mercury pallet if the tube is inverted is nearly: (atmospheric pressure = 75 cm Hg of Hg)
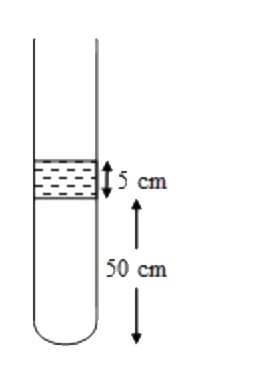
View Solution
Knowledge Check
A vertical tube of length 100 cm contains a mercury pallet of length 5 cm as shown in the figure. The length of the tube above mercury pallet if the tube is inverted is nearly: (atmospheric pressure = 75 cm Hg of Hg)
 A56 cmB57 cmC60 cmD50 cm
A56 cmB57 cmC60 cmD50 cm
A tube 1.5 m long and closed at on end is half filled with mercry and is then inverted with its open ends just dipping into a mercury tough.Find the height of mercury in the tube if the reading of the barometer is 0.75 m.
View SolutionA container of cross-section area 'S' and height 'h' is filled with mercury up to the brim. Then the container is sealed airtight and a hole of small cross section area 'S/n' (where 'n' is a positive constant) is puched in its bottom. Find out the time interval upto which the mercury will come out from the bottom hole.
[Take the atmospheric pressure to be equal to h0 height of mercury column: h>h0]
View SolutionA container of cross-section area 'S' and height 'h' is filled with mercury up to the brim. Then the container is sealed airtight and a hole of small cross section area 'S/n' (where 'n' is a positive constant) is puched in its bottom. Find out the time interval upto which the mercury will come out from the bottom hole.
[Take the atmospheric pressure to be equal to h0 height of mercury column: h>h0]
View SolutionAn adibatic cylindrical tube of cross-sectional area 1cm2 is closed at one end fitted with a piston at the other end. The tube contains 0.03g of an ideal gas . At 1 atm pressure and at the temperture of the surrounding, the length of the gas column. The pressure of the gas falls to 0.355atm. Find th speed of sound in the gas at atmospheric temperture.
View SolutionA pressure exerted by mercury at the bottom of a container of area of cross section 2cm2 is 10 Pa. Determine the weight of the mercury in the container.
View SolutionA pressure exerted by mercury at the bottom of a container of area of cross section 2cm2 is 10 Pa. Determine the weight of the mercury in the container.
View Solutiona glass tube of length 133cm and of uniform cross-section is to be filled with mercury so that the volume of the unoccupied by mercury remains the same at all temperatures. If cubical coefficient for glass and mercury are respectively 0.000026/∘C and 0.000182/∘C , calculate the length of mercury column.
View Solution
