A disc of mass 3 m and a disc of mass m are connected by a massless spring of stiffness k. The heavier is disc placed on the ground with the spring vertical and lighter disc on top from its equilibrium position the upper disc is pushed down by a distance δ and released. Then.
if δ>3mg/k, the lower disc will bounce up
if δ=2mg/k, maximum normal reaction from ground on lower disc =6mg
if δ=2mg/k, maximum normal reaction from ground on lower disc =4mg
if δ>4mg/k, the lower disc will bounce up
The correct Answer is:B, D
From energy conservation
_E01_067_Q01.png)
12kδ12=mg(δ1+x1)+12kx12
⇒δ12−2mgkδ1=15m2g2k2=0
⇒δ1=5mgk=δ=4mgk
⇒ If δ≥4mgk the lower disk will bounce up.
Now if δ=2mgk then maximum normal reaction
from ground on lower disk
N=3mg+k(x0+δ)=6mg
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column1videos
SIMPLE HARMONIC MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Asseration & Reason7videos
SIMPLE HARMONIC MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-01117videos
SIMPLE HARMONIC MOTION
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)25videos
RACE
- BOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS4videos
TEST PAPER
Similar Questions
Figure shows two discs of same mass m. They are rigidy attached to a spring of stiffness k. The system is in equlibrium. From this equilibrium position, the upper disc is pressed down slowly by a distance x and released. Find the minimum vlue of x, if the lower disc is just lifted off the ground.
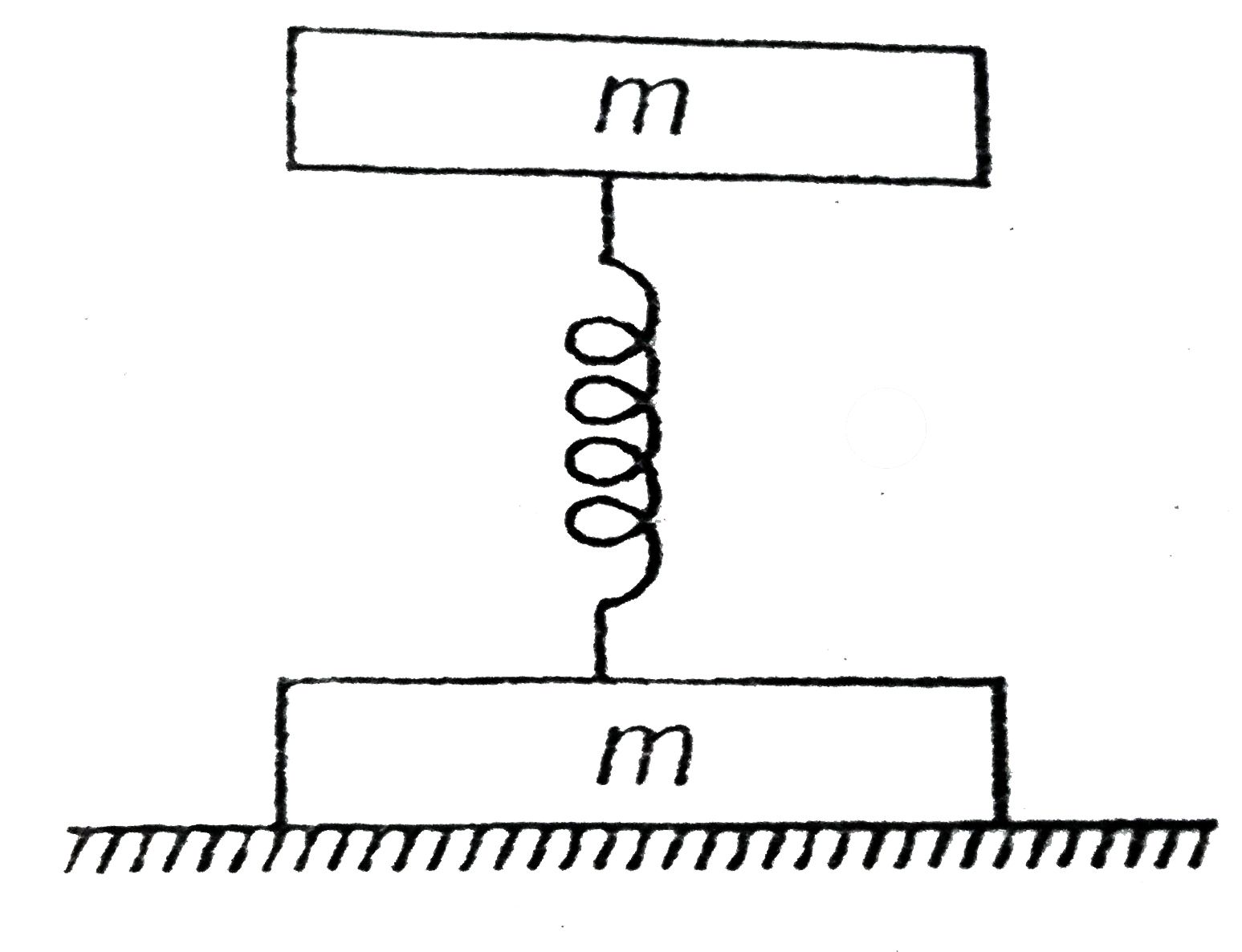 View Solution
View SolutionA disk of mass m is connected to two springs of stiffness k1andk2 as shown in the figure. Find the angular frequency of the system for small oscillation. Disc can roll on the surface without slipping
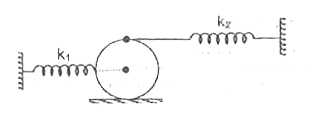 View Solution
View Solution
Knowledge Check
- Question 1 - Select One or More
A disc of mass 3 m and a dise of mass m are connected by a massless sping of stiffness k. The heavier is disc placed on the ground with the spring vertical and lighter disc on top from its equilibrium position the upper disc is pushed down by a distance δ and released. Then.
Aif δ>3mgk the lower desc will bounce up.Bif δ=2mgk maximum normal reaction fron on lower disc = 6mg .Cif δ=2mgk maximum normal reaction from ground on lower disc = 4 mg.Dif δ>4mgk, the lower disc will bounce up - Question 2 - Select One
A disc of mass m is connected with an ideal spring of stiffness k. If it is released from rest, it rolls without sliding on an inclination plane. The maximum elongation of the spring is:
 AmgsinθkB2mgsinθ3kC3mgsinθkD2mgsinθk
AmgsinθkB2mgsinθ3kC3mgsinθkD2mgsinθk - Question 3 - Select One
A uniform circular disc of mass 'm' and radius 'R' is placed on a rough horizontal surface and connected to the two ideal non-deformed spring of stiffness k and 2k at the centre 'C' and point 'A' as shown . The centre of the disc is slighty displaced horizontally from equilibrium positon and then released, then the time period of small oscillation of the disc is (there is no slipping between the disc and the surface).
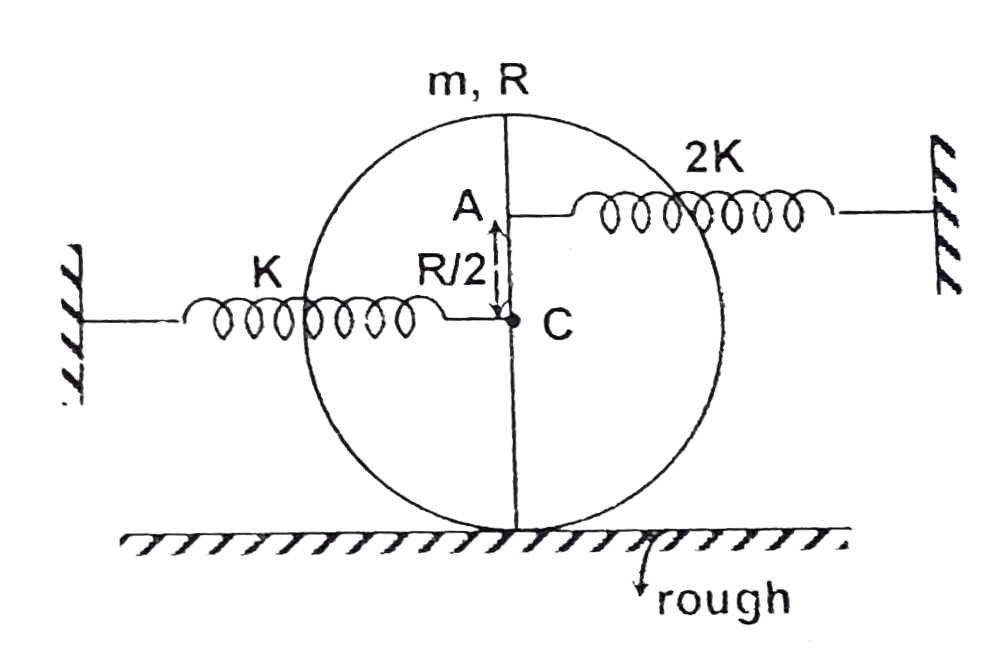 A2π√5m7kB2π√3m7kC2π√5m11kD2π√3m11k
A2π√5m7kB2π√3m7kC2π√5m11kD2π√3m11k
A disc of mass m2 is placed on a table. A stiff spring is attached to it and is vertical. The other end of the spring is attached to a disc of mass m1 What minimum force should be applied to the upper disc to press the spring such that the lower disc is lifted off the table when the external force is suddenly removed?
 View Solution
View SolutionIn the figure shown the spring constant is K the mass of the upper disc is m and that of the lower disc is 3m the upper block is depressed down from its equilibrium position by a distance δ=5mg/k and released at t=0 find the velocity of m when normal reaction on 3m is mg
 View Solution
View SolutionA disc of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between disc and plank to prevent slipping. A force F is applied at the centre of the disc.

Force of friction between the disc and the plank isView SolutionOne end of spring of spring constant k is attached to the centre of a disc of mass m and radius R and the other end of the spring connected to a rigid wall. A string is wrapped on the disc and the end A of the string (as shown in the figure) is pulled through a distance a and then released.
The disc is placed on a horizontal rough surface and there is no slipping at any contact point. What is the amplitude of the oscillation of the centre of the disc?
 View Solution
View SolutionA disc of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between disc and plank to prevent slipping. A force F is applied at the centre of the disc.

Acceleration of the plank isView Solution

