A particle moves along the curve y^(2) = 2x where x = (t^(2))/(2) & y

$v_{x}$ is the velocity of a particle moving along the x-axis as shown in the figure. If $x = 2.0 m$ at $t = 1.0 s,$ what is the position of the particle at $t = 6.0 s$ ?

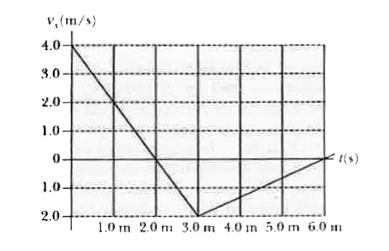A $- 2.0$ m
B $+ 2.0$ m
C $+ 1.0$ m
D $- 1.0$ m
A particle moves along the positive branch of the curve $y = \frac{x^{2}}{2}$ where $x = \frac{t^{2}}{2}, x$ and y are measured in metres and t in second. At $t = 2 s$ , the velocity of the particle is
A $2 ˆ i - 4 ˆ j m s^{- 1}$
B $4 ˆ i + 2 ˆ j m s^{- 1}$
C $2 ˆ i + 4 ˆ j m s^{- 1}$
D $4 ˆ i - 2 ˆ j m s^{- 1}$
A particle moves a distance x in time t according to equation $x^{2} = 1 + t^{2}$ . The acceleration of the particle is
A $\frac{1}{x^{3}}$
B $\frac{1}{x} - \frac{1}{x^{2}}$
C $- \frac{t}{x^{2}}$
D $\frac{1}{x} - \frac{t^{2}}{x^{3}}$

A particle moves along the curve $y^{2} = 2 x$ where $x = \frac{t^{2}}{2}$ & $y > 0$ . What is the acceleration of the particle at $t = 2 s$ ?