Four charges equal to −Q are placed at the four corners of a square and a charge q is at its centre. If the system is in equilibrium the value of q is
−Q2(1+2√2)
Q4(1+2√2)
−Q4(1+2√2)
Q2(1+2√2)
The correct Answer is:B
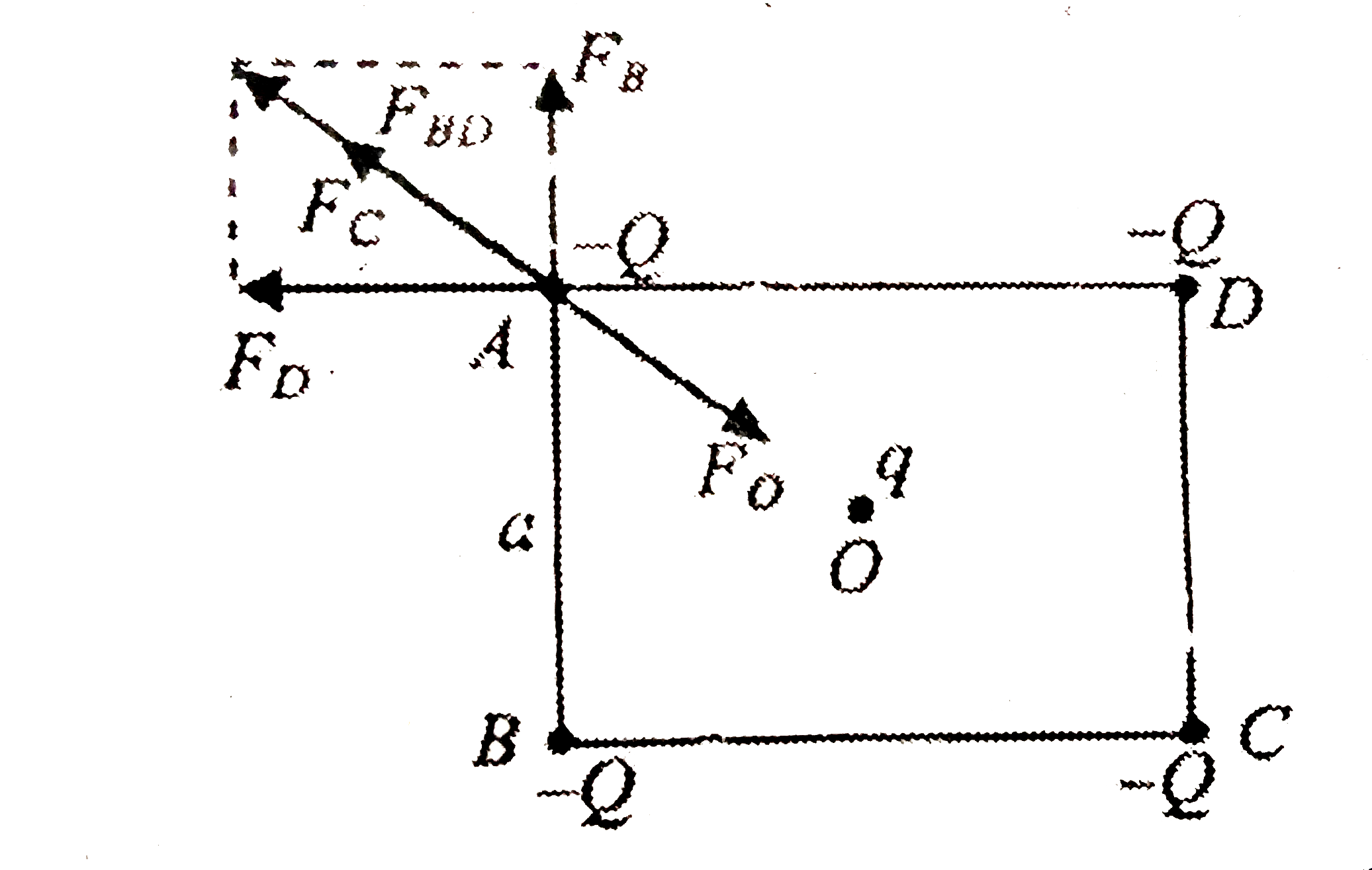
Consider the equilibrium of charge -Q at A.
FB=14πɛ0Q2a2
FD=14πɛ0Q2a2
FBD=√2FB=14πɛ0√2Q2a2
FC=14πɛ0Q2(√2a)2=14πɛ0Q22a2
FO=14πɛ0qQ(√2a2)2=14πɛ02qQa2
For equilibrium, FBD+FC=FO
14πɛ0√2Q2a2+14πɛ0Q22a2=14πɛ02qQa2
or √2Q+Q2=2q
or 2q=Q2(2√2+1)
or q=Q4(1+2√2)
Topper's Solved these Questions
Similar Questions
Four positive point charges Q are placed at the corners of a square of side a. Calculate the charge that should be placed at the centre so that system remains in equilibrium.
View SolutionFour identical charges, Q each, are fixed at the vertices of a square. A free charge q is placed at the centre of the square. Investigate the nature of equilibrium of charge q if it is to be displaced slightly along any of the two diagonals of the square.
View Solution
Knowledge Check
Four charges equal to −Q are placed at the four corners of a square and a charge q is at its centre, If the system is in equilibrium the value of q si
A−Q4(1+2√2)BQ4(1+2√2)C−Q2(1+2√2)DQ2(1+2√2)Four charges equal to Q are placed at the four comers of a square and a charge q is at its centre . If the system is in equilibrium , the value of q is
A−Q4(1+2√2)BQ4(1+2√2)C−Q2(1+2√2)DQ2(1+2√2)Four charges +Q,−Q,+Q,−Q are placed at the corners of a square taken in order. At the centre of the square
AE=0,V=0BE=0,V≠0CE≠0,V=0DE=0,V≠0
Three charges, each of value q, are placed at the vartices of an equilateral triangle. A fourth charge Q is placed at the centre of the triangle. (i) If Q=−q, will the charge at the vertices move towards the centre of fly away from it? (ii) For what value of Q will the charges remain stationary?
View SolutionFour charges Q-Q, Q-Q are placed at the corners of a square as shown . The potential is zero at points
View SolutionA charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium if q is equal to:
View SolutionFour positive charges (2√2−1)Q are arranged at the four corners of a square. Another charge q is placed at the center of the square. Resulting force acting on each corner charge is zero if q is
View SolutionThree charges each of value q are placed at the corners of an equilateral triangle. A fourth charge Q is placed at the centre of the triangle. If Q=−q:
View Solution
