Determine a point which divides a line segment 6 cm long externally in the ratio 3:5.

Text Solution

Verified by Experts

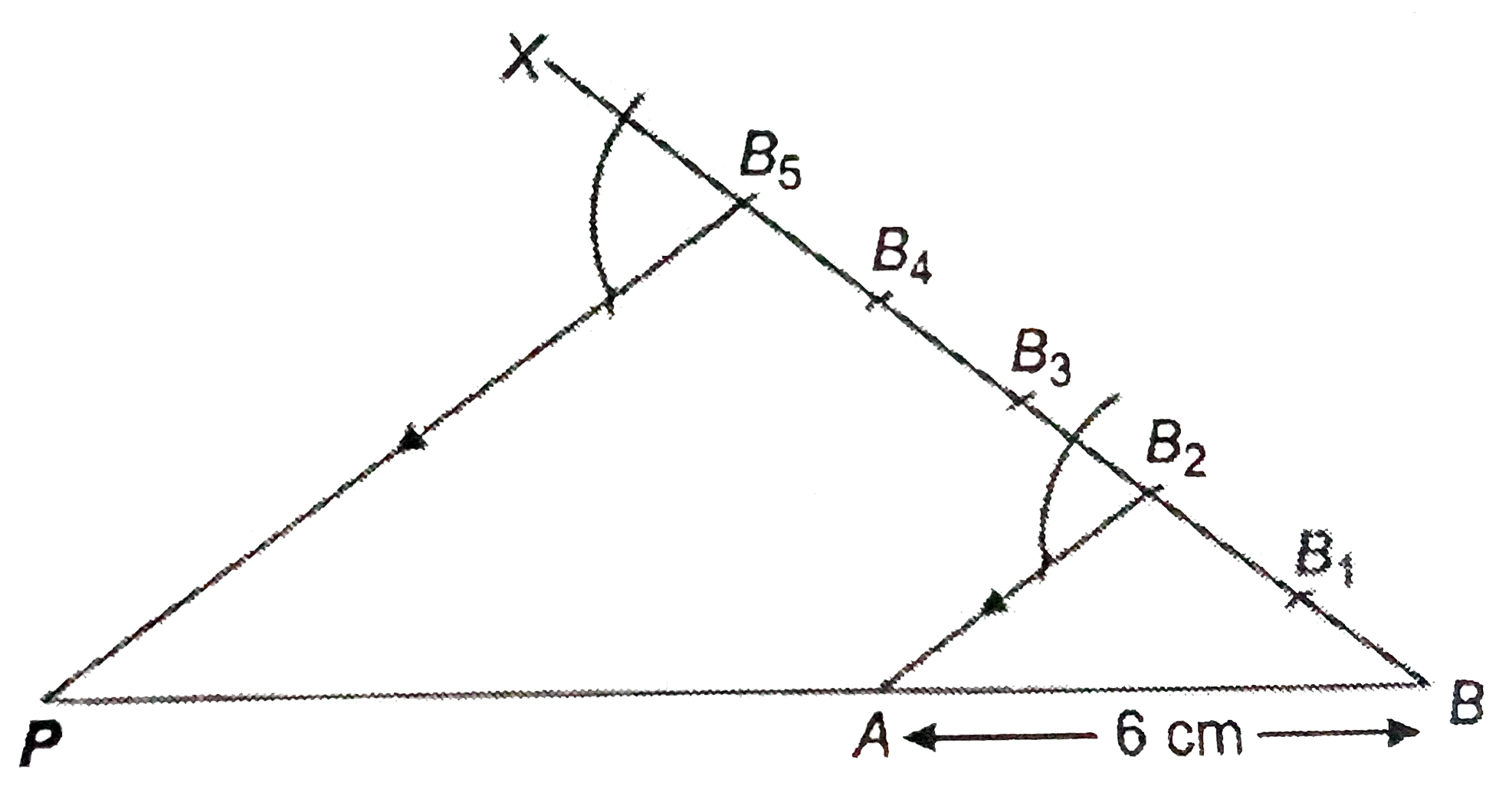
Step of Construction :
1. Draw a line segment AB=6cm.
2. Draw any ray making an acute $∠ A B X$ with AB.
3. Along BX, mark off ( larger among the ratios) 5 points $B_{1}, B_{2}, B_{3}, B_{4} and B_{5}$ such that $B B_{1} = B_{1} B_{2} = B_{2} B_{3} = B_{3} B_{4} = B_{4} B_{5}$ .
4. Join the point $B_{2} (5 - 3)$ with end point A.
5. Draw a line parallel to $B_{2} A$ from $B_{5}$ (larger among the ratios ) which meets BA produced at P.
The point P so obtained is the required point such that the AP:BP=3:5.
Justification: In $Δ P B B_{5}$ ,
Since , $B_{2} A ∣ ∣ B_{5} P$ ( construction)
$∴ \frac{B_{2} B_{5}}{B B_{5}} = \frac{A P}{B P}$ ( by B.P theorem)
$\Rightarrow \frac{3}{5} = \frac{A P}{B P}$ ( construction)
i.e., P divides AB externally in the ratio 3:5.

Updated on:21/07/2023

|

Class 10 MATHS CONSTRUCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Find the coordinate of the point which divides the line segment joining $A (1, - 2, 3) and B (3, 4, - 5)$ in the ratio $2 : 3$ externally.
A $(3, 14, 19)$
B $(- 3, - 14, 19)$
C $(- 3, 14, 19)$
DNone of these

NAGEEN PRAKASHAN-CONSTRUCTIONS-PROBLEMS FROM NCERT/ EXEMPLAR

Home
Ask Doubt
Profile