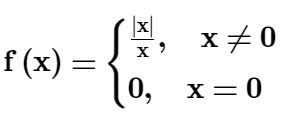If the function f(x) satisfies limx→1f(x)−2x2−1=π, evaluate limX→1f(x).
Video Solution
Text Solution
According to the problem,
limx→1f(x)−2x2−1=π
Now, limX→1[f(x)−2]=limX→1[f(x)−2x2−1.(x2−1)]
=limx→1f(x)−2x2−1.limx→1(x2−1)
=π×0=0
⇒limx→1f(x)=2
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - LIMITS AND DERIVATIVESEXERCISE - EX -13.211videos
LIMITS AND DERIVATIVES
- BOOK - NAGEEN PRAKASHANCHAPTER - LIMITS AND DERIVATIVESEXERCISE - Miscellaneous Exercise30videos
LIMITS AND DERIVATIVES
- BOOK - NAGEEN PRAKASHANCHAPTER - LIMITS AND DERIVATIVESEXERCISE - EX-13H9videos
LIMITS AND DERIVATIVES
- BOOK - NAGEEN PRAKASHANCHAPTER - INTRODUCTION OF THREE DIMENSIONAL GEOMETRYEXERCISE - Miscellaneous Exercise6videos
INTRODUCTION OF THREE DIMENSIONAL GEOMETRY
- BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR INEQUALITIESEXERCISE - MISCELLANEOUS EXERCISE14videos
LINEAR INEQUALITIES
Similar Questions
Knowledge Check
- Question 1 - Select One
If the function f(x) satisfies limx→1f(x)−2x2−1=π, then limx→1f(x) is equal to
A1B2C0D3 - Question 2 - Select One
limx→1xm−1xn−1 is equal to
A1BmnC−mnDm2n2 - Question 3 - Select One
If a differentiable function f(x) satisfies
limx→−1f(x)+1x2−1=32
Then what is limx→−1f(x) equal to ?A−32B−1C0D1