A uniform solid right circular cone of base radius R is joined to a uniform solid hemisphere of radius R and of the same density, so as to have a common face. The centre of mass of the composite solid lies on the common face. The height of the cone is:
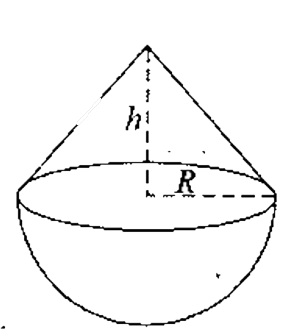
2r
√3r
3 r
√6r
The correct Answer is:B
Step by step video & image solution for A uniform solid right circular cone of base radius R is joined to a uniform solid hemisphere of radius R and of the same density, so as to have a common face. The centre of mass of the composite solid lies on the common face. The height of the cone is: by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A uniform solid right circular cone of base radius R is joined to a uniform solid hemisphere of radius R and of the same density, as shown in the figure. The centre of mass of the.composite solid lies at the centre of base of the cone. The height of the cone is √xR. Find x.
'(##CEN_KSR_PHY_JEE_C08_E01_017_Q10##)'
View SolutionFind the centre of mass of a uniform solid cone.
View Solution
Knowledge Check
A uniform solid right circular cone of base radius R is joined to a uniform solid hemisphere of radius R and of the same density, as shown. The centre of mass of the composite solid lies at the centre of base of the cone. The height of the cone is
 A1.5RB√3RC3RD2√3R
A1.5RB√3RC3RD2√3RA uniform solid sphere of radius R has a cavity of radius 1m cut from it if centre of mass of the system lies at the periphery of the cavity then
 A(R2+R+1)(2–R)=1B(R2–R–1)(2–R)=1C(R2–R+1)(2–R)=1D(R2+R–1)(2–R)=1
A(R2+R+1)(2–R)=1B(R2–R–1)(2–R)=1C(R2–R+1)(2–R)=1D(R2+R–1)(2–R)=1Two solid right cones of equal height and radii r1 and r2 are melted and made to form a solid sphere of radius R. Then the height of the cone is
A4R2r21+r22B4Rr1+r2C4R3r21+r22DR2r21+r22
A right circular cone of radius R and height 2R is placed on a hemisphere of radius R. Locate c.m. of the combined mass from O.
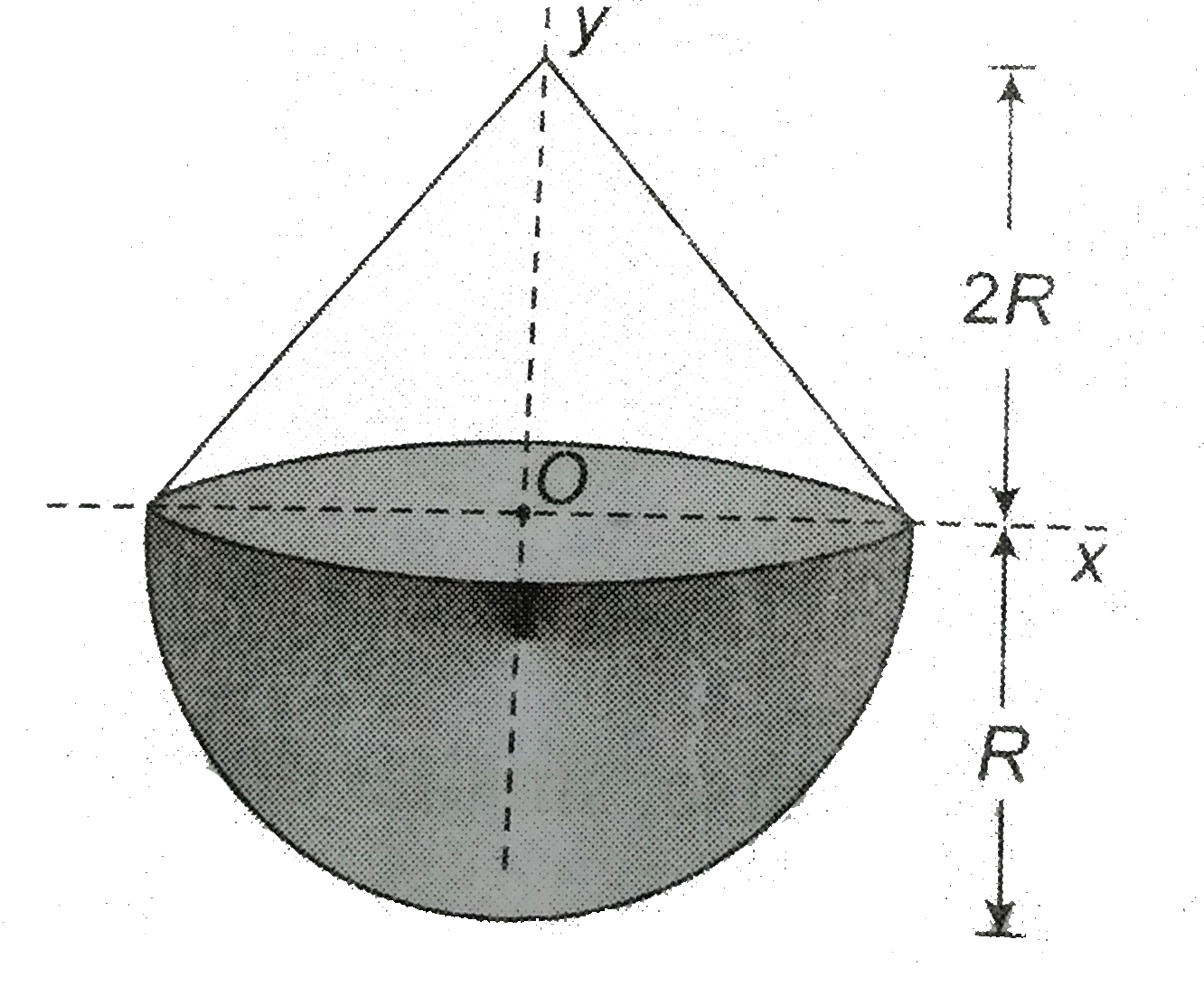
View SolutionDetermine the centre of mass of a uniform hemisphere of radius R.
View SolutionFind the maximum volume of a cone that can be carved out of a solid hemisphere of radius r.
View SolutionTwo solid right cones of equal heights are of radii r1 and r2 are melted and made to form a solid sphere of radius R. Then the height of the cone is:
View SolutionIf two solid hemispheres of same base radius r are joined together along their bases, them curved surface area of the this new solid is
View Solution
