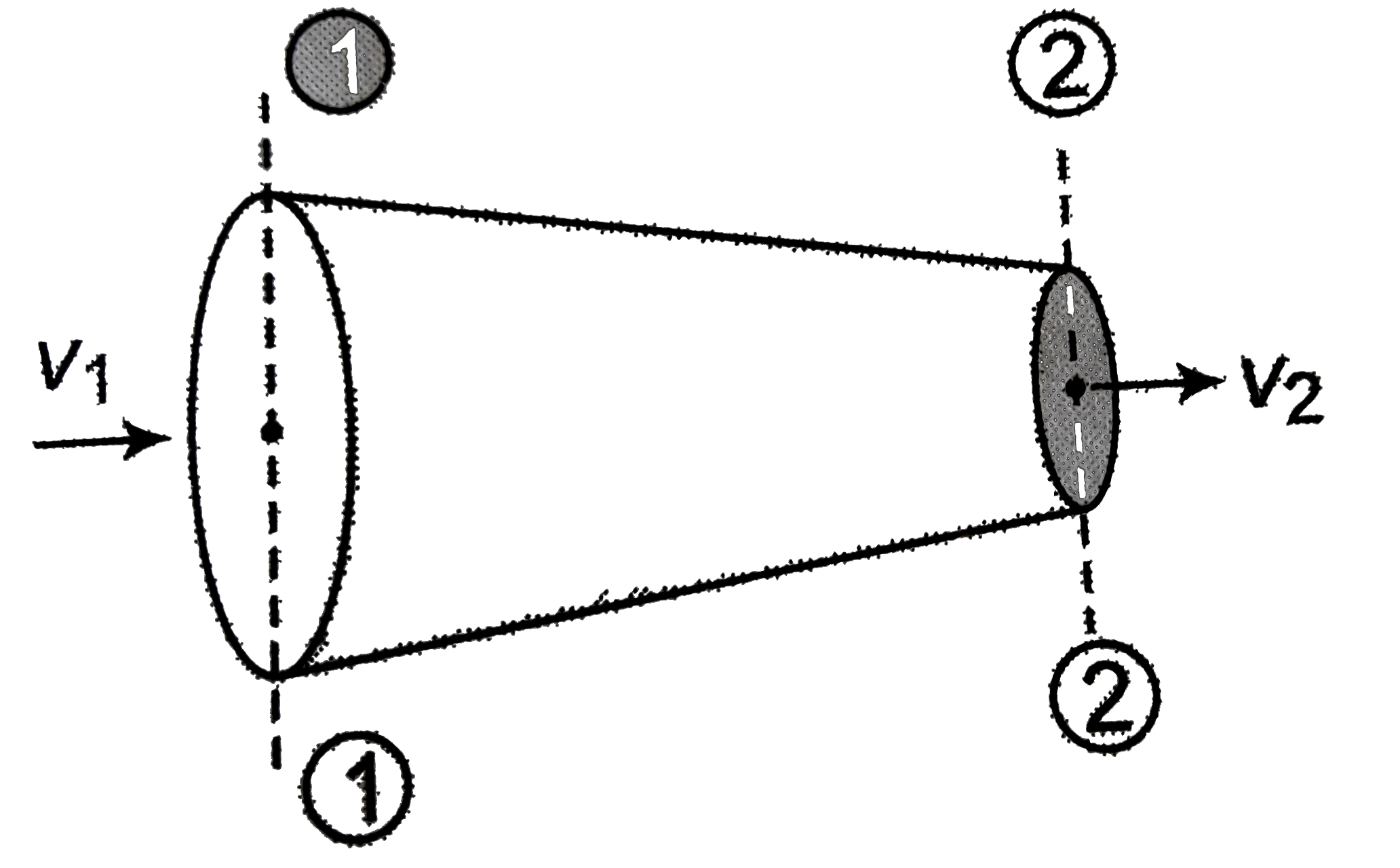
Water flows steadily through a horizontal pipe of a variable cross-section. If the pressure of the water is p at a point , where the speed of the flow is v. What is the pressure at another point , where the speed of the flow is 2 v ? Let the density of water be 1gcm−3.
p+(32)ρv2
p−2ρv2
p−3ρv2
p−(32)ρv2
The correct Answer is:D
To solve the problem, we will use Bernoulli's equation, which relates the pressure, velocity, and height of a fluid flowing in a horizontal pipe. The equation is given by:
P1+12ρv21+ρgh1=P2+12ρv22+ρgh2
Step 1: Identify the known values
- At point 1:
- Pressure P1=P
- Velocity v1=v
- At point 2:
- Pressure P2=?
- Velocity v2=2v
- Density of water ρ=1g/cm3 (which is equivalent to 1000kg/m3 in SI units)
- Since the pipe is horizontal, the heights h1 and h2 are equal, so h1=h2.
Step 2: Simplify Bernoulli's equation
Since h1=h2, the terms involving height cancel out:
P+12ρv2=P2+12ρ(2v)2
Step 3: Substitute the known values
Substituting v2=2v:
P+12ρv2=P2+12ρ(4v2)
Step 4: Rearrange the equation to solve for P2
Rearranging gives:
P2=P+12ρv2−12ρ(4v2)
This simplifies to:
P2=P+12ρv2−2ρv2
P2=P−32ρv2
Step 5: Substitute the density value
Now substituting ρ=1g/cm3=1000kg/m3:
P2=P−32(1)v2
Final Result
Thus, the pressure at the second point where the speed of the flow is 2v is:
P2=P−32v2
- At point 1:
- Pressure P1=P
- Velocity v1=v
- At point 2:
- Pressure P2=?
- Velocity v2=2v
- Since the pipe is horizontal, the heights h1 and h2 are equal, so h1=h2.
Since h1=h2, the terms involving height cancel out:
Substituting v2=2v:
Rearranging gives:
Now substituting ρ=1g/cm3=1000kg/m3:
Thus, the pressure at the second point where the speed of the flow is 2v is:
Topper's Solved these Questions
Similar Questions
Water is flowing through a horizontal pipe of varying cross-section. If the pressure of water equals 2 cm of Hg where velocity of flow is 32cms−1, what is the pressure at another point where the velocity of flow is 40cms−1?
View SolutionIt water flows horizontally through a pipe of varying cross section and the pressure of water equals 10cm of g at a point where the velocity of flow is 40cm/s, what is the pressure at another point where the velocity of flow is 50cm/s?
View Solution
Knowledge Check
Water flows steadily through a horizontal pipe of a variable cross-section. If the pressure of water is p at a point where the velocity of flow is v, what is the pressure at another point where the velocity of flow is 2v, ρ being the density of water?
Ap+2ρv2Bp−2ρv2Cp+32ρv2Dp−32ρv2Water flows steadily through a horizontal pipe of variable cross-section. If the pressure of water is p at a point where the velocity of flow is v, at another point (pressure p'), where the velocity of flow in ηv : The following statements are given below
Ap'p<1, when η<1Bp'p<1, when η>1Cp'p>1, when η<1Dp'p>1, when η>1A liquid flows through a horizontal tube of variable diameter. Then the pressure is lowest where
Avelocity is lowestBvelocity is highestCdiameter is largestDboth velocity and diameter are largest
Water is flowing steadily through a horizontal pipe of non-uniform cross-section. If the pressure of water is 4×104N/m2 at a point where cross-section is 0.02m2 and velocity of flow is 2m/s. What is the pressure at a point where cross-section reduces to 0.01m2 ?
 .
.
View SolutionWater flows through a horizontal pipe of varying cross-section at the rate of 20 litres per minuts , determine the velocity of water at a point where diameter is 4cm
View SolutionWater flowing steadily through a horizontal pipe of non-unifrom cross-section. If the pressure of water is 4×104N/m2 at a point where cross-section is 0.02m2 and velocity of flow is 2ms−1 . The pressure at a point where cross-section reduces to 0.01m2 is 3.4×10n Pa. What is the value of n ?
View SolutionWater is flowing steadily through a horizontal pipe of non - uniform cross - section . If the velocity of water cross - section is 0.02m2 is 2m/s , what is the velocity of water at another point where the cross - section is 0.01m2 ?
View SolutionWater is flowing steadily through a horizontal pipe of non-uniform cross-section. If the pressure of water is 4×104N/m2 at a point where cross-section is 0.02m2 and velocity of flow is 2m/s. What is the pressure at a point where cross-section reduces to 0.01m2 ?
 .
.
View Solution
