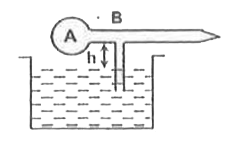
The figure shows a model of perfume atomizer . When the bulb A is compressed , air (density ρa) flows through the vertical tube and emerges through the end . If excess pressure applied to the bulb ins progress be Δpthen the minimu speed of air in the tube to lift perfume is :
√2[Δp+ogh]ρa
√2[Δp−ρgh]ρa
√Δρ+ρghρa
None of these
Applying Bernoull.s principle at 1 and 2 , we obtain
P1+12ρav21=P2+12ρav22
Putting v1=0 since of the air is at rest inside the bulb ,
P1=P10+ΔPrP2=P0−ρgh whre ρ= density of liquid ( perfume)
ρa density of air
va = minimum velocity of air to lift the liquid through the height h = v (say)
P0 = atmospheric pressure
Hence ( A) is correct
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - FLUID MECHANICS EXERCISE - LINKED COMPREHENSION TYPE2videos
FLUID MECHANICS
- BOOK - FIITJEECHAPTER - FLUID MECHANICS EXERCISE - LINKED COMPREHENSION TYPE I1videos
FLUID MECHANICS
- BOOK - FIITJEECHAPTER - FLUID MECHANICS EXERCISE - SOLVED PROBLEMS (SUBJECTIVE)10videos
FLUID MECHANICS
- BOOK - FIITJEECHAPTER - ELECTROSTATICSEXERCISE - Example14videos
ELECTROSTATICS
- BOOK - FIITJEECHAPTER - GENERAL PHYSICSEXERCISE - MATRIX MATCH TYPE3videos
GENERAL PHYSICS
Similar Questions
The figure shows a crude type of perfume atomizer. When bulb at A is compressed, air flows swifty through the tiny BC with uniform speed v, there by causing a reduced pressure at the position of verticall tube DE. The liquid of density 500kg/m3, then rises in the tube, enters tube BC and sprayed out. When bulb is in natural position the air in the bulb and tube are at atmospheric pressure P50=15N/m2. When bulb A is compressed, it creates an exces pressure Δp=0.001P0 inside the bulb A. Density of air is 1.3kg/m3. If the magnitude of minimum value of speed v required to cause the liquid to rise to tube BC is 5km/s. Find the value of k. (g=10m/s2)
 View Solution
View SolutionThe figure shows a crude type of perfume atomizer. When bulb at A is compressed, air flows swifty through the tiny BC with uniform speed v, there by causing a reduced pressure at the position of verticall tube DE. The liquid of density 500kg/m3, then rises in the tube, enters tube BC and sprayed out. When bulb is in natural position the air in the bulb and tube are at atmospheric pressure P50=15N/m2. When bulb A is compressed, it creates an exces pressure Δp=0.001P0 inside the bulb A. Density of air is 1.3kg/m3. If the magnitude of minimum value of speed v required to cause the liquid to rise to tube BC is 5km/s. Find the value of k. (g=10m/s2)
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Water is flowing through a tube of radius r with a speed v. If this tube is joined to another tube of radius r/2, what is the speed of water in the second tube?
Av/4Bv/2C2vD4v - Question 2 - Select One
Figure shows a crude type of atomizer. is compressed, air flows swiftly through tube BC causing a reduced pressure in the particles of the vertical to Liquid rises in the tube, enters BC and is sprayed out. if the pressure in the bulb is Pa+P, where P is the gauge pressure and Pa is the atmospheric pressure, v is the speed of air in BC, find how large would v need to be to cause the liquid to rise to BC. Density of air =1.3kg/m3.
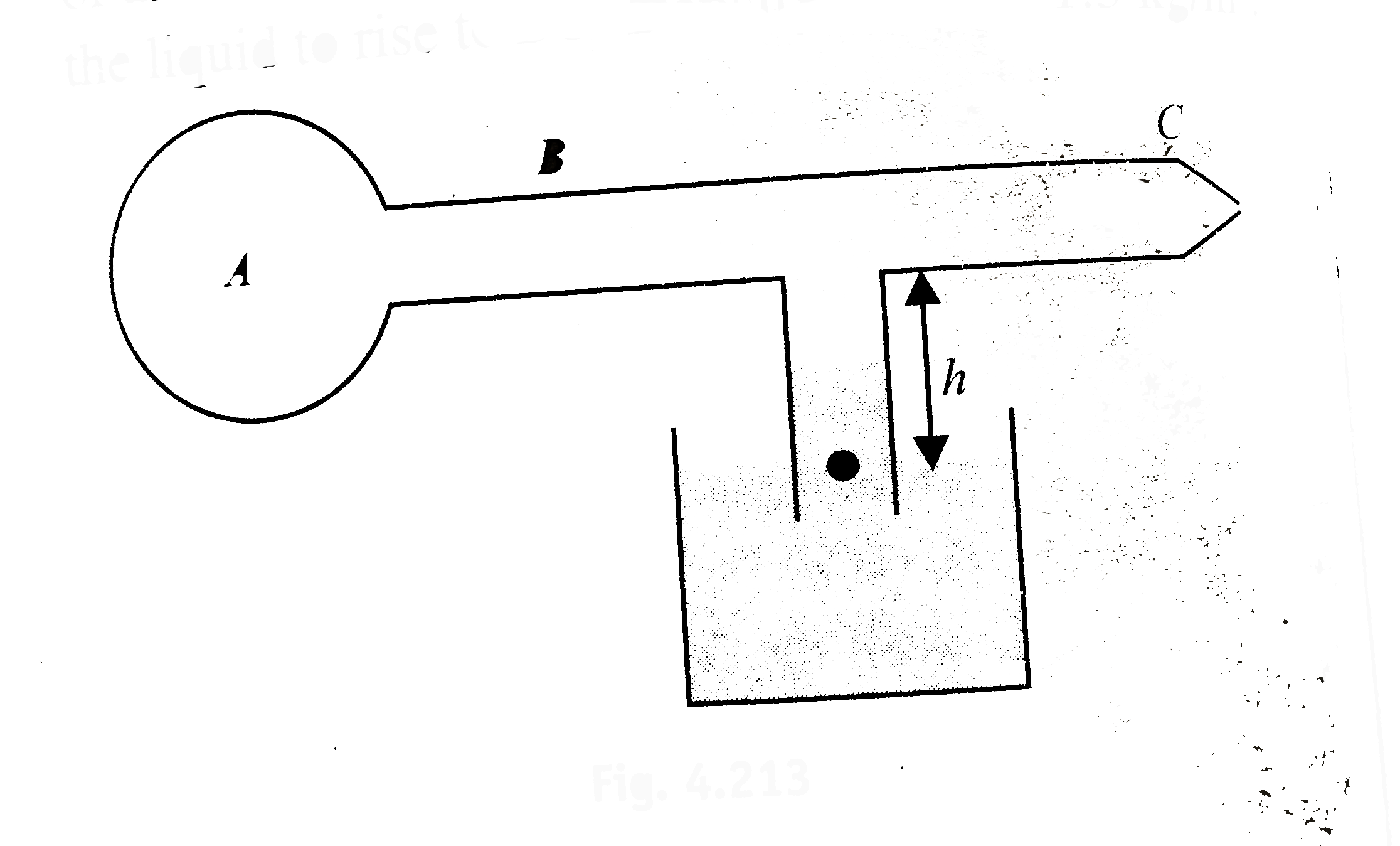 AP+ρgh√0.65BPhρg√0.650CP√hρg0.65DPhρg√0.65
AP+ρgh√0.65BPhρg√0.650CP√hρg0.65DPhρg√0.65
Water is flowing through a tube of radius r with a speed v. If this tube is joined to another tube of radius r/2, what is the speed of water in the second tube?
View SolutionWater is flowing through a long horizontal tube. Let PAandPB be the pressures at two points A and B of the tube
View SolutionWater is flowing through a long horizontal tube. Let PAandPB be the pressures at two points A and B of the tube
View SolutionWhen bulbs are connected in series, same current flows through them.
View SolutionWhen bulbs are connected in series, same current flows through them.
View SolutionFigure shows a crude type of atomizer. is compressed, air flows swiftly through tube BC causing a reduced pressure in the particles of the vertical to Liquid rises in the tube, enters BC and is sprayed out. if the pressure in the bulb is Pa+P, where P is the gauge pressure and Pa is the atmospheric pressure, v is the speed of air in BC, find how large would v need to be to cause the liquid to rise to BC. Density of air =1.3kg/m3.
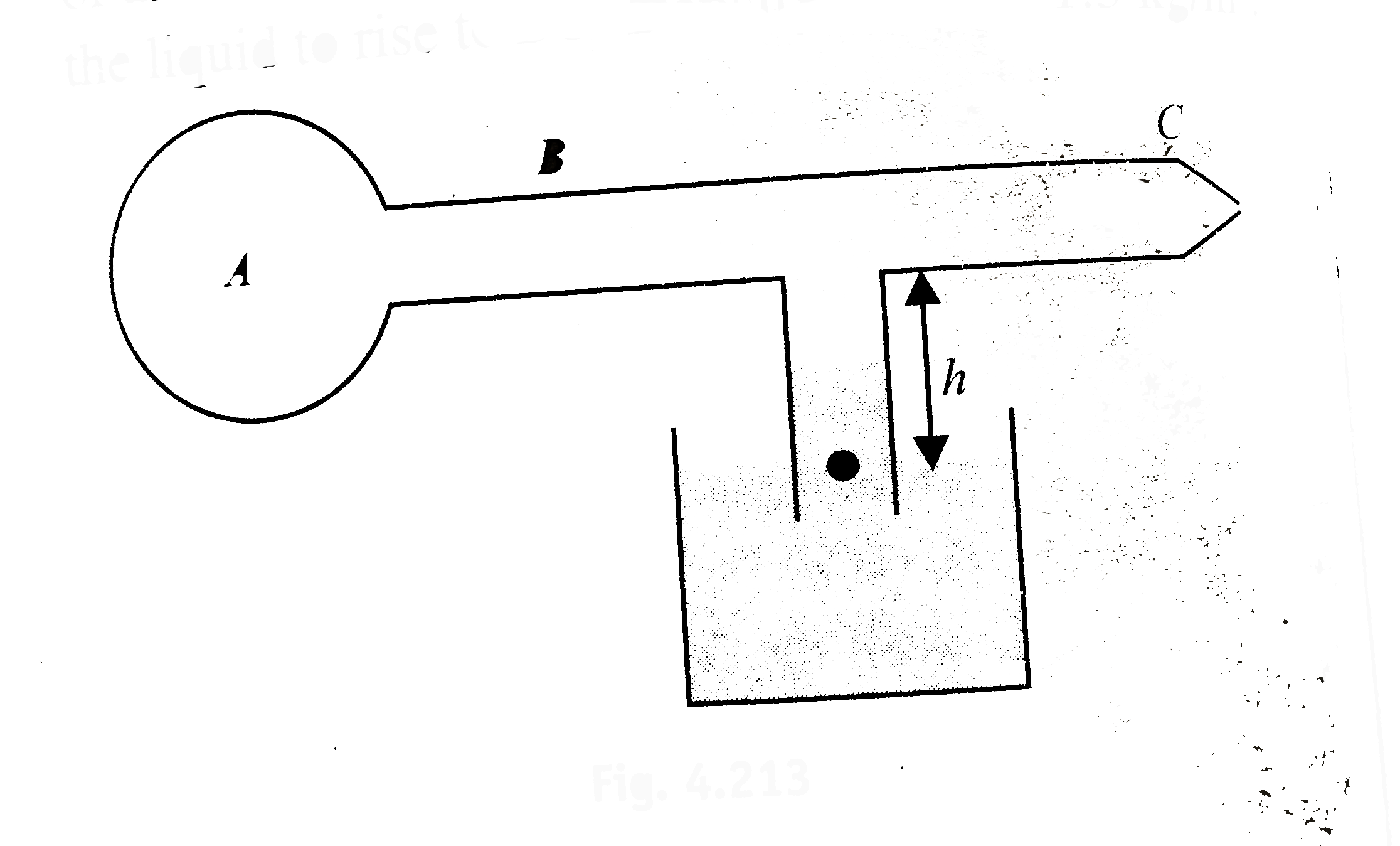 View Solution
View Solution

