Write the equation of SHM for the situtions shown below:
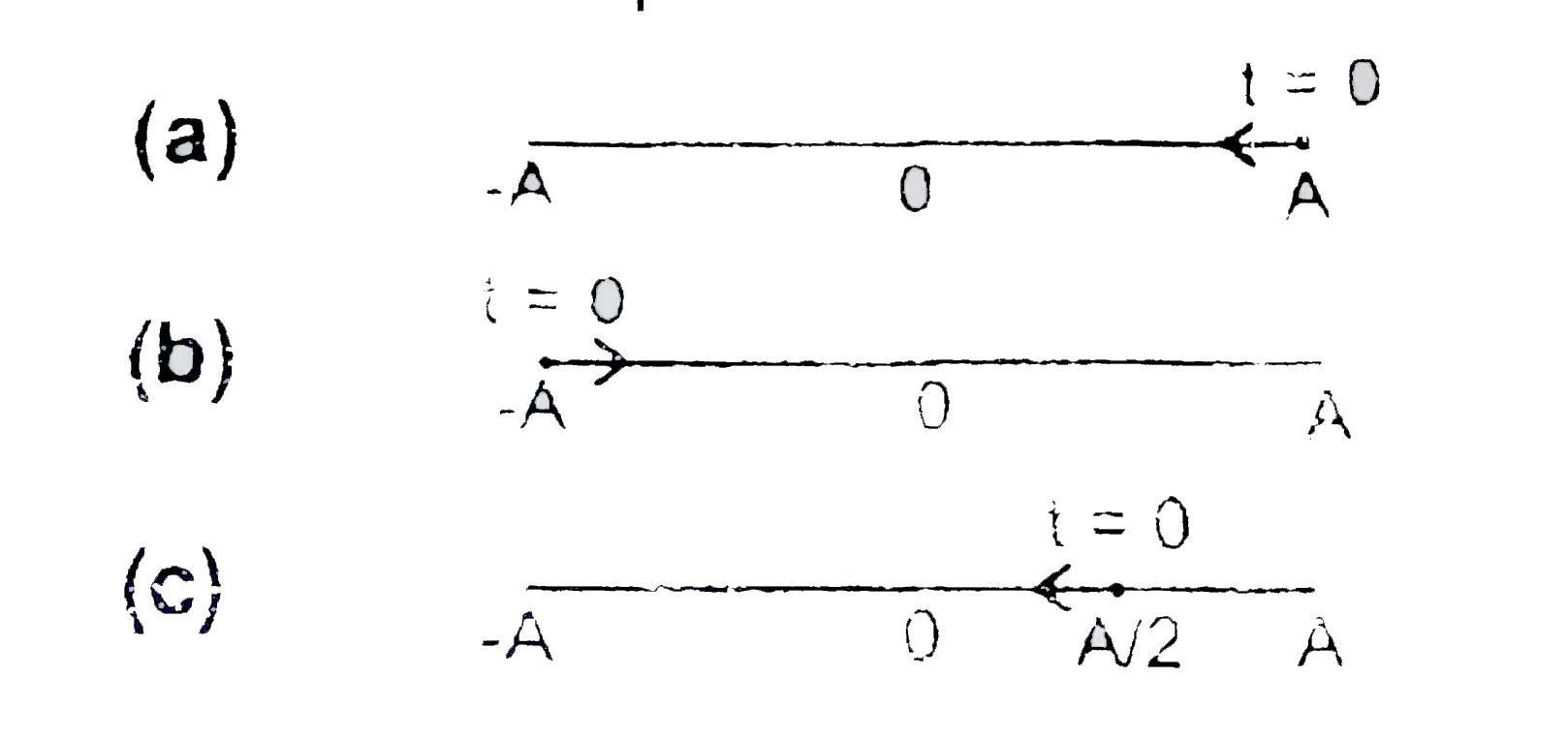
Text Solution
(a) At t=0,x=+A
x=Asin(ωt+ϕ)
A=Asin(ϕ)
ϕ=π/2
x=Asin(ωt+π2)=Acos(ωt)
(b) At t=0,x=−A
x=Asin(ωt+ϕ)
−A=Asinϕ
ϕ=3π2
x=Asin(ωt+3π2)
x=−Acos(ωt)
(c) At t=0,x=A2
x=Asin(ωt+ϕ)
A2=Asin(ωt+ϕ)
12=sinϕ⇒ϕ=30∘,150
Particle is moving towards the mean position and in negative direction.
velocity v=Aωcos(ωt+ϕ)
At t=0,v=−ve
hence v=Aωcisϕ
hence ϕ=150∘
x=Asin(ωt+150∘)
(a) x=Acosoemgat, (b) x=−Acosωt, (c) x=Asin(ωt+150∘)
|
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Board Level Exercise
24 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - I
25 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- SEMICONDUCTORSBOOK - RESONANCECHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - FST-3
30 Videos
Similar Questions
Write the equation of SHM for the sitution shown below :
_S01_004_Q01.png)
View SolutionWrite the equation of SHM for the sitution shown below :
_S01_004_Q01.png)
View SolutionWrite the equation of SHM for the situation shown below :
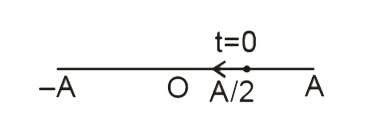
View SolutionWrite the equation of SHM for the situations show below:

View SolutionWrite the equation of SHM for the situations show below:

View SolutionWrite the equation of SHM for the situations show below:

View SolutionWrite equations for the straight lines shown in the following graphs.
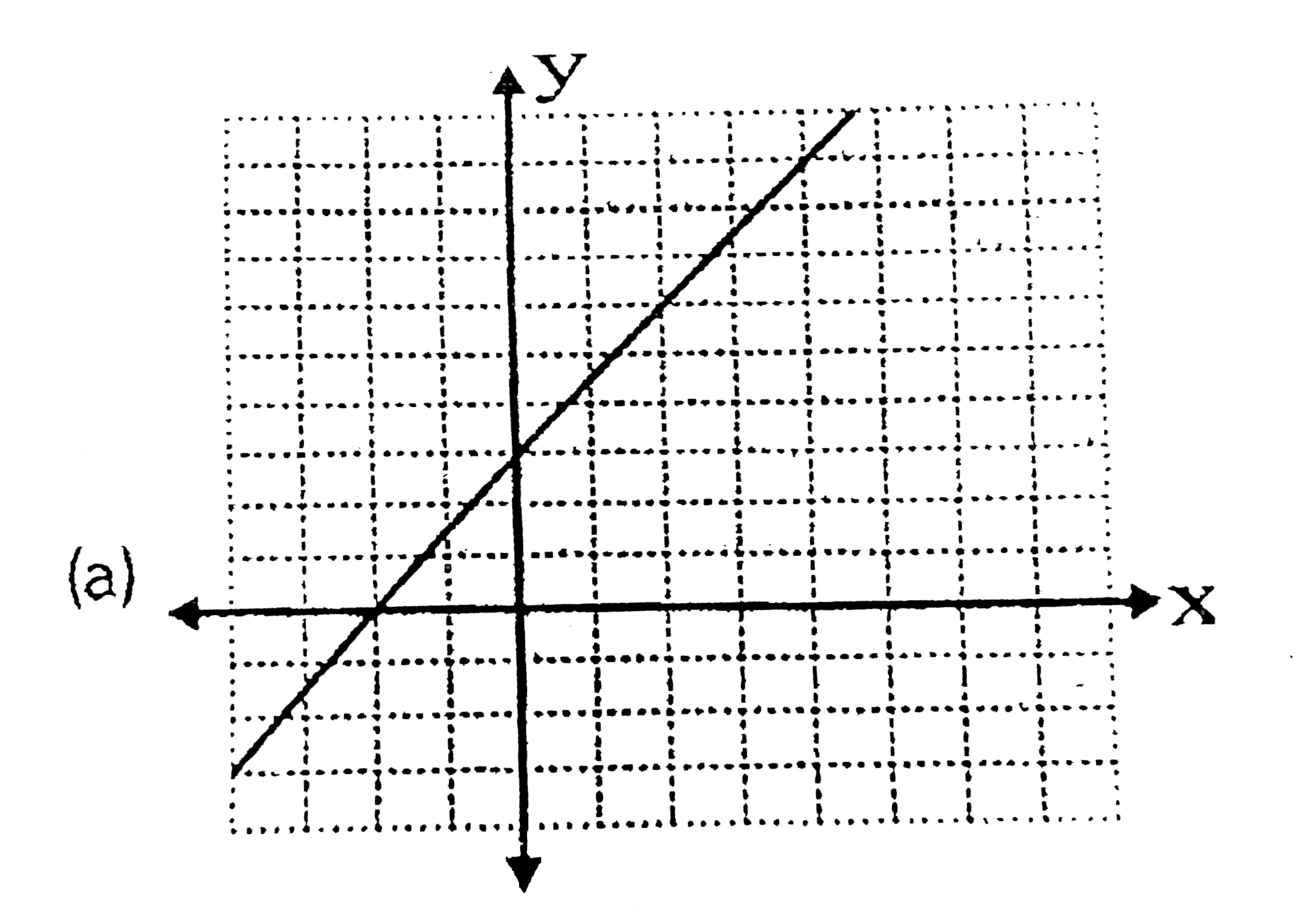
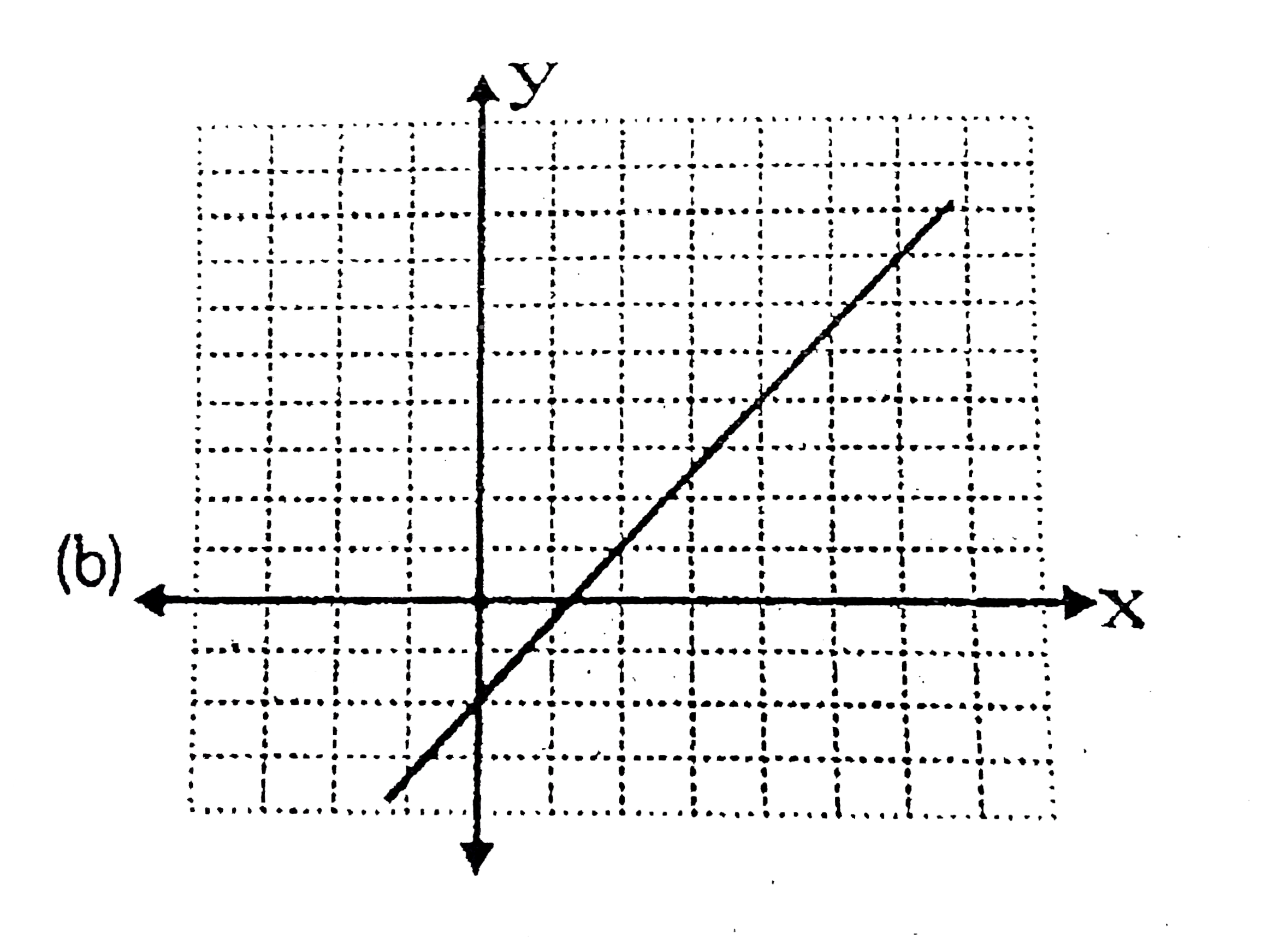


View SolutionWrite equations for the straight lines shown in the following graphs.




View SolutionWrite the equation of an S.H.M of amplitude 5cm if 150 oscillations are performed in one minute and the intial phase is 45∘
View SolutionShm - Equation Of Motion
View Solution
