A convex mirror and a concave mirror each of focal length 10cm are placed coaxially. The are separated by 40cm and their reflecting surfaces face each other. A point object is kept on the principle axis at a distance xcm from the concave mirror such that final image after two reflections, first on the concave mirror, is on the object itself. Find integer next to x .
The correct Answer is:13
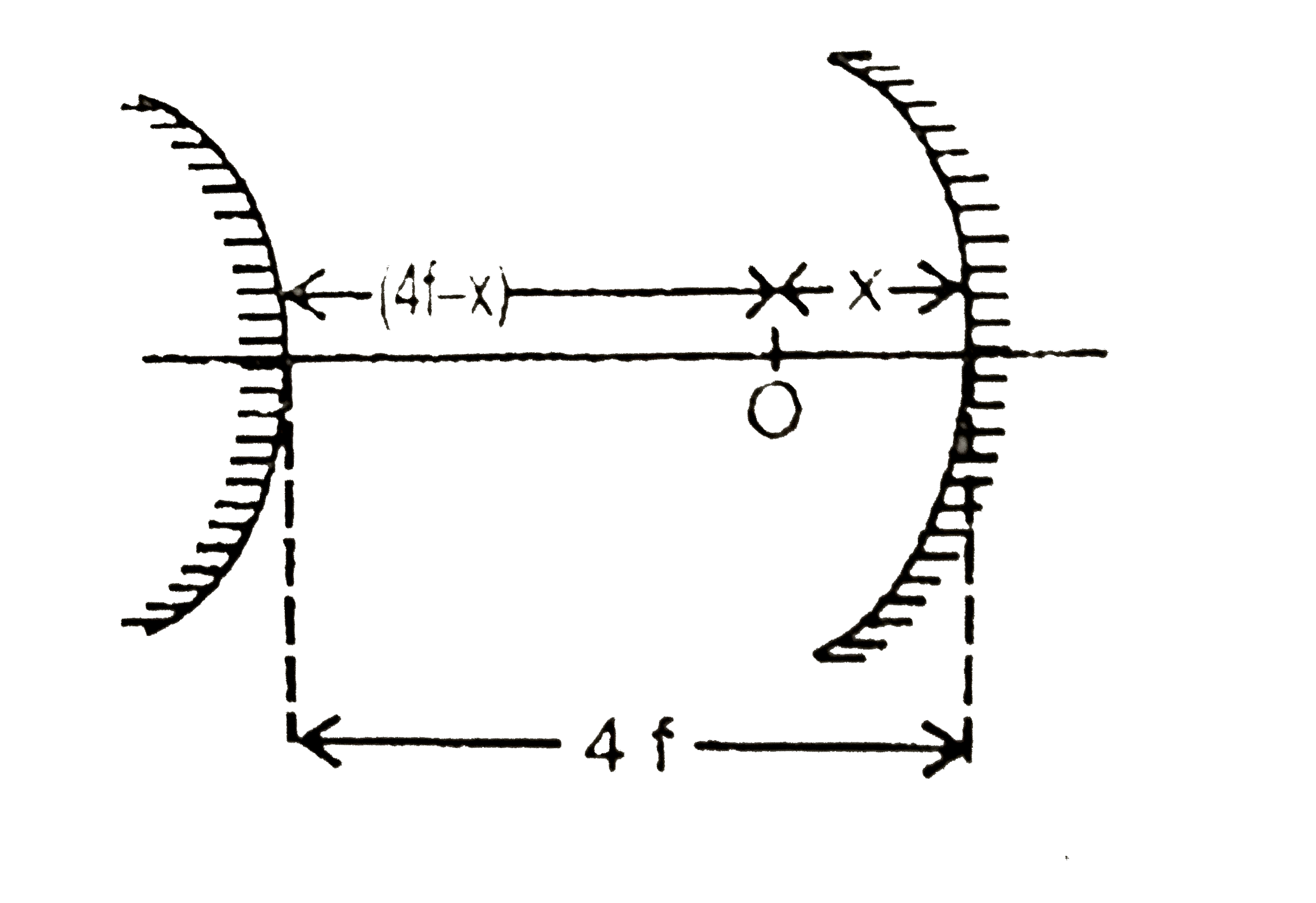
Let the situation be as shown in figure with the object and iamge shown at A . For image farmation by concave mirror,
u=−x
f=−f
1v=1f−1u=−1f+1x⇒v=fxf−x
∴ Distance of the image from the convex mirror =4f+fxf−x=4f2−3fx(f−x)
u=−(4f2−3fx)(f−x)
f=f
By the question,
v=−(4f−x) ∴ 1v=1f−1ugives ,
1−(4f−x)=1f+(f−x)(4f2−3fx)
on solving, we get,
x2−6fx+6f2=0
which gives, x=(3±√3)f But x<4f
Hence, x=(3−√3)10cm=12.68cm
Topper's Solved these Questions
- GEOMATRICAL OPTICS BOOK - RESONANCECHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-3
79 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCECHAPTER - GEOMATRICAL OPTICS EXERCISE - Advance level Problems
35 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCECHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-1
142 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCECHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
- GRAVITATIONBOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - HIGH LEVEL PROBLEMS
16 Videos
Similar Questions
A concave mirror of focal length 10cm and a convex mirror of focal length 15cm are placed facing each other 40cm apart. A point object is placed between the mirrors, on their common axis and 15cm from the concave mirror. Find the position and nature of the image produced by successive reflections, first at the concave mirror and then at the convex mirror.
View SolutionAn object of height 2 cm is placed on a principal axis at a distance of 10 cm from the pole of concave mirror. The image height is 5 cm. Find the image distance and the focal length of the concave mirror if the image is real and inverted.
View Solution
Knowledge Check
A concave mirror of focal length 10cm and a convex mirror of focal length 15cm are placed facing each other 40cm apart. A point object is placed between the mirrors, on their common axis and 15cm from the concave mirror. Find the position and nature of the image produced by successive reflections, first at the concave mirror and then at the convex mirror.
A12cm behind convex mirror, realB9cm behind convex mirror, realC6cm behind convex mirror, virtualD3cm behind convex mirror, virtualAn object is present on the principal axis of a concave mirror at a distance 30cmfrom it.Focal length of mirror is20cm
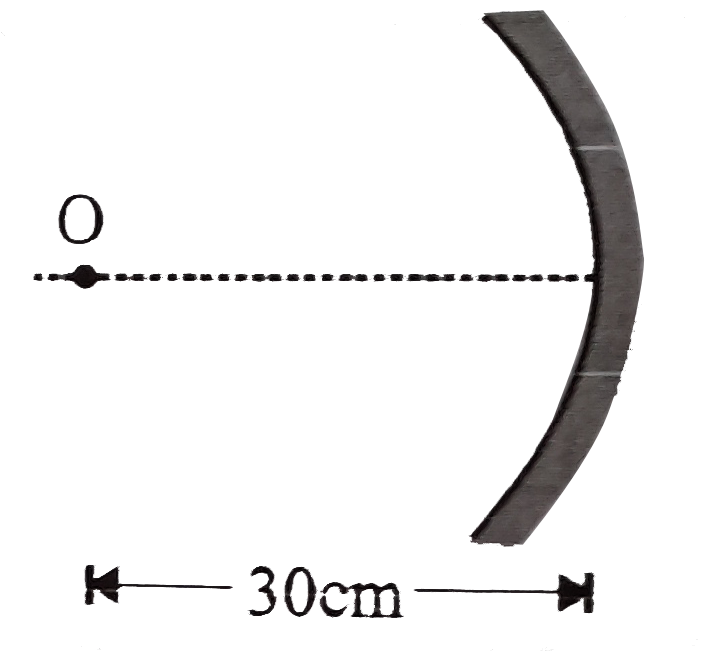
Image formed by mirror isAAt a distance 60cmin front of mirror.BAt a distance 60cmbehind of mirror.CAt a distance 12cmin front of mirror.DAt a distance 12cmbehind of mirror.An object is placed at a distance of 15 cm from a concave mirror and its real image is formed at 30 cm in front of that mirror. The focal length of the mirror is
A+20 cmB−20 cmC−10 cmD+10 cm
An object is placed at a distance of 30 cm from a concave mirror and its real image is formed at a distance of 30 cm from the mirror. The focal length of the mirror is
View SolutionAn object is present on the principal axis of a concave mirror at a distance 30cm from it. Focal length of mirror is 20cm.
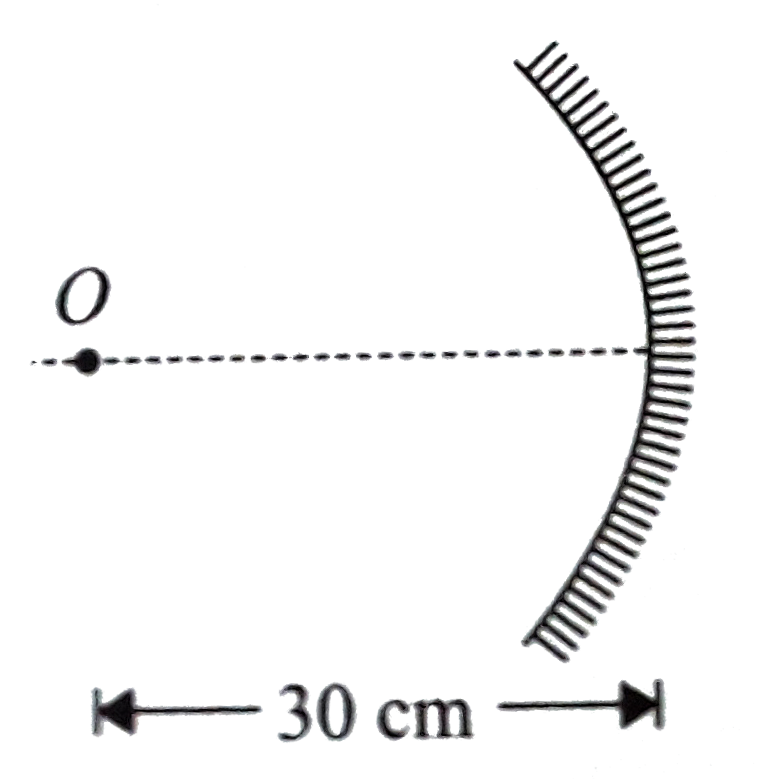
Q. Image formed by mirror is
View SolutionAn object is placed at a distance of 30 cm from a concave mirror and its real image is formed at a distance of 30 cm from the mirror. The focal length of the mirror is
View SolutionAn object is present on the principal axis of a concave mirror at a distance 30cm from it. Focal length of mirror is 20cm.

Q. Image formed by mirror is
View SolutionTwo mirrors, one concave and the other convex, are placed 60 cm apart with their reflecting surfaces facing each other. An object is placed 30 cm from the pole of either of them on their axis. If the focal lengths of both the mirrors are 15 cm, the position of the image formed by reflection, first at the convex and then at the concave mirror, is:
View Solution
