A conducting slab of thickness ‘t’ is introduced between the plates of a parallel plate capacitor, separated by a distance d (t<d). Derive an expression for the capacitance of the capacitor. What will be its capacitance when t = d ?
To derive the expression for the capacitance of a parallel plate capacitor with a conducting slab of thickness t introduced between the plates, we can follow these steps:
Step 1: Understand the Configuration
We have a parallel plate capacitor with a distance d between the plates. A conducting slab of thickness t is inserted between the plates. The remaining distance between the slab and the plates will be d−t.
Step 2: Identify the Electric Fields
- The electric field in the air gap (distance d−t) can be denoted as E0.
- The electric field in the conducting slab is zero because it is a conductor.
Step 3: Calculate the Potential Difference
The total potential difference V across the capacitor can be calculated as the sum of the potential differences across the two regions:
1. Potential difference across the air gap:
Vair=E0⋅(d−t)
where E0=Qϵ0A.
2. Potential difference across the conducting slab is zero:
Vslab=0
Thus, the total potential difference is:
V=Vair+Vslab=E0(d−t)+0=E0(d−t)
Step 4: Substitute the Electric Field
Substituting E0:
V=Qϵ0A(d−t)
Step 5: Calculate the Capacitance
The capacitance C is defined as:
C=QV
Substituting the expression for V:
C=QQϵ0A(d−t)=ϵ0Ad−t
Step 6: Final Expression for Capacitance
Thus, the capacitance of the capacitor with the conducting slab inserted is:
C=ϵ0Ad−t
Step 7: Case When t=d
When t=d, the conducting slab fills the entire space between the plates. In this case, there is no air gap left, and the potential difference across the capacitor becomes zero. Therefore, the capacitance becomes:
C=Q0→undefined (infinite capacitance)
This indicates that the capacitor behaves like a short circuit when the slab completely fills the space.
Summary of Results
1. For t<d:
C=ϵ0Ad−t
2. For t=d:
C→undefined (infinite capacitance)
We have a parallel plate capacitor with a distance d between the plates. A conducting slab of thickness t is inserted between the plates. The remaining distance between the slab and the plates will be d−t.
- The electric field in the air gap (distance d−t) can be denoted as E0.
- The electric field in the conducting slab is zero because it is a conductor.
The total potential difference V across the capacitor can be calculated as the sum of the potential differences across the two regions:
1. Potential difference across the air gap:
Vair=E0⋅(d−t)
where E0=Qϵ0A.
Vslab=0
V=Vair+Vslab=E0(d−t)+0=E0(d−t)
Substituting E0:
V=Qϵ0A(d−t)
The capacitance C is defined as:
C=QV
Substituting the expression for V:
C=QQϵ0A(d−t)=ϵ0Ad−t
Thus, the capacitance of the capacitor with the conducting slab inserted is:
C=ϵ0Ad−t
When t=d, the conducting slab fills the entire space between the plates. In this case, there is no air gap left, and the potential difference across the capacitor becomes zero. Therefore, the capacitance becomes:
C=Q0→undefined (infinite capacitance)
This indicates that the capacitor behaves like a short circuit when the slab completely fills the space.
1. For t<d:
C=ϵ0Ad−t
2. For t=d:
C→undefined (infinite capacitance)
Topper's Solved these Questions
- ELECTROSTATICS AND CURRENT ELECTRICITY BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROSTATICS AND CURRENT ELECTRICITY EXERCISE - LONG ANSWER QUESTIONS
6 Videos
- ELECTROSTATICS AND CURRENT ELECTRICITY BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROSTATICS AND CURRENT ELECTRICITY EXERCISE - NUMERICALS
49 Videos
- ELECTROSTATICS AND CURRENT ELECTRICITY BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROSTATICS AND CURRENT ELECTRICITY EXERCISE - SHORT ANSWER QUESTIONS (2 MARKS)
59 Videos
- ELECTRONIC DEVICES BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTRONIC DEVICES EXERCISE - NUMERICALS
7 Videos
- SAMPLE QUESTION PAPER 2019BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - SAMPLE QUESTION PAPER 2019EXERCISE - Section C
19 Videos
Similar Questions
A conducting slab of thickness 't' is introduced without touching between the plates of a parallel plate capacitor , separated by a distance 'd' (t<d) . Derive an expression for the capacitance of the capacitor .
View SolutionA dielectric slab of thickness 't' is kept between the plates of a parallel plate capacitor separated by a distance 'd' (t<d) . Derive the expression for the capacity of the capacitor .
View Solution
Knowledge Check
A dielectric slab is placed between the plates of a parallel plate capacitor. Its capacitance
Abecomes zeroBremains the sameCdecreasesDincreasesWhen a conducting slab fills the space between the two plates of a capacitor, its capacitance
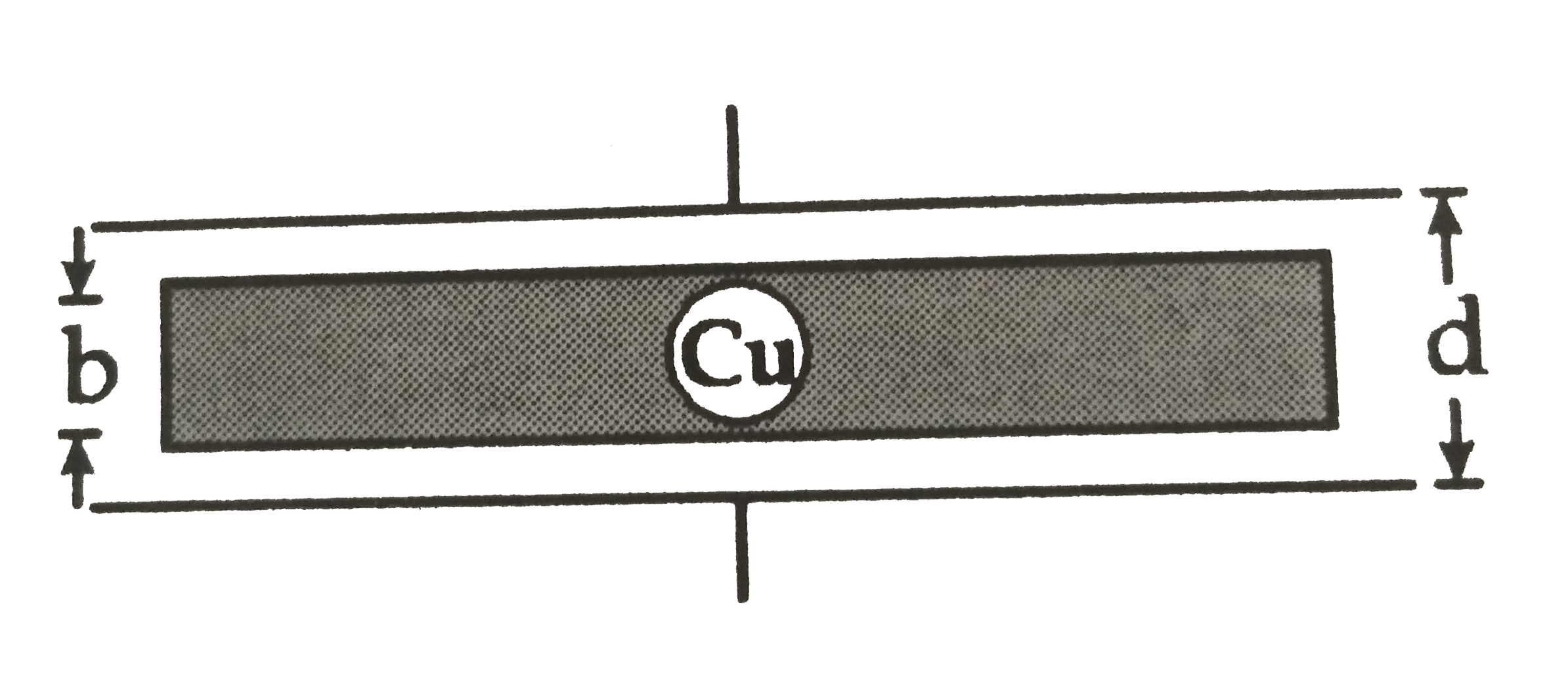
Abecomes infiniteBbecomes four times the original one.Cremains same.Dbecomes zeroA copper plate of thickness b is placed inside a parallel plate capacitor of plate distance d and area A as shown in figure. The capacitance of capacitor is
 AAε0dBAε0bCAε0d−bD∞
AAε0dBAε0bCAε0d−bD∞
Derive an expression for the capacitance of a parallel-plate capacitor filled with a dielectric.
View SolutionIf a dielectric slab of thickness t and area A is inserted in between the plates of a parallel plate capacitor of plate area A and distance between the plates d(d>t) then find out capacitance of system. What do you predict about the dependence of capacitance on location of slab?
View SolutionWhat is a parallel plate capacitor? Obtain an expression for the capacitance of a parallel plate capacitor when a conducting slab is inserted between its plates.
View SolutionA slab of copper of thickness b is inserted in between the plates of a parallel plate capacitor as shown in figure. The separation of the plates is d. If b=d/2, then the ratio of capacities of the capacitor after and before inserting the slab will be
View SolutionIf a dielectric slab of thickness 5 mm and dielectric constant K=6 is introduced between the plates of a parallel plate air capacitor, with plate separation of 8 mm, then its capacitance is
View Solution
