Two cells of emf E1andE2 having internal resistance r1andr2 are connected in parallel. Calculate Eeq and req for the combination.
To solve the problem of finding the equivalent EMF (E_eq) and equivalent internal resistance (R_eq) of two cells connected in parallel, we can follow these steps:
Step 1: Understand the Configuration
We have two cells connected in parallel:
- Cell 1 has an EMF of E1 and an internal resistance of R1.
- Cell 2 has an EMF of E2 and an internal resistance of R2.
Step 2: Write the Formula for Equivalent EMF
In a parallel connection, the equivalent EMF (E_eq) can be calculated using the formula:
Eeq=E1R2+E2R1R1+R2
This formula is derived from the fact that the voltage across each branch (cell) is the same in a parallel circuit.
Step 3: Write the Formula for Equivalent Resistance
The equivalent internal resistance (R_eq) for resistors in parallel is given by:
1Req=1R1+1R2
This can be rearranged to find R_eq:
Req=R1R2R1+R2
Step 4: Combine the Results
Now we have both E_eq and R_eq:
- Equivalent EMF:
Eeq=E1R2+E2R1R1+R2
- Equivalent Resistance:
Req=R1R2R1+R2
Final Answer
Thus, the equivalent EMF and equivalent resistance for the two cells connected in parallel are:
Eeq=E1R2+E2R1R1+R2
Req=R1R2R1+R2
We have two cells connected in parallel:
- Cell 1 has an EMF of E1 and an internal resistance of R1.
- Cell 2 has an EMF of E2 and an internal resistance of R2.
In a parallel connection, the equivalent EMF (E_eq) can be calculated using the formula:
Eeq=E1R2+E2R1R1+R2
This formula is derived from the fact that the voltage across each branch (cell) is the same in a parallel circuit.
The equivalent internal resistance (R_eq) for resistors in parallel is given by:
1Req=1R1+1R2
This can be rearranged to find R_eq:
Req=R1R2R1+R2
Now we have both E_eq and R_eq:
- Equivalent EMF:
Eeq=E1R2+E2R1R1+R2
- Equivalent Resistance:
Req=R1R2R1+R2
Thus, the equivalent EMF and equivalent resistance for the two cells connected in parallel are:
Eeq=E1R2+E2R1R1+R2
Req=R1R2R1+R2
Topper's Solved these Questions
- ELECTROSTATICS AND CURRENT ELECTRICITY BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROSTATICS AND CURRENT ELECTRICITY EXERCISE - LONG ANSWER QUESTIONS
6 Videos
- ELECTROSTATICS AND CURRENT ELECTRICITY BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROSTATICS AND CURRENT ELECTRICITY EXERCISE - NUMERICALS
49 Videos
- ELECTROSTATICS AND CURRENT ELECTRICITY BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROSTATICS AND CURRENT ELECTRICITY EXERCISE - SHORT ANSWER QUESTIONS (2 MARKS)
59 Videos
- ELECTRONIC DEVICES BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTRONIC DEVICES EXERCISE - NUMERICALS
7 Videos
- SAMPLE QUESTION PAPER 2019BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - SAMPLE QUESTION PAPER 2019EXERCISE - Section C
19 Videos
Similar Questions
Two cells of emf ε1 and ε2 having internal resistances r1 and r2 respectively are connected in parallel as shown in Fig. Deduce the expressions for the equivalent emf and equivalent internal resistance of a cell which can replace the combination between the pointsB1 and B2 .

View SolutionTwo cells of emfs 1.5 V and 2.0 V having internal resistances 0.2Ω and 0.3Ω respectively are connected in parallel. Calculate the emf and internal resistance of the equivalent cell.
View Solution
Knowledge Check
Two batteris of emf ε1 and ε2 wit respective internal resistance r1 and r2 are connected in parallel. Now
AThe effective emf of this parallel combination is (ε1r1+ε2r2)(r1+r2)BThe effective internal resistance of this combination is 2r1r2(r1+r2)Cthe effective emf of this parallel combination is (ε1r2+ε2r1)(r1+r2)DThe effective internal resistance of this combination is r1r2(r1+r2)
Two cells of emfs 1.5 V and 2.0v having internal resistance 0.2Σ and 0.3 Σ respectively are connected in parallel . Calculate the emf and internal resistance of the equivalent cell .
View SolutionTwo cells of emfs 1.5 V and 2.0v having internal resistance 0.2Σ and 0.3 Σ respectively are connected in parallel . Calculate the emf and internal resistance of the equivalent cell .
View Solution(a) Two cells of emf ε1 and ε2 having internal resistances r1 andr2 respectively are connected in parallel as shown in Fig. Deduce the expressions for the equivalent emf and equivalent internal resistance of a cell which can replace the combination between the points B1 and B2 . (b) Calculate the current shown by the ammeter in the circuit diagram given below
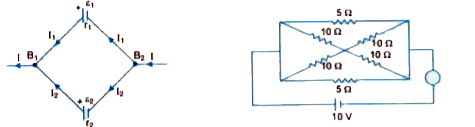
View SolutionTwo batteries of e.m.f. E1 and E2 and internal resistance r1 and r2 are connected in parallel. Determine their equivelent e.m.f.
View SolutionTwo cells of equal e.m.f. E b ut of difference internal resistance r1 and r2 are connected in parallel and the combination is connected with an external resistance R to obtain maximum power in R. find the value of R

View SolutionTwo cells of emfs ε1andε2 and internal resistances r1andr2 respectively are connected in parallel . Obtain expressions for the equivalent (i) resistance and , (ii) emf of the combination.
View SolutionTwo cells of emf 2ε and ε and internal resistance 2r and r respectively, are connected in parallel. Obtain the expressions for the equivalent emf and the internal resistance of the combination.
View Solution
