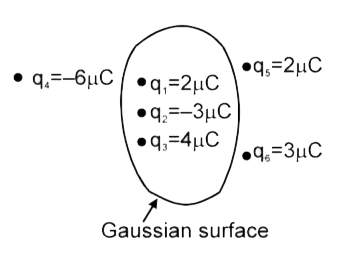
Calculate the net flux emergin from given enclosed surface Nm2C−1

4.5×1011
45×1012
zero
1.12×1012
The correct Answer is:A
∫¯¯¯E.¯¯¯¯ds=qε0=2+5−38.85×10−12=4.5×1011
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NARAYNACHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EXERCISE -2 (C.W.)
39 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NARAYNACHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EXERCISE -2 (H.W)
36 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NARAYNACHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EXERCISE -1 (C.W)
24 Videos
- DUAL NATUREBOOK - NARAYNACHAPTER - DUAL NATUREEXERCISE - LEVEL-II (H.W)
17 Videos
- ELECTRO MAGNETIC INDUCTIONBOOK - NARAYNACHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - Level-II (H.W)
23 Videos
Similar Questions
Knowledge Check
If the electric flux entering and leaving an enclosed surface are ϕ1andϕ2 respectively, then the electric charge present within the surface is
A1∈0(ϕ1+ϕ2)B1∈0(ϕ2−ϕ1)C∈0(ϕ1+ϕ2)D∈0(ϕ2−ϕ1)If the electric flux entering and leaving an enclosed surface respectively is ϕ1 and ϕ2 then the electric charge inside the surface will he
A(ϕ+ϕ2)ε0B(ϕ2−ϕ1)ε0C(ϕ1−ϕ2)ε0D(−ϕ1−ϕ2)ε0If the electric flux entering and leaving an enclosed surface respectively is ϕ1 and ϕ2, the electric charge inside the surface will be
Aϕ3−ϕ1εnBϕ2+ϕ1εnC(ϕ1−ϕ3)εnDεn(ϕ2−ϕ1)
A spherical Gaussian surface encloses a charge of 8.85×10−8C (i) Calculate the electric flux passing through the surface (ii) If the radius of Gaussian surface is doubled, how would the flux change ?
View SolutionWhat is the total charge enclosed by a closed surface if the electric flux entering and leaving the surface are 20000 NC−1m2 and 30000 NC−1m2 respectively.
View SolutionCalculate the total charge enclosed by a closed surface, the number of lines of force emerging from it is 15000 and that entering it is 5000.
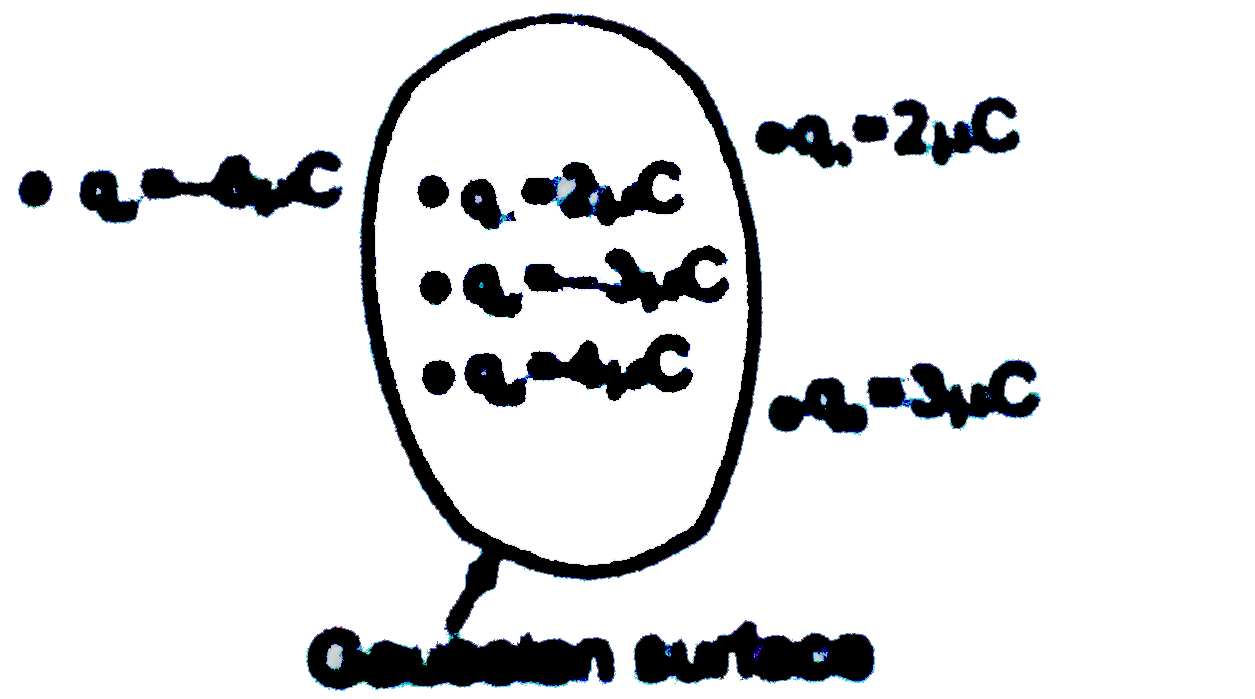
View SolutionFind out flux through the given Gaussian surface.
View SolutionIf the electric flux entering and leaving an enclosed surface respectively is ϕ1 and ϕ2, the electric charge inside the surface will be
View Solution