Four conductors of same resistance connected to form a square. If the resistance between diagonally opposite corners is , the resistive between any two adjacent corners is
32 ohm
8 ohm
ohm
6 ohm
The correct Answer is:D
To solve the problem of finding the resistance between any two adjacent corners of a square formed by four conductors of the same resistance, we can follow these steps:
1. Understanding the Configuration:
- We have a square formed by four resistors, each with resistance R. The corners of the square are labeled A, B, C, and D.
- The resistors are connected as follows: AB, BC, CD, and DA.
2. Resistance Between Diagonally Opposite Corners:
- The resistance between corners A and C (diagonally opposite) is given as 8Ω.
- When calculating the resistance between A and C, we can see that the path consists of two resistors in series (AB and CD) and two resistors in parallel (AD and BC).
3. Calculating the Equivalent Resistance:
- The total resistance RAC can be expressed as:
RAC=R+R2+R+R+R2=2R
- Since RAC=8Ω, we have:
2R=8⟹R=4Ω
4. Finding Resistance Between Adjacent Corners:
- Now, we need to find the resistance between two adjacent corners, say A and B.
- The resistors AB and AD are in series, while the resistors BC and CD are in parallel with respect to the path from A to B.
5. Calculating the Resistance Between A and B:
- The equivalent resistance RAB can be calculated as:
RAB=R+(R⋅RR+R)
- Substituting R=4Ω:
RAB=4+(4⋅44+4)=4+(168)=4+2=6Ω
Final Answer:
The resistance between any two adjacent corners is 6Ω.
- We have a square formed by four resistors, each with resistance R. The corners of the square are labeled A, B, C, and D.
- The resistors are connected as follows: AB, BC, CD, and DA.
- The resistance between corners A and C (diagonally opposite) is given as 8Ω.
- When calculating the resistance between A and C, we can see that the path consists of two resistors in series (AB and CD) and two resistors in parallel (AD and BC).
- The total resistance RAC can be expressed as:
RAC=R+R2+R+R+R2=2R
- Since RAC=8Ω, we have:
2R=8⟹R=4Ω
- Now, we need to find the resistance between two adjacent corners, say A and B.
- The resistors AB and AD are in series, while the resistors BC and CD are in parallel with respect to the path from A to B.
- The equivalent resistance RAB can be calculated as:
RAB=R+(R⋅RR+R)
- Substituting R=4Ω:
RAB=4+(4⋅44+4)=4+(168)=4+2=6Ω
The resistance between any two adjacent corners is 6Ω.
Similar Questions
Twelve resistors of equal value R are connected in the form of a cube. Effective resistance between diagonals of the cube is
 View Solution
View SolutionTwelve wires of equal length and same cross-section are connected in the form of a cube. If the resistance of each of the wires is R, and the effective resistance between the two diagonal ends is Re, then the ratio RRe is equal to __.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Four conductors of same resistance connected to form a square. If the resistance between diagonally opposite corners is 8 ohm, the resistance between any two adjacent corners is
A32 ohmB8 ohmC16 ohmD6 ohm - Question 2 - Select One or More
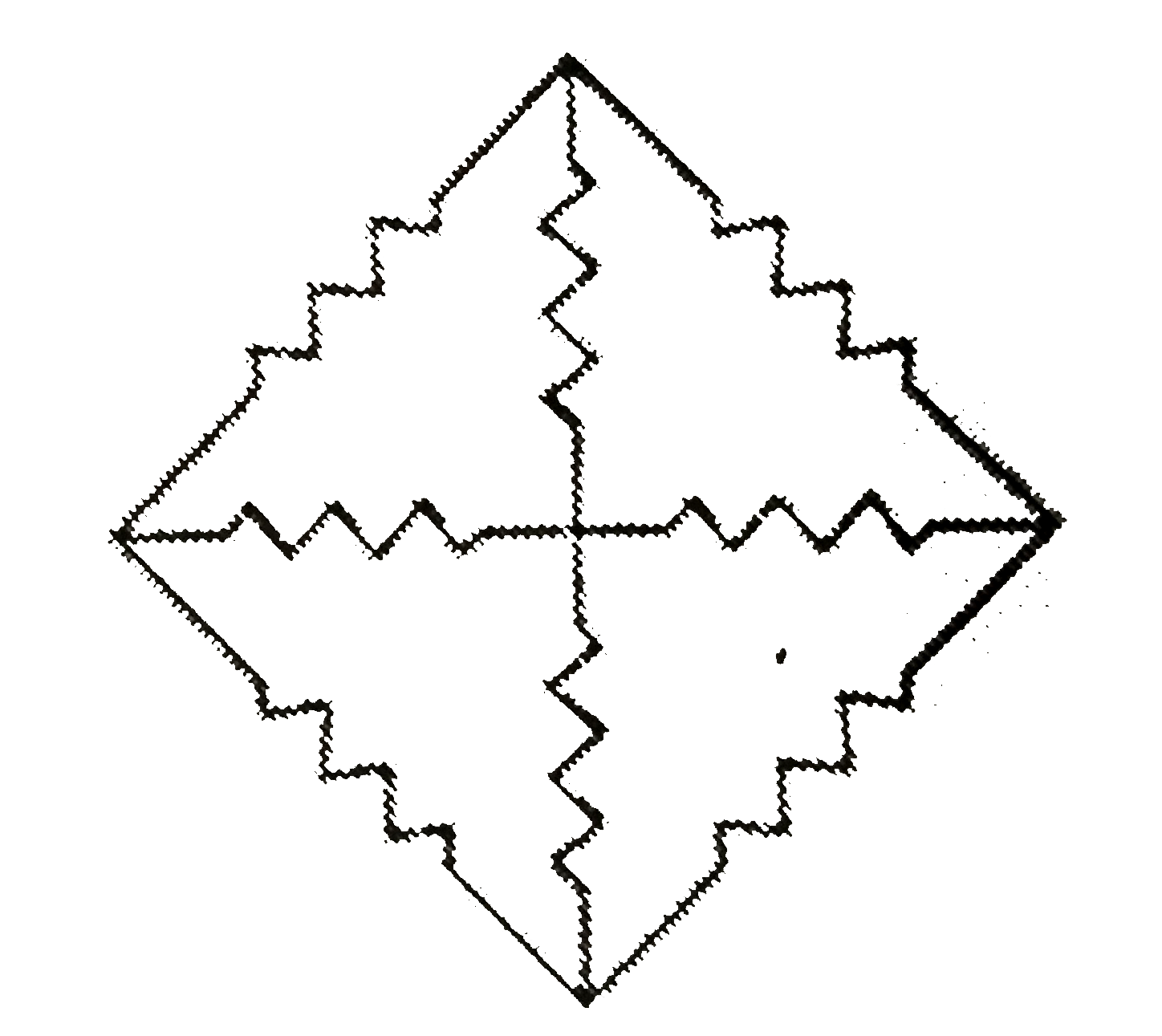
In the network shown, each resistance is equal to R. The equivalent resistance between diagonally opposite corners isARBR/3C2R/3D4R/3 - Question 3 - Select One
Twelve wires, each of resistance 6Ω are connected to form a cube. The effective resistance between two diagonally opposite corners of the cube is
A6ΩB12ΩC5ΩD10Ω
A metallic wire of resistance 12Ω is bent to form a square. The resistance between two diagonal points would be
View SolutionA metallic wire of resistance 12Ω is bent of form a square. The resistance between two diagonal points would be
View SolutionThree resistance each of 4Ω are connected to form a triangle. The resistance between any two terminals is
View SolutionFour wires of equal length and of resistance 5ohm each are connected in the form of a square. The equivalent resistance between the diagonally opposite corners of the square is
View SolutionFour resistances of 100Ω each are connected in the form of square. Then, the effective resistance along the diagonal points is
View Solution

