A thin uniform annula disc (see figure) of mass M has outer radius 4R and inner radius 3R. The work required to take a unit mass from point P on its axis on infinity is

2GM7R(4√2−5)
−2GM7R(4√2−5)
GM2R
2GM5R(√2−1)
The correct Answer is:A
dm=M(2πr)drπ(16R2−9R2)4R
dV=−G(dm)√r2+16R2,V=∫4R3RdV
W=m[V∞−V]
Topper's Solved these Questions
Similar Questions
A thin uniform disc (see figure) of mass M has outer radius 4R and inner radius 3R. The work required to take a unit mass for point P on its axis to infinity is
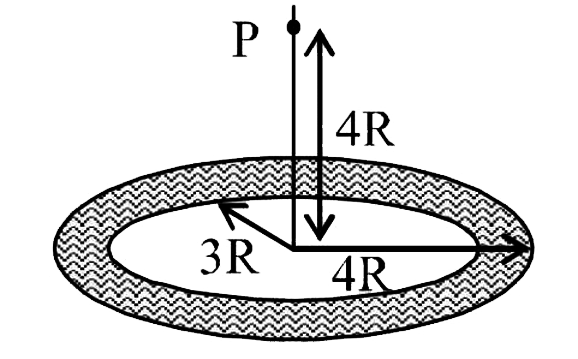
View SolutionA thin uniform disc (see figure) of mass M has outer radius 4R and inner radius 3R. The work required to take a unit mass for point P on its axis to infinity is

View Solution
Knowledge Check
A thin uniform disc (see figure) of mass M has outer radius 4R and inner radius 3R. The work required to take a unit mass for point P on its axis to infinity is
 A2GM7R(4√2−5)B−2GM7R(4√−5)CGM4RD2GM5R(√2−1)
A2GM7R(4√2−5)B−2GM7R(4√−5)CGM4RD2GM5R(√2−1)The energy required to move a body of massm from an orbit of radius 3R to 4R is
AGMm2RBGMm6RCGMm12RDGMm24RThe moment of inertia of an annular disc of mass M, outer and inner radii R and r, about its diameter is
AM(R2+r2)/2BMR2CMr2DMR2+r24
A thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω . Another disc of same dimensions but of mass 14 M is placed gently on the first disc co-axially. The angular velocity of the system is
View Solution
A uniform disc of mass M and radius R is pivoted about the horizontal axis through its centre C A point mass m is glued to the disc at its rim, as shown in figure. If the system is released from rest, find the angular velocity of the disc when m reaches the bottom point B.
View SolutionThe moment of inertia of an annular disc of mass M, outer and inner radii R and r, about its diameter is
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω . Another disc of same dimensions but of mass 14 M is placed gently on the first disc co-axially. The angular velocity of the system is
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω. Another disc of same dimensions but of mass M/4 is placed gently on the first disc coaxially. The angular velocity of the system now is
View Solution
