In a series L.R circuit, power of 400 W is dissipated from a source of 250 V, 50 Hz. The power factor of the circuit is 0.8. In order to bring the power factor to unity, a capactor of value C is added in series to the L and R. Taking the value of C as (n3π)μF, then value of n is ____________
To solve the problem, we will follow these steps:
Step 1: Calculate the RMS current (I_rms)
Given the power (P) and the voltage (V_rms), we can use the formula for power in an AC circuit:
P=Vrms⋅Irms⋅cosϕ
Where:
- P=400W
- Vrms=250V
- cosϕ=0.8
Rearranging the formula to find Irms:
Irms=PVrms⋅cosϕ
Substituting the values:
Irms=400250⋅0.8=400200=2A
Step 2: Calculate the resistance (R)
Using the power formula again, we have:
P=I2rms⋅R
Rearranging to find R:
R=PI2rms
Substituting the values:
R=40022=4004=100Ω
Step 3: Calculate the inductive reactance (X_L)
Using the power factor relationship, we know:
cosϕ=RZ
Where Z is the impedance and Z=√R2+X2L.
From the power factor:
0.8=100Z⟹Z=1000.8=125Ω
Now, using the impedance formula:
Z2=R2+X2L
Substituting the known values:
1252=1002+X2L
Calculating:
15625=10000+X2L⟹X2L=5625⟹XL=75Ω
Step 4: Determine the required capacitive reactance (X_C)
To achieve a power factor of 1 (unity), we need XL=XC:
XC=75Ω
Step 5: Calculate the capacitance (C)
The capacitive reactance is given by:
XC=1ωC
Where ω=2πf and f=50Hz:
ω=2π⋅50=100πrad/s
Setting XC=75:
75=1100πC
Rearranging to find C:
C=175⋅100π=17500πF
Step 6: Convert capacitance to microfarads
To convert C to microfarads:
C=17500π⋅106μF=1067500πμF
Step 7: Express C in the form n3π
We need to express C in the form n3π:
C=1067500π=4003πμF
Thus, comparing with n3π:
n=400
Final Answer
The value of n is 400.
---
Given the power (P) and the voltage (V_rms), we can use the formula for power in an AC circuit:
- P=400W
- Vrms=250V
- cosϕ=0.8
Using the power formula again, we have:
Using the power factor relationship, we know:
To achieve a power factor of 1 (unity), we need XL=XC:
The capacitive reactance is given by:
To convert C to microfarads:
We need to express C in the form n3π:
The value of n is 400.
Topper's Solved these Questions
Similar Questions
In a LCR series a.c. circuit ……….. power is dissipated at resonance, as value of power factor is………………
View SolutionA circuit draws a power of 600 W from an AC source of 200 V 50 Hz . The power factor of the circuit is 0.6 . The current lags behing the voltage . What value of capacitance should be connected in the circuit to bring its power factor to unity?
View Solution
Knowledge Check
For a series L C R circuit, the power loss at resonance is
AV2ωL−1ωCBi2CωCi2RDV2ωCIn a series resonant L-C-R circuit, the power factor is
A0B1C0.3D1.5In a series LCCR circuit having L=30mH,R=8Ω and the resonant frequency is 50 Hz. The quality factor of the circuit is
A0.118B11.8C118D1.18
In series L-C-R circuit the quality factor is
View SolutionIn series L-C-R circuit the quality factor is
View SolutionIn the given circuit, power factor between A and B is 0.5 and over all power factor of the circuit is 1.
Find the value of Rand L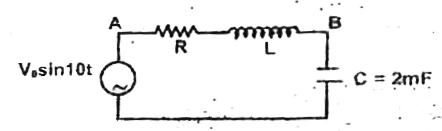
View SolutionThe power factor is an L-C-R circuit at resonance is
View SolutionA circuit draws a power of 550 watt from a source of 220 volt, 50Hz. The power factor of the circuit is 0.8 and the current lags in phase behind the potential difference. To make the power factor of the circuit as 1.0, The capacitance should be connected in series with it is
View Solution
