A man walks for some time 't' with velocity(v) due east. Then he walks for same time 't' with velocity (v) due north. The average velocity of the man is:-
2v cos40∘
√2v
v
v√2
The correct Answer is:D
To solve the problem of finding the average velocity of a man who walks due east and then due north, we can follow these steps:
Step 1: Understand the Motion
The man walks for time 't' with velocity 'v' due east and then for the same time 't' with velocity 'v' due north.
Step 2: Calculate the Displacement
1. Displacement in East Direction:
- Distance covered in the east direction = velocity × time = v×t.
2. Displacement in North Direction:
- Distance covered in the north direction = velocity × time = v×t.
3. Resultant Displacement:
- Since the man moves in two perpendicular directions (east and north), we can use the Pythagorean theorem to find the resultant displacement S:
S=√(vt)2+(vt)2=√2(vt)2=vt√2
Step 3: Calculate the Total Time
- The total time taken for the journey is the sum of the time spent walking in both directions:
Total Time=t+t=2t
Step 4: Calculate the Average Velocity
- The average velocity Vavg is defined as the total displacement divided by the total time:
Vavg=DisplacementTotal Time=S2t=vt√22t
- Simplifying this gives:
Vavg=v√22
Final Answer
Thus, the average velocity of the man is:
v√22
The man walks for time 't' with velocity 'v' due east and then for the same time 't' with velocity 'v' due north.
1. Displacement in East Direction:
- Distance covered in the east direction = velocity × time = v×t.
2. Displacement in North Direction:
- Distance covered in the north direction = velocity × time = v×t.
- Since the man moves in two perpendicular directions (east and north), we can use the Pythagorean theorem to find the resultant displacement S:
S=√(vt)2+(vt)2=√2(vt)2=vt√2
- The total time taken for the journey is the sum of the time spent walking in both directions:
Total Time=t+t=2t
- The average velocity Vavg is defined as the total displacement divided by the total time:
Vavg=DisplacementTotal Time=S2t=vt√22t
- Simplifying this gives:
Vavg=v√22
Thus, the average velocity of the man is:
v√22
Topper's Solved these Questions
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - EXERCISE-3
41 Videos
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - EXERCISE-1
99 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
48 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
Similar Questions
A man traversed half the distance with a velocity v0. The remaining part of the distance was covered with velocity V1. For half the time and with velocity v2 for the other half of the time . Find the average speed of the man over the whole time of motion.
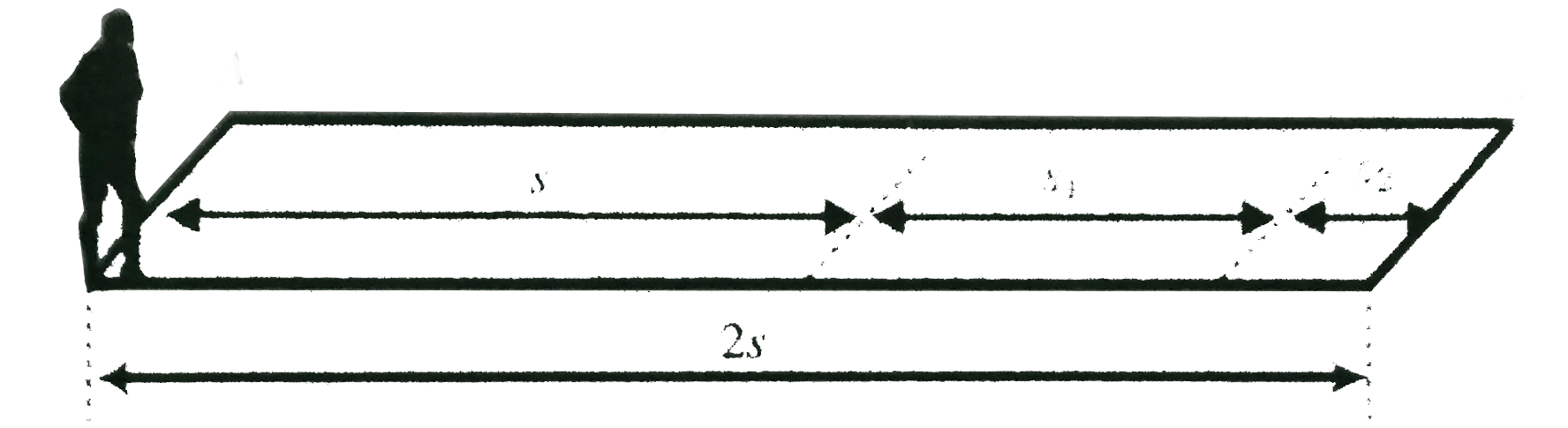 .
.
View SolutionA block moves in a straight line with velocity v for time t0. Then, its velocity becomes 2v for next t0 time. Finally, its velocity becomes 3v for time T. If average velocity during the complete journey was 2. 5 v, then find T in terms of t0.
View Solution
Knowledge Check
A body moves due East with velocity 20km/hour and then due North with velocity 15km/hour. The resultant velocity
A5km/hourB15km/hourC20km/hourD25km/hourSeeta is moving due east with a velocity of V1m/s and geets iss moving due west with velocity of V2m/s. The velocity of Seeta with respect to Geeta is
AV1+V2 due eastBV1−V2 due eastCV1−V2 due westDV1+V2 due west.
A body travels with velocity v1fortime t_(1)secondandwithvelocity v_(2) for time t_(2) second in the same direction, fide the avetage velocity of the body.
View SolutionA person travelling on a straight line moves with a uniform velocity v1 for some time and with uniform velocity v2 for the next equal time. The average velocity v is given by
View SolutionA particle starts moving due east with a velocity V1=5 m/sec. for 10 sec, turns to north and moves with a velocity V2=10 m/sec. for 5 sec. Find the average velocity of the particle during 15 sec. from starting.
View SolutionA man is walking due west with a velocity 3km/h and rain appears to be falling vertically with a velocity 4km/h. Find the velocity of rain with respect to the ground.
View Solution(A): Rain is falling vertically downwards with velocity 6 km/hr. A man walks with a velocity of 8 km/hr. Relative velocity of rain w.r.t. the man is 10km/hr.
(R): Relative velocity is the ratio of two velocities.
View SolutionStatement-1 : Rain is falling vertically downwards with velocity 6 km/h. A man walks with a velocity of 8 km/h. Relative velocity of rain w.r.t. the man is 10 km/h. Statement-2 : Relative velocity is the ratio of two velocities
View Solution
