A water barrel stands on a table of height h. If a small holes is punched in the side of the barrel at its base, it is found that the resultant stream of water strikes the ground at a horizontal distance R from the table. What is the depth of water in the barrel?
R2
R24h
R2h
h2
The correct Answer is:B
R=vt
R=√2gd√2hg
d=R24h
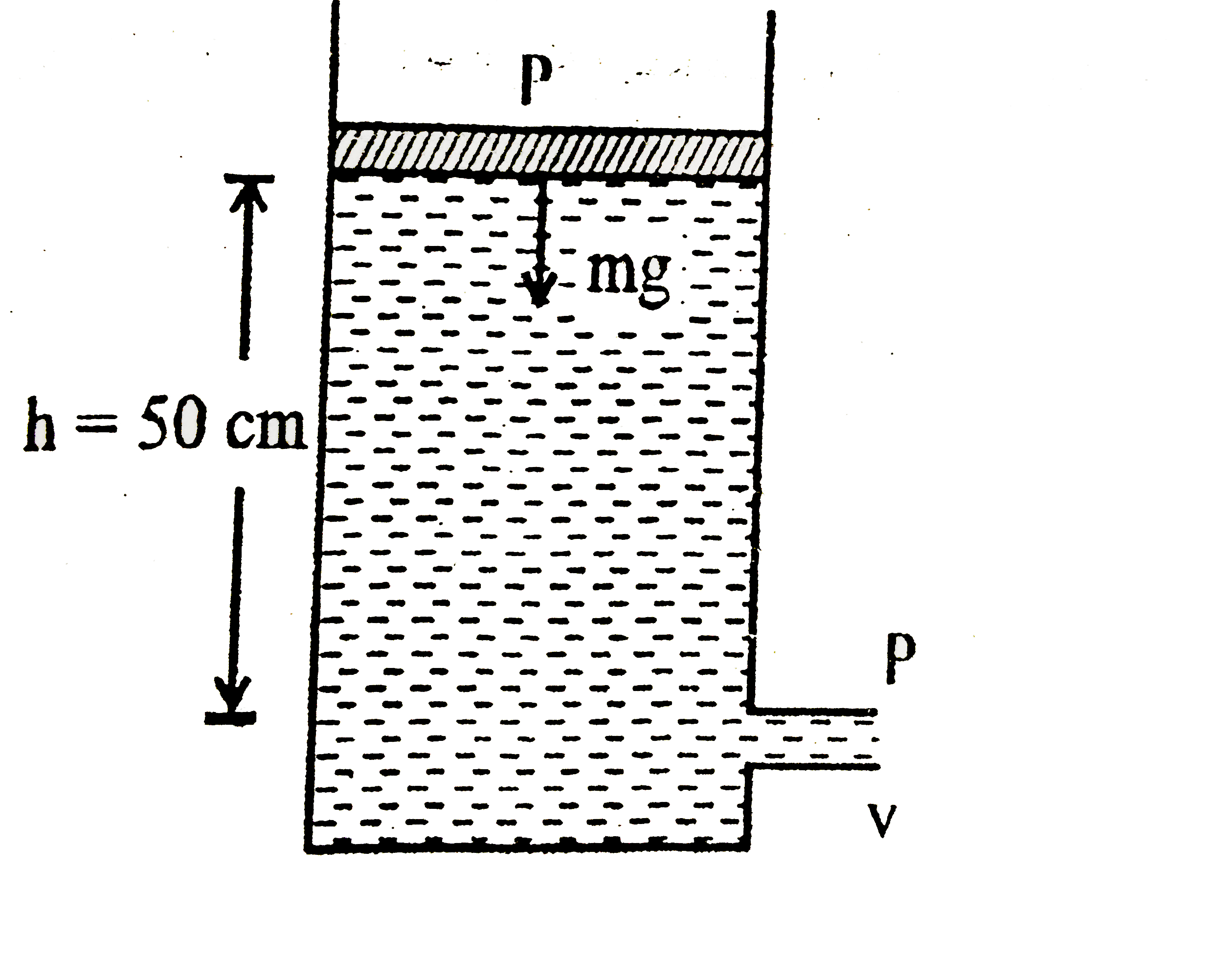
Applying Bernoulli theorem
(P+mgA)+12ρ(0)2+ρgh=P+12ρv2+0
mgA+ρgh=12ρv2
10×100.1+1000×10×0.5=12×1000×v2
1000+5000=500v2
v=2√3m/s⇒v=3.4m/s
Topper's Solved these Questions
Similar Questions
A water barrel stands on a table of height h.If a small hole is punched in the side of the side of the barrel at its base. It is found that the resultant stream of water striks the ground at a horizontal distance R from the barrel. What is the depth of water in the berrel?
View SolutionA stream of water escapes from a hole 2m above the base of a tank and strikes the ground at a horizontal distance of 6m .The depth of the hole from the free surface of water is
View Solution
Knowledge Check
A water bgarrel having water up to a depth d is placed on a table of height H. A small hole is made on the wall of the barrel at its bollom. If the stream of water comiong out of the hole falls on the ground at a horizontal distance r from the barrel, then the value of d is
A4Hr2B4Hr2Cr24HDH4r2A tank of height H is fully filled with water. If the water rushing from a hole made in the tank below the free surface , strikes the floor at a maximum horizontal distance , then the depth of the hole from the free surface must be
A34HB23HC14HD12HA water tank standing on the floor has two small holes punched in the vertical wall one above the other. The holes are 2.4 cm and 7.6 cm above the floor. If the jest of water from the holes hit the floor at the same point, then the height of water in the tank is
A10 cmB5 cmC20 cmD4.8 cm
A stream of water escapes from a hole 2m above the Q. 1 base of a tank and strikes the ground at a horizontal distance of 6m .The depth of the hole from the free surface of water is
View SolutionA stream of water escapes from a hole 2m above the Q. 1 base of a tank and strikes the ground at a horizontal distance of 6m .The depth of the hole from the free surface of water is
View SolutionWater stands at a depth H in a tank whose side walls are vertical. A hole is made in one of the walls at a height h below the water surface. The stream of water emerging from the hole strikes the floor at a distance R from the tank, where R is given by
View SolutionA tank is filled by water (ρ=103kg/m3). A small hole is made in the side wall of the tank at depth 10 m below water surface. A water jet emerges horizontal from the hole and falls at horizontal distance R from it. The amount of extra pressure (in terms of atmospheric pressure) that must be applied on the water surface, so that range becomes 3R on the on the ground will be (cross section area of hole is negligible and 1 atm = 105 Pa, g = 10 m/s2)
View SolutionA tank is filled with water up to a height H. Water is allowed to come out of a hole P in one of the walls at a depth D below the surface of water. Express the horizontal distance x in terms of H and D
 .
.
View Solution