A uniform magnetic field (where is a positive constant) fills a cylindrical volume of radius then the potential difference in the conducting rod due to electrostatic field is :
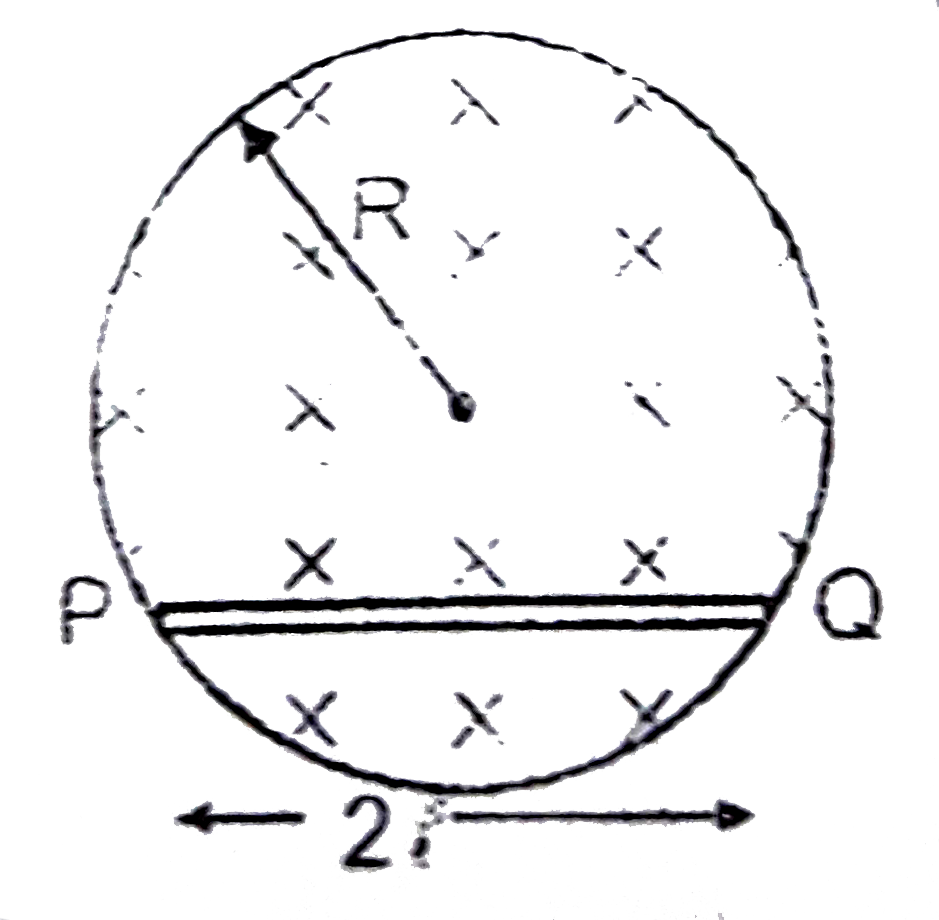
The correct Answer is:C
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-2 PART 2
17 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-2 PART 3
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-1 PART 2
51 Videos
- ELECTRODYNAMICSBOOK - RESONANCECHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP
39 Videos
Similar Questions
a uniform magnetic field of induction B fills a cylindrical volume of radius R. A rod AB of length 2l is placed as shown in . If B changing at the rate dB/dt, the emf that is produced by the changing magnetic field and that acts between the ends of the rod is
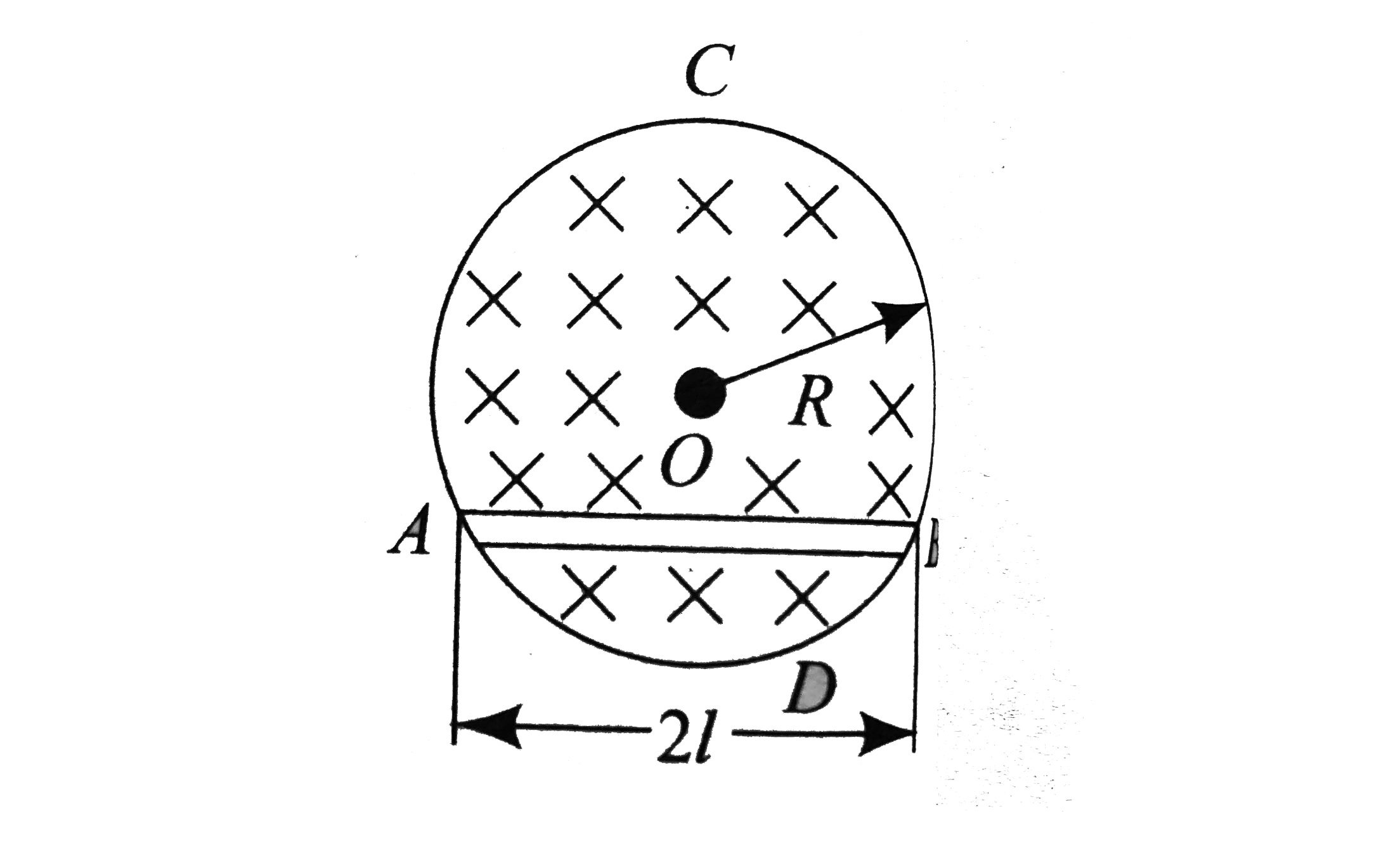
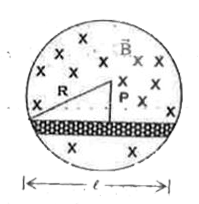
View SolutionA uniform magneitc field B fills a cylindrical volume of radius R, A metal rod of length l is placed as shown. If B is changing at the rate of dB/dt, the emf that is produced by the changing magnetic field is ξ here p = √R2−(l2)2. then
View Solution
Knowledge Check
A uniform magnetic field, B=B0t (where B0 is a positive constant) , fills a cylinderical volume of radius R, then the potential difference in the conducting rod PQ due to electorstatic field is :
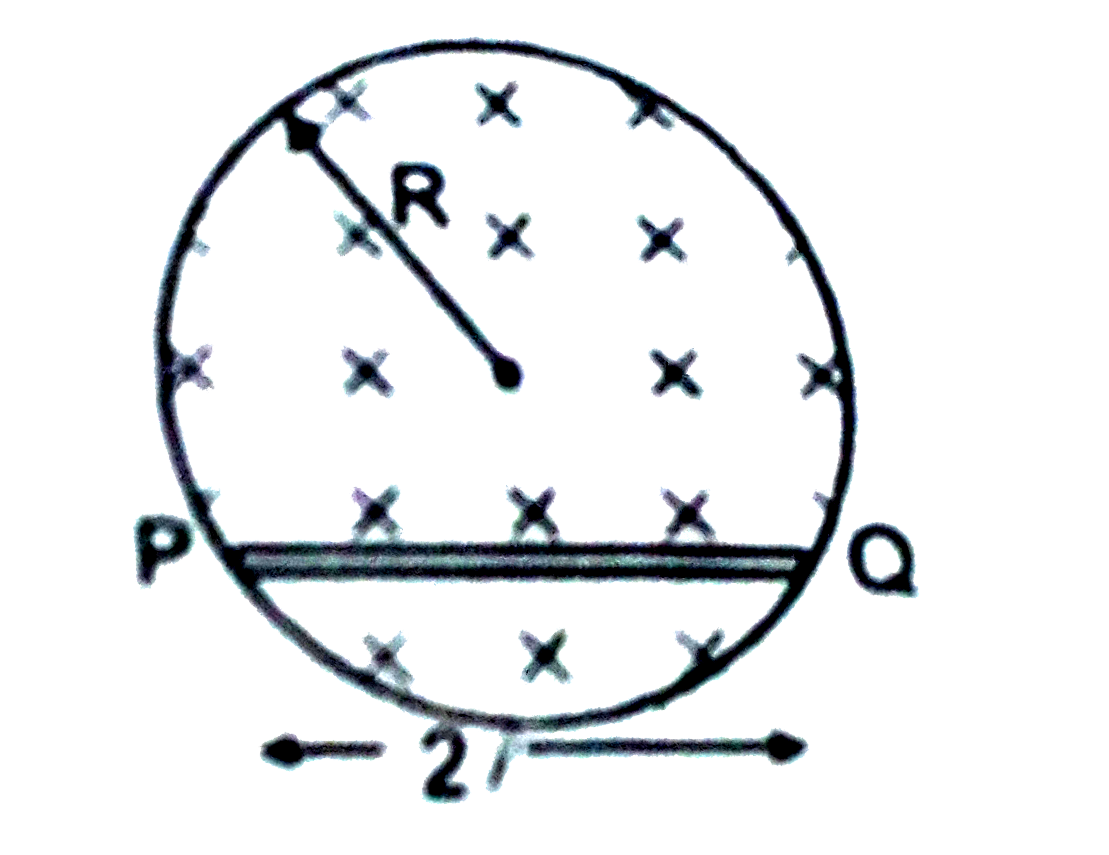 AB0l√R2+l2BB0l√R2−l24CB0l√R2−l2DB0R√R2−l2
AB0l√R2+l2BB0l√R2−l24CB0l√R2−l2DB0R√R2−l2a uniform magnetic field of induction B fills a cylinderical volume of radius R. A rod AB of length 2l is placed as shown in . If B changing at the rate dB/dt, the emf that is produced by the changing magnetic field and that acts between the ends of the rod is
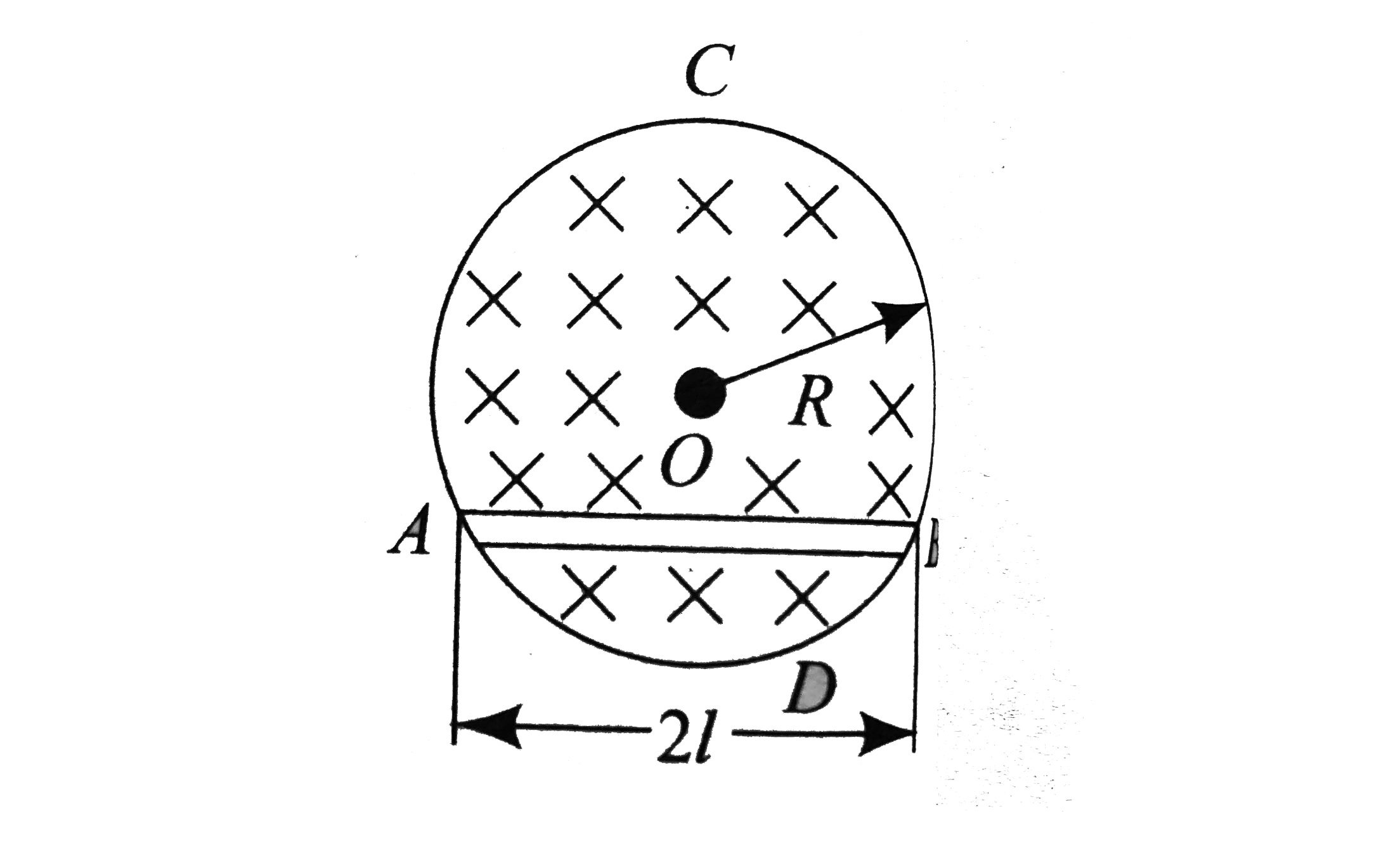 A(a) dBdtl√R2−l2B(b) dBdtl√R2+l2C( c) 12dBdtl√R2−l2D(d) 12dBdtl√R2+l2
A(a) dBdtl√R2−l2B(b) dBdtl√R2+l2C( c) 12dBdtl√R2−l2D(d) 12dBdtl√R2+l2A uniform magneitc field B fills a cylindrical volume of radius R, A metal rod of length l is placed as shown. If B is changing at the rate of dB/dt, the emf that is produced by the changing magnetic field is ξ here p = √R2−(l2)2. then
 AThe magnitude of the electric field created is 12rdBdtBThe magnitude of the electric field component along the length of the rod is 12pdBdtCThe magnitude of the electric field component perpendicular to the rod is zeroDThe induced emf is 12lpdBdt.
AThe magnitude of the electric field created is 12rdBdtBThe magnitude of the electric field component along the length of the rod is 12pdBdtCThe magnitude of the electric field component perpendicular to the rod is zeroDThe induced emf is 12lpdBdt.
In a region at a distance r from z-axis, magnetic field →B=B0rtˆk is present where B0 is constant and t is time. Then the magnetic of induced electric field at a distance r from z-axis is given by
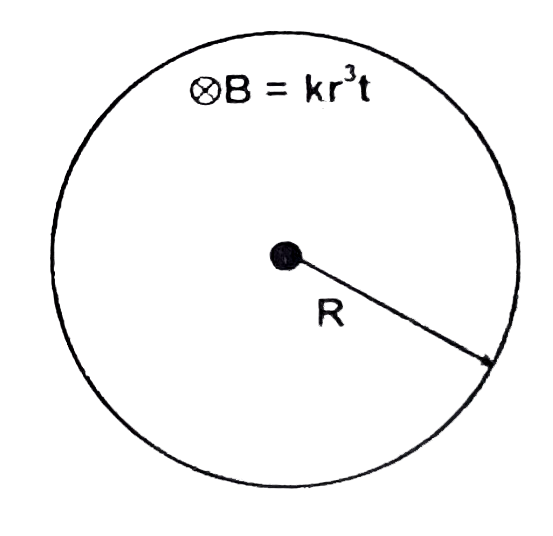
View SolutionA time varying magnetic field B=Kr3t is existing in a cylindrical region of radius 'R' as shown (where k is a constant). The induced electric field 'E' at rR2 is
View SolutionIn a region at a distance r from z-axis, magnetic field →B=B0rtˆk is present where B0 is constant and t is time. Then the magnetic of induced electric field at a distance r from z-axis is given by
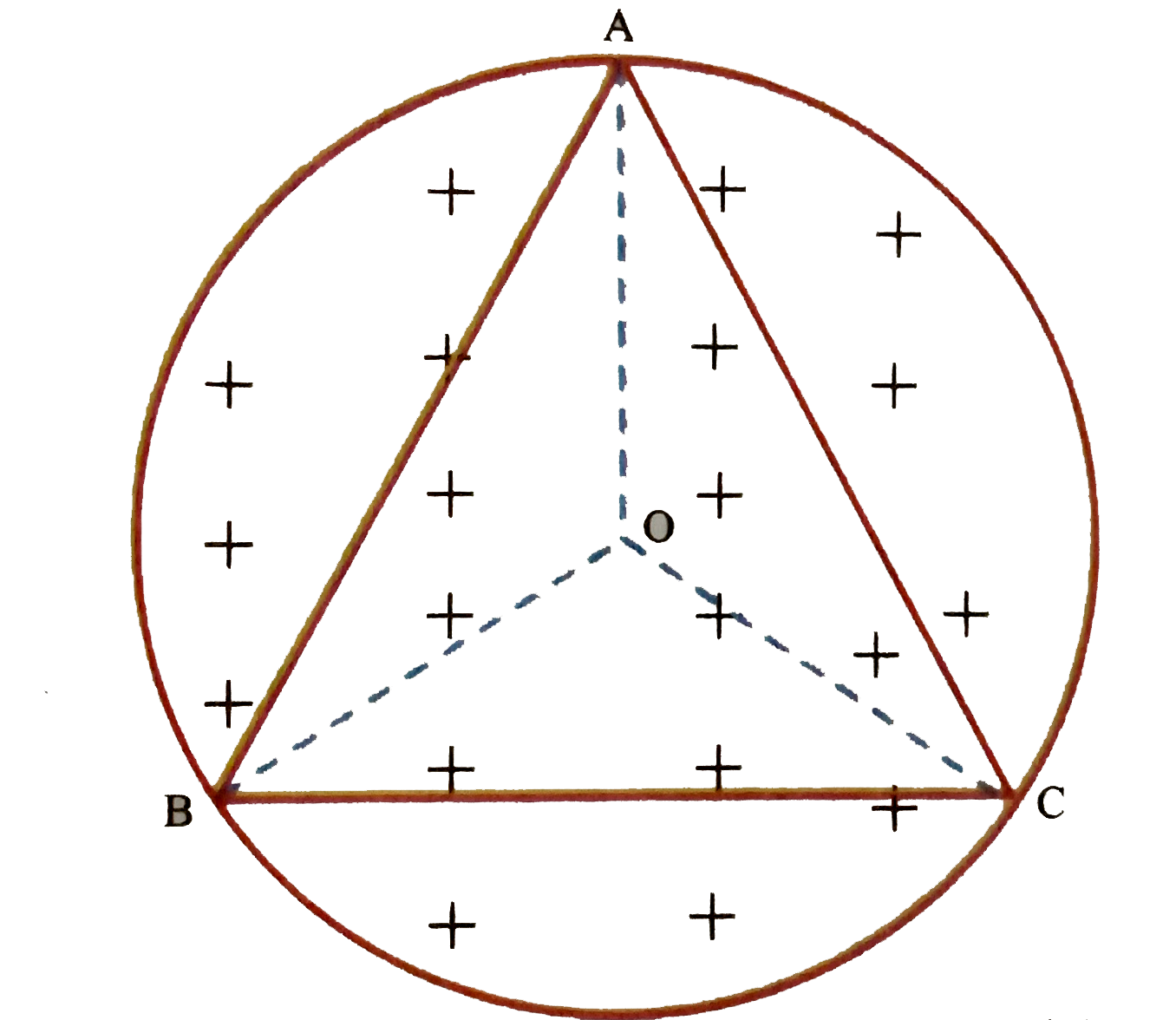
View SolutionIn the figure shown there exists a uniform time varying magnetic field B=[(4T/s)t+0.3T] in a cylinderical region of radius 4m. An equilateral traingualr conducting loop is placed in the magnetic field with its centroide on the axis of the field and its plane perpendicular to the field.
View SolutionA time varying magnetic field B=Kr3t is existing in a cylindrical region of radius 'R' as shown (where k is a constant). The induced electric field 'E' at rR2 is

View Solution
