A conducting disc of radius $R$ is placed in a uniform and constant magnetic field $B$ parallel to the axis of the disc.With what angular speed should the disc be rotated about its axis such that no electric field develops in the disc (the electric charge and mass are $e$ and $m$ )

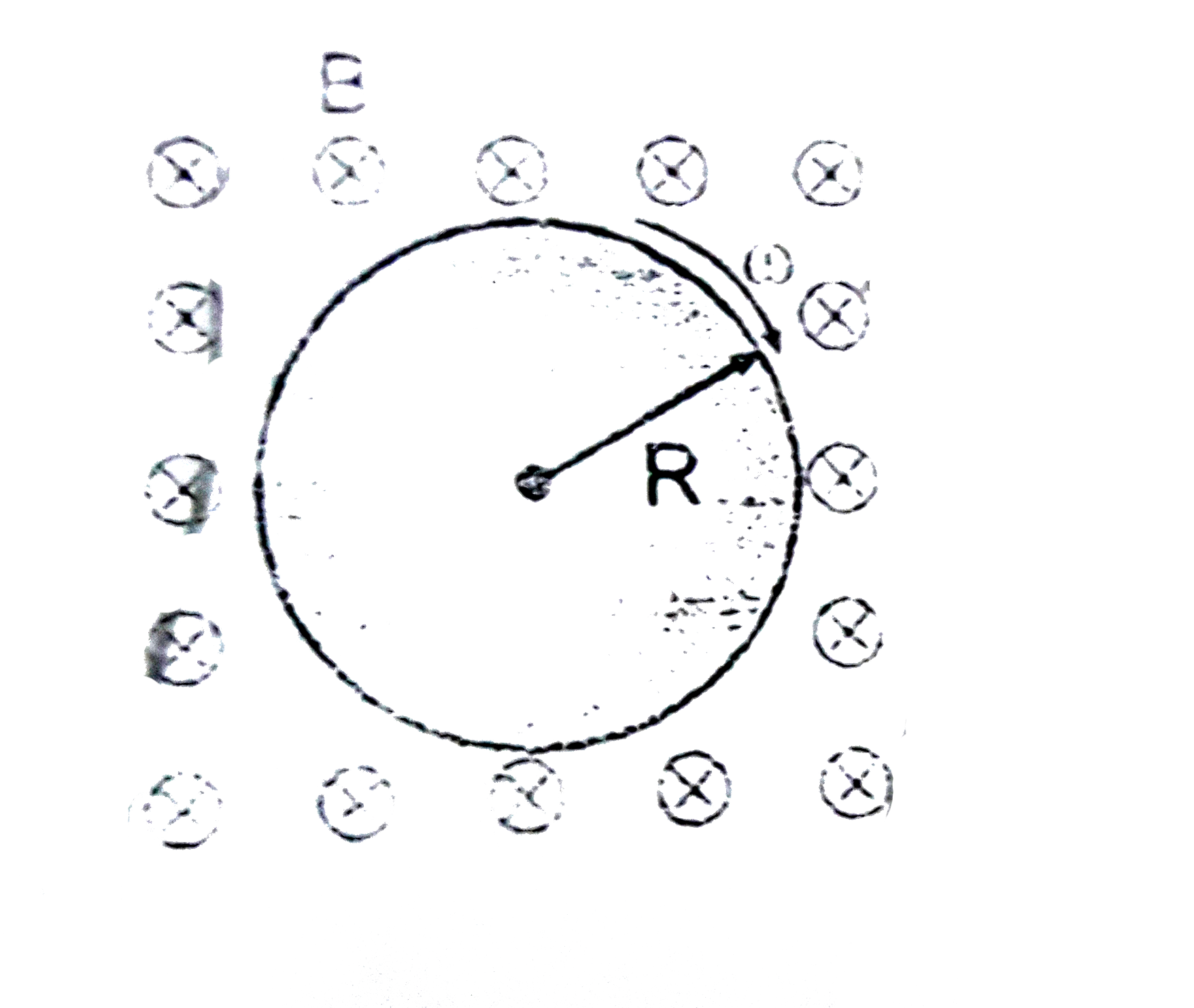
$\frac{e B}{2 m}$

$\frac{e B}{m}$

$\frac{2 π m}{e B}$

$\frac{π m}{e B}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

A brass disc is rotating about its axis. If temperature of disc is increased then its
Aradius of gyration increases, but moment of inertia remains the same
Bmoment of inertia increases but radius of gyration remains the same
Cradius of gyration, moment of inertia both remain the same
Dradius of gyration, moment of inertia both increase
A uniform disc of mass M and radius R is hinged at its centre C. A force F is applied on the disc as shown. At this instant, the angular acceleration of the disc is

A $\sqrt{3} \frac{F}{M R}$
B $\frac{F}{M R}$
C $\frac{2}{\sqrt{3}} \frac{F}{M R}$
D $\frac{F}{2 M R}$
When a circular disc of radius 0.5 m is rotating about its own axis, then the direction of its angular momentum is
Aalong the tangent drawn at every point
Bradial
Cperpendicular to the direction of angular velocity
Dalong the axis of rotation of the body