In certain region of space with volume 0.2 m3 the electric potential is found to be 5V throughout. The magnitude of electric field is this region is
zero
0.5 N/C
1 N/C
5 N/C
To solve the problem, we need to find the magnitude of the electric field in a region where the electric potential is constant.
1. Understand the Concept of Electric Potential and Electric Field:
- The electric potential V at a point in space is defined as the work done per unit charge in bringing a positive test charge from infinity to that point.
- The electric field E is related to the electric potential by the formula:
E=−dVdx
- Here, dVdx represents the rate of change of electric potential with respect to distance.
2. Analyze the Given Information:
- We are given that the electric potential is constant at 5V throughout the region of space with a volume of 0.2m3.
- Since the potential is constant, it means that there is no change in potential in any direction.
3. Calculate the Electric Field:
- Since the electric potential V is constant (5V), the change in potential dV is zero.
- Therefore, we can express this as:
dV=0
- Substituting this into the formula for electric field:
E=−dVdx=−0dx=0
- This indicates that the electric field E in this region is zero.
4. Conclusion:
- The magnitude of the electric field in the region where the electric potential is constant at 5V is:
E=0N/C
Final Answer:
The magnitude of the electric field in this region is 0 N/C.
- The electric potential V at a point in space is defined as the work done per unit charge in bringing a positive test charge from infinity to that point.
- The electric field E is related to the electric potential by the formula:
E=−dVdx
- Here, dVdx represents the rate of change of electric potential with respect to distance.
- We are given that the electric potential is constant at 5V throughout the region of space with a volume of 0.2m3.
- Since the potential is constant, it means that there is no change in potential in any direction.
- Since the electric potential V is constant (5V), the change in potential dV is zero.
- Therefore, we can express this as:
dV=0
- Substituting this into the formula for electric field:
E=−dVdx=−0dx=0
- This indicates that the electric field E in this region is zero.
- The magnitude of the electric field in the region where the electric potential is constant at 5V is:
E=0N/C
The magnitude of the electric field in this region is 0 N/C.
Topper's Solved these Questions
Similar Questions
Knowledge Check
In certain region of space with volume 0.2 m3 the electric potential is found to be 5V throughout. The magnitude of electric field is this region is
A0.5N/CB1N/CC5N/CDzeroIn a certain 0.1m3 of space, electric potential is found to be 5 V throughout. What is the electric field in this region ?
A50V/mBzeroC0.5V/mDNone of theseIn a certain region of space the electric potential V is known to be constant. Is the electric field in this region (a) positive (b) zero ,or (c ) negative ?
AAlso constantBPositiveCNegativeDZero
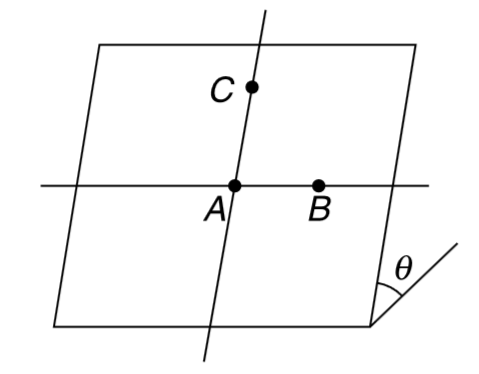
It is known that there exists a uniform electric field in a certain region. Imagine an incline plane (figure) in the region which is inclined at θ=37∘ to the horizontal when one moves horizontally along the incline from A to B (AB=1cm), the electric potential decreases by 10V. Similarly, potential at C(AC=1cm) is less than potential at A by 10 V where line AC lies on the incline and is perpendicualr to AB. When one moves vertically up from point A to a point D(AD=1cm), the potential drop by 10 V again. Find the magnitude of the electric field in the region and the angle that it makes with vertical [given sin37∘=35]
View SolutionIn a certain region of space, the potential field depends on x and y-coordinates as V=(−x2+y2). The corresponding electric field lines in xy-plane are correctly represented by
View SolutionElectric potential at any point is V=−5x+3y+√15z, then the magnitude of the electric field is
View SolutionElectric potential at any point is V=−5x+3y+√15z, then the magnitude of the electric field is
View SolutionIn a certain region of space, the electric field is zero. From this, we can conclude that the electric potential in this region is:
View Solution
