A rod length l is uniformly charged with charge q. Calculate potential at point P.

Take a small element of length dx, at a distance x from left end. Potential due to this small element

DV=K(dp)x ∴ Total potential ⇒ V=x=l∫x=0kdqx
Where dp=qldx ⇒ V=x=r+l∫x=rK(qldx)x=Kqlloge(l+rr)
Topper's Solved these Questions
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Problems
22 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (A)
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCECHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
Similar Questions
Figure shows a rod of length L which is uniformly charged with linear charge density λ kept on a smooth horizontal surface. Right end of rod in contact with a vertical fixed wall. A block of mass m and charge q is projected with a velocity v from a point very far from rod in the line of rod. find the distance of closest approach between the block & the left end A of the rod.

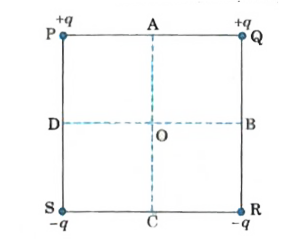
View SolutionThere is a, and four charges +q, +q, -q and -q are fixed at the four corners of square. See the figure for the placement of charges.
Calculate:
(i) Electric potential at point O.
(ii) Work done to move a charge +e from point O to point B.
(iii) Work done to move a charge +e from point O to A.
View Solution
Knowledge Check
A ring of radius R is charged uniformly with a charge +Q. The potential at a point on its axis at a distance 'r' from any point on ring will be-
AkQ√R2+r2BkQrCkQ(r2+R2)3/2DkQr2(r2−R2)1/2A rod of length l having uniformly distributed charge Q is rotated about one end with constant frequency f Its magnetic moment
AπfQl2BπfQl23C2πfQl23D2πRQ2A rod of length l having uniformly distributed charge Q is rotated about one end with constant frequency 'f'. Its magnetic moment is πfQl2n Then the value of n is
A7B5C9D3
A rod of length l, has a uniform positive charge per unit length and a total charge Q. calculate the electric foeld at a point P located along the long axis of the rod and at a distance a from one end

View SolutionFind the electric potential at the axis of a uniformly charged disc and use potential to find the electric field at same point.
View SolutionA thin straight rod of length l carrying a uniformly distributed charge q is located in vacuum. Find the magnitude of the electric force on a point charge 'Q' kept as shown in the figure.

View SolutionThe outer curved surface of a cone of slant length l is shown is figure is charged uniformly with the charge Q .Find the potential at the vertex of the cone.
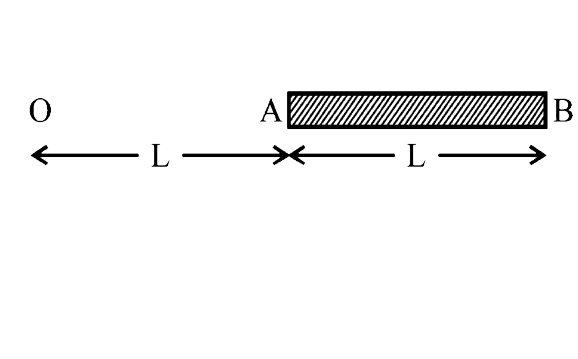
View SolutionA charge Q is uniformly distributed over a long rod AB of length L as shown in the figure. The electric potential at the point O lying at distance L from the end A is
View Solution