In a double star, two stars (one of mass $m$ and the other of mass $2 m$ ) distance $d$ apart rotate about their common centre of mass., Deduce an expressioin for the period of revolution. Show that te ratio of their angular momenta about the centre of mass is the same as the ratio of their kinetic energies.

Video Solution

Text Solution

Verified by Experts

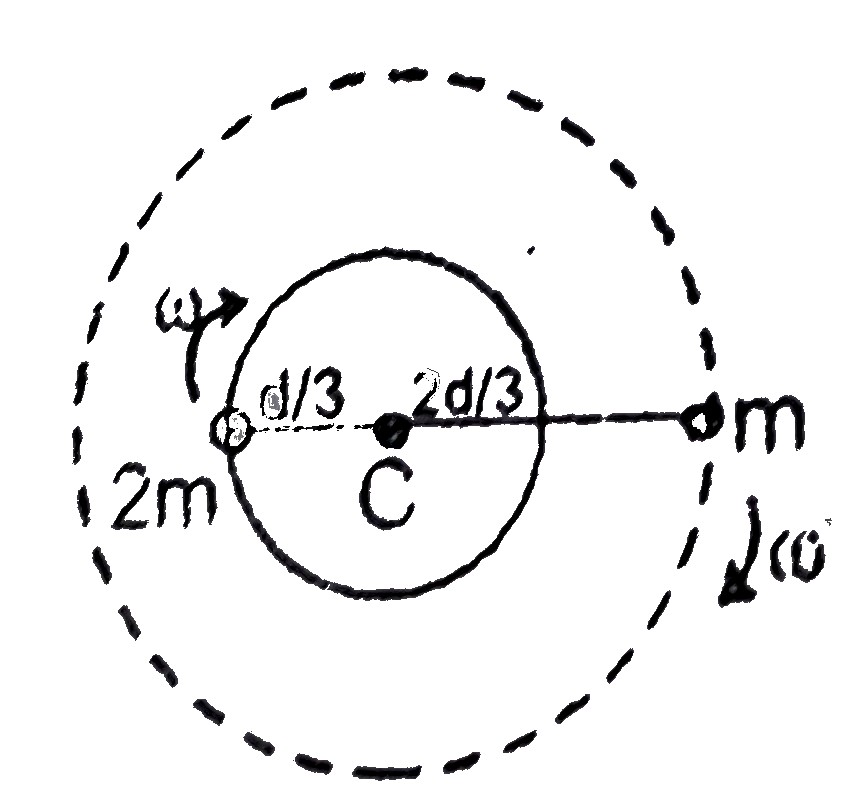
The centre of mass $C$ will be at distances $d / 3$ and $2 d / 3$ from the masses $2 m$ and m respectively Both the stars rotate round $C$ in their respective orbits with the same angular velocity $ω$ the gravitational force acting on each star due to the other supplies the necessary centripetal force The gravitational force on either star is $\frac{G (2 m) m}{d^{2}}$ If we consider the rotation of the smaller star, the centripetal force $(m r ω^{2}) i s [m (\frac{2 d}{3}) ω^{2}]$ and for bigger star $[\frac{2 m d ω^{2}}{3}]$ i.e same
$∴ \frac{G (2 m) m}{d^{2}} = m (\frac{2 d}{3}) ω^{2}$ or $ω = \sqrt{\frac{3 g m}{d^{3}}}$
Therefore, the period of revolution is given by $T = \frac{2 π}{ω} = 2 π \sqrt{\frac{d^{3}}{3 G m}}$
The ratio of the angular momentum is $\frac{{(I ω)}_{b i g}}{{(i ω)}_{s m a l l}} = \frac{I_{b i g}}{I_{s m a l l}} = \frac{(2 m) {(\frac{d}{3})}^{2}}{m {(\frac{2 d}{3})}^{2}} = \frac{1}{2}$
since $ω$ is same for both The ratio of their kinetic energygies is $\frac{{(\frac{1}{2} I ω^{2})}_{b i g}^{2}}{{(\frac{1}{2} I ω^{2})}_{s m a l l}^{2}} = \frac{I_{b i g}}{I_{s m a l l}} = \frac{1}{2}$
which is the same as the ratio of their angular momentum .
.

Updated on:21/07/2023

Two stars of masses m and 2m at a distance d rotate about their common centre of mass in free space. The period of revolution is :
A $\frac{1}{2 π} \sqrt{\frac{d^{3}}{3 G m}}$
B $2 π \sqrt{\frac{d^{3}}{3 G m}}$
C $\frac{1}{2 π} \sqrt{\frac{3 G m}{d^{3}}}$
D $2 π \sqrt{\frac{3 G m}{d^{3}}}$
In a double star system one of mass $m_{1}$ and another of mass $m_{2}$ with a separation d rotate about their common centre of mass. Then rate of sweeps of area of star of mass $m_{1}$ to star of mass $m_{2}$ about their common centre of mass is
A $\frac{m_{1}}{m_{2}}$
B $\frac{m_{2}}{m_{1}}$
C $\frac{m_{1}^{2}}{m_{2}^{2}}$
D $\frac{m_{2}^{2}}{m_{1}^{2}}$
In a binary star system one star has thrice the mass of other. The stars rotate about their common centre of mass then :
ABoth stars have same angular momentum about common centre of mass
BBoth stars have angular momentum of same magnitude about common centre of mass
CBoth stars have same angular speeds
DBoth stars have same linear speeds