Three particle each of mass m, are located at the vertices of an equilateral triangle of side a. At what speed must they move if they all revolve under the influence of their gravitational force of attraction in a circular orbit circumscribing the triangle while still preserving the equilateral triangle ?
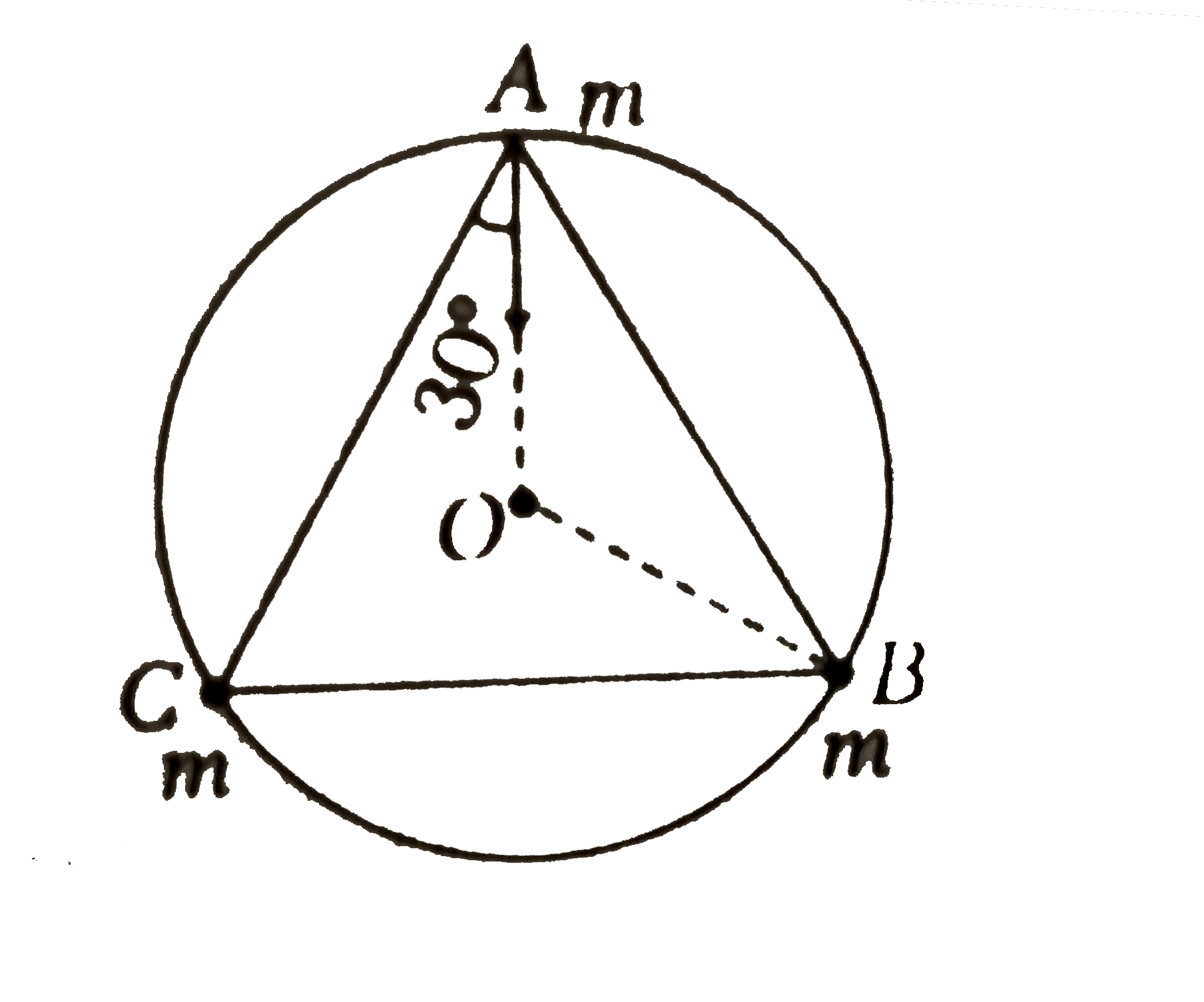
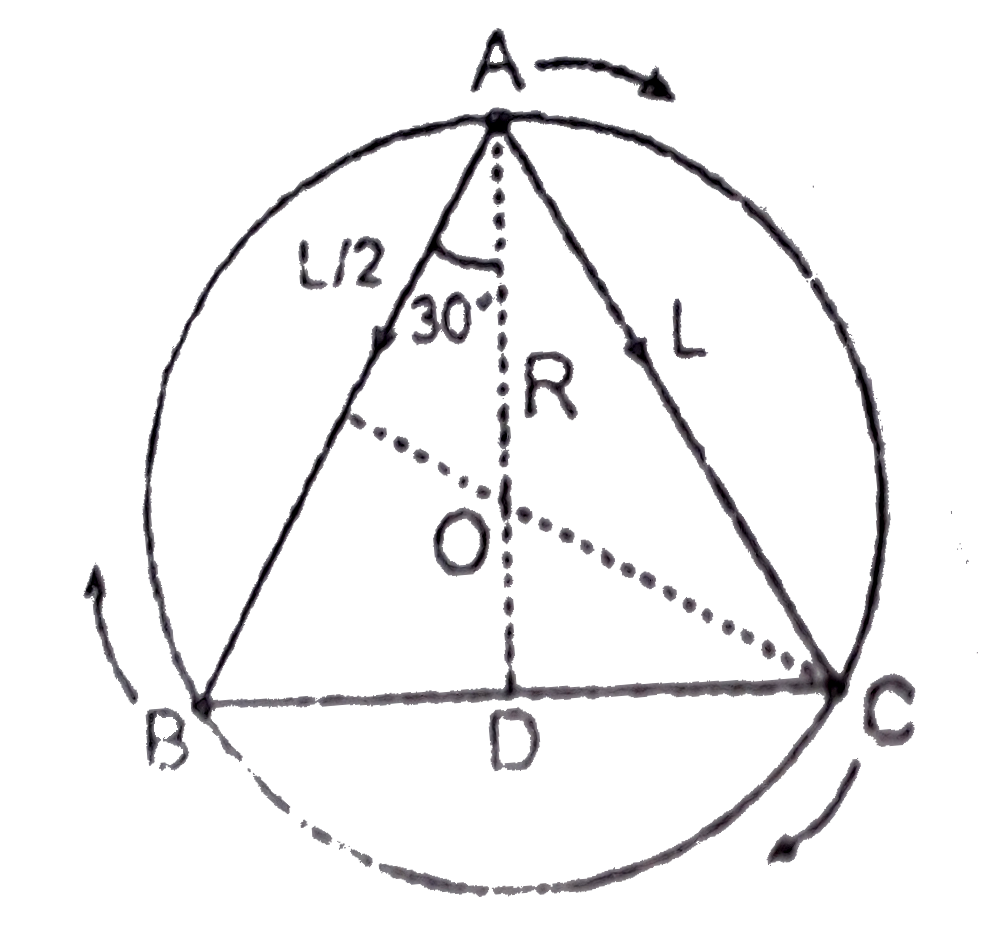
Let A,B and C be the three masses and O the centre of the circumscribing circle The radius of this circle is
R=L2sec30∘=L2×2√3=L√3
let v be the speed of each mass M along the circle Let us consider the motion of the mass at A The force of gravitational attraction on it due to the masses at B and C are
GM2L2 along AB and GM2L2 along AC
The resultant force is therefore
2GM2L2cos30∘=√3GM2L2alongAD
This for preserving the triangle must be equal to the neceassary centripetal force That is
√3GM2L2=Mv2R=√3Mv2L[∵R=L/√3] or v=√GML
 .
.
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - EXAMPLE2videos
GRAVITATION
- BOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - EXERCISE-1 PART-113videos
GRAVITATION
- BOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - HIGH LEVEL PROBLEMS16videos
GRAVITATION
- BOOK - RESONANCECHAPTER - GEOMATRICAL OPTICS EXERCISE - Advance level Problems35videos
GEOMATRICAL OPTICS
- BOOK - RESONANCECHAPTER - NUCLEAR PHYSICSEXERCISE - Advanced level solutions16videos
NUCLEAR PHYSICS
Similar Questions
Three particles each of mass m=1 kg, are located at the vertices of an equilateral triangle of side a =4m. The speed at which they must move to revolve in a circular orbit, under the inluence of mutual gravitational force, circumscribing and preserving the equilateral triangle is v=n√G, then the value of n is
View SolutionFour identical particles of mass M are located at the corners of a square of side ‘a’. What should be their speed if each of them revolves under the influence of others’ gravitational field in circular orbit circumscribing the square ?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Three particles each of mass m are kept at vertices of an equilateral triangle of side L. The gravitational field at centre due to these particle is
AzeroB3GML2C9GML2D12GM√3L2 - Question 2 - Select One
Three indentical bodes of mass M are locatd at the verticles of an equailateral triangle of side L they revolve undr the effect of mutual gravitational force in a circular orbit circumscibing the traingle while preserving the equilateral triangle their orbital velocity is
A√GMLB√3GM/(2L)C√3GMLD√2GM3L - Question 3 - Select One
Three particles each of mass m are kept at the vertices of an euilateral triangle of side L. The gravitational field at the centre due to these particle is
A−3GMLB−√3GMLC−3√3GMLD−GM3√3L
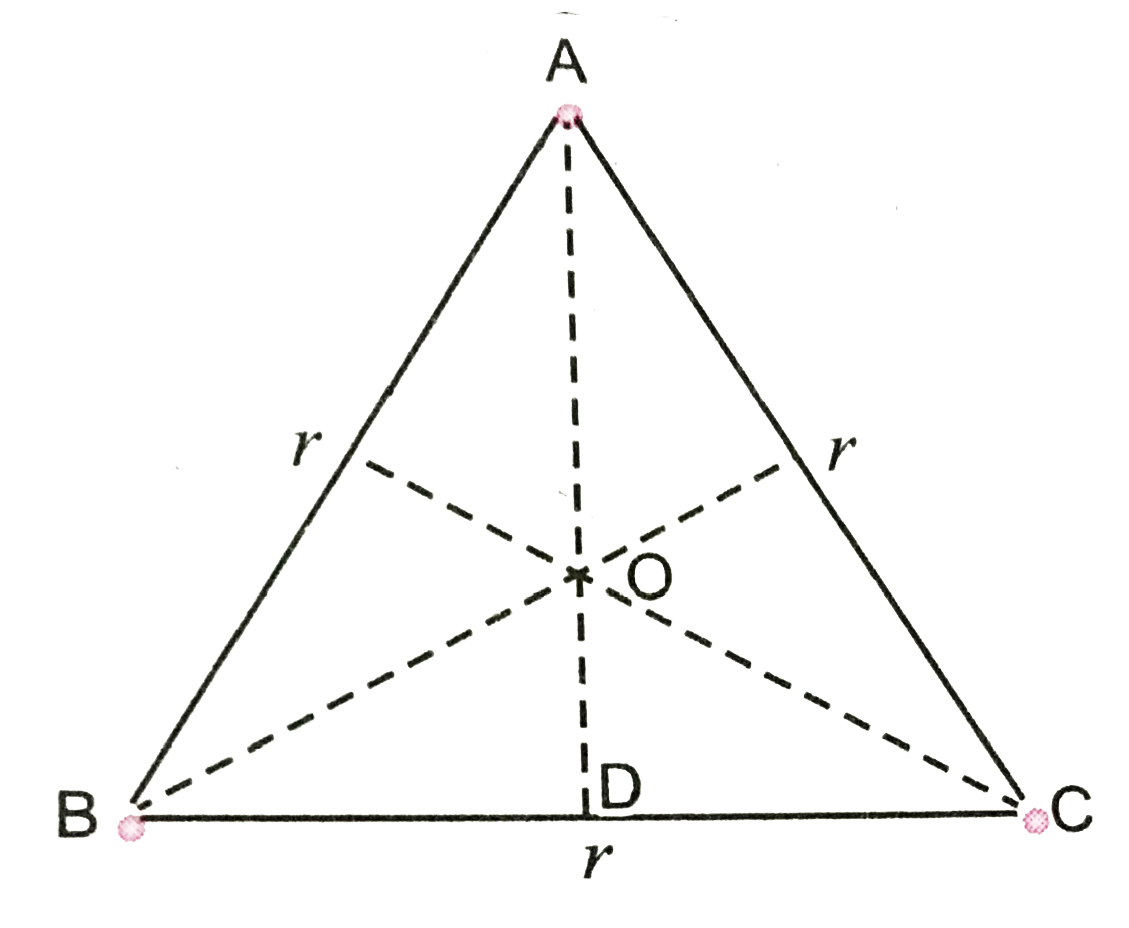
Three particles, each of mass m are placed at the vertices of an equilateral triangle of side a. What are the gravitation field and gravitational potential at the centroid of the triangle.
View SolutionThere are three identical point mass bodies each of mass m locted at the vertices of an equilateral triangle with side r. They are experting gravitational force of attraction on each other, which can be given by Newton's law of gravitaiton. Each mass body produces its gravitational field in the surrounding region. the magnitude of gravitational field at a point due to a point mass body is the measure of gravitaitonal intensity at that point. The gravitational potential at a point in a gravitational field is the amount of workdone in bringing a unit mass body infinity to the given point without acceleration.
Answer the following questions :
At what speed must they move if they all revolve under the influence of one another's gravitation in a circular orbit circumsribing the triangle still preserving the equilateral triangleView SolutionThree identical particles each of mass "m" are arranged at the corners of an equiliteral triangle of side "L". If they are to be in equilibrium, the speed with which they must revolve under the influence of one another's gravity in a circular orbit circumscribing the triangle is
View SolutionThree particle each of mass m are placed at the corners of equilateral triangle of side l

Which of the following is/are correct ?View SolutionThree particles each of mass m are kept at the vertices of an euilateral triangle of side L. The gravitational field at the centre due to these particle is
View Solution

