Let V and E denote the gravitational potential and gravitational field at a point. Then, match the following columns
Column-IColumn-II(A)E=0,V=0(p)At centre of spherical shell(B)E≠0,V=0(q)At centre of solid sphere(C)V≠0,E=0(r)At centre of circular ring(D)V≠0,E≠0(s)At cetre of two point masses of equal magnitude(t)None
The correct Answer is:A, B, C, D
Step by step video, text & image solution for Let V and E denote the gravitational potential and gravitational field at a point. Then, match the following columns {:(,"Column-I",,"Column-II"),("(A)",E = 0","V = 0,"(p)","At centre of spherical shell"),("(B)",E ne0","V=0,"(q)","At centre of solid sphere"),("(C)",V ne0","E=0,"(r)","At centre of circular ring"),("(D)",V ne0","E ne0,"(s)","At cetre of two point masses of equal magnitude"),(,,"(t)","None"):} by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - EXERCISE-2 PART-116videos
GRAVITATION
- BOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - EXERCISE-2 PART-210videos
GRAVITATION
- BOOK - RESONANCECHAPTER - GRAVITATIONEXERCISE - EXERCISE-1 PART-221videos
GRAVITATION
- BOOK - RESONANCECHAPTER - GEOMATRICAL OPTICS EXERCISE - Advance level Problems35videos
GEOMATRICAL OPTICS
- BOOK - RESONANCECHAPTER - NUCLEAR PHYSICSEXERCISE - Advanced level solutions16videos
NUCLEAR PHYSICS
Similar Questions
In the diagram shown in figure mass of both the balls in same. Match the following columns.
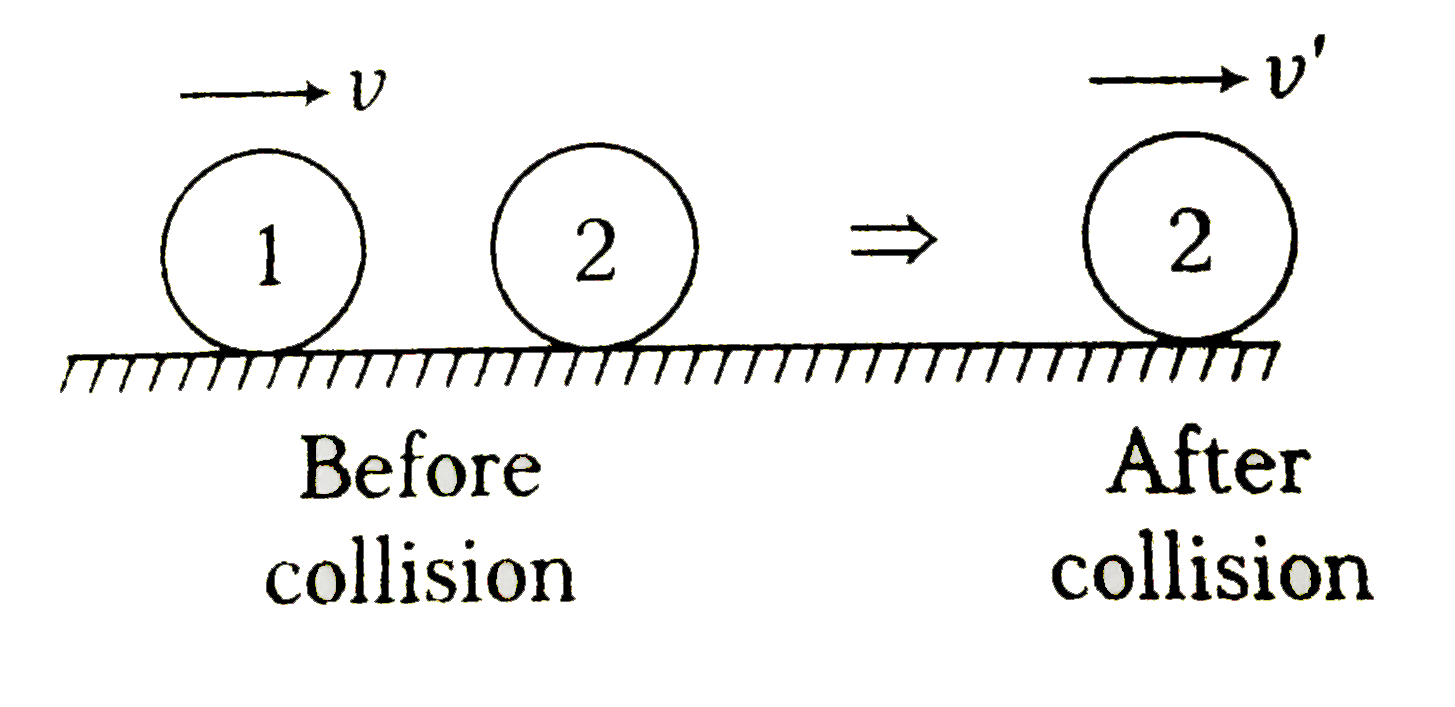
Column1Column2A Forv′=vP e=0B Forv′=v/2Q e=1C Forv′=3/4vR e=1/2-(S data is insufficientView SolutionA disc rolls on ground without slipping. Velocity of centre of mass is v. There is a point P on circumference of disc at angle θ. Suppose, vP is the speed of this point. Then, match the following columns
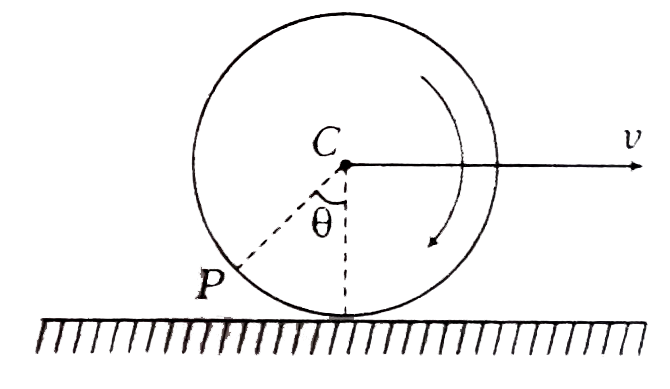
Column-IColumn-II(A)Ifθ=60∘(p)vP=√2v(B)Ifθ=90∘(q)vP=v(C)Ifθ=120∘(r)vP=2vD)Ifθ=180∘(s)vP=√3vView Solution
Knowledge Check
- Question 1 - Select One or More
Let V and E denote the gravitational potential and gravitational field at a point. It is possile to have
AV=0andE=0BV=0andE≠0CV=0andE=0DV≠0andE≠0 - Question 2 - Select One
Let V0 be the potential at the origin in an electric field →E=Exˆi+Eyˆj. The potential at the point (x,y) is
AV0−xEx−yEyBV0+xEx+yEyCxEx+yEy−V0D(√x2+y2)√E2x+E2y−V0 - Question 3 - Select One
Given that the gravitation potential on Earth surface is V0. The potential at a point distant half the radius of earth from the cetre will be
A114V0BV02C2V0D118V0
In the circuit shown in figure, E = 10 V, r = 1Ω,
R = 4Ω and L = 5H. The circuit is closed at time t = 0.
Then, match the following two columns.
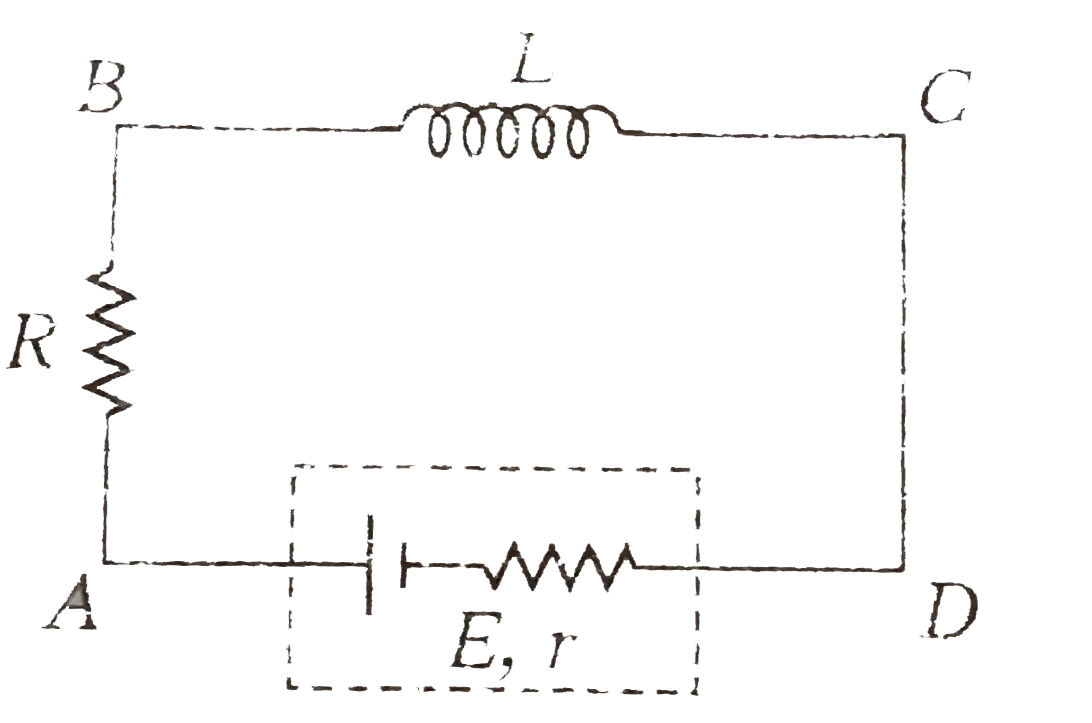
Column IColumn IIA.VAD at t = 0p4VB.VBC at t = 0q8VC.VAB at t =∞r.0VD.VAD at t=∞s.10VView SolutionMatch the following
Column IColumn II(A)E∝1r2(p)Point charge(B)E∝r(q)Spherically symmetric charge distribution(C)V∝1r(r)Long line charge(D)V2−V1=f(r2r1)(s)Plane sheet of charge(E)V2−V1∝r2−r1(t)Electric dipoleView SolutionColumn IColumn II(A)R/L(p)Time(B)C/R(q)Frequency(C)E/B(r)Speed(D)√ε0μ0(s)None
View SolutionColumn I shows charge distribution and column II shows electrostatic field created by that charge at a point r distance from its centre.
(,Column I ,,Column II),((A),A stationary point charge,(p),E prop r^(0))),((B),A stationary uniformly long charge rod,(q),Eprop r^(1)),((C ),Astationarye≤ctricdipo≤,(r ),E∝r⋅−1),(s),E∝r−2),(,,(t),E∝r−3):}View SolutionLet V and E represent the gravitational potential and field at a distance r from the centre of a uniform solid sphere. Consider the two statements.
A. the plot of V agasinst r is discotinuous
B. The plot of E against r is discontinuous.View Solution

