v epsilon(o)= (N-2n) epsilon, r(o) = Nr

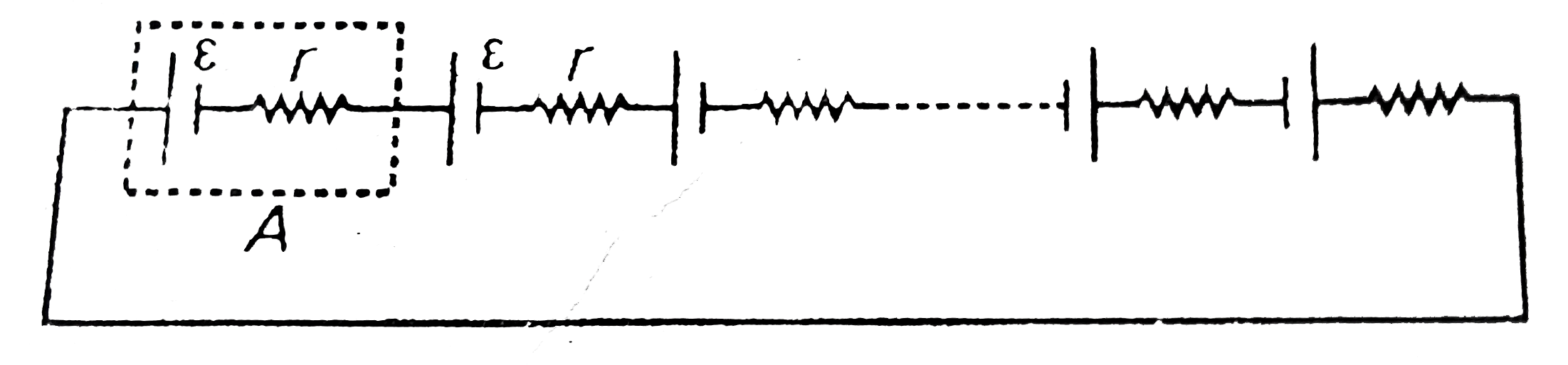
N identical cells, each of emf E and internal resistance r are joined in series. Out of N cells, n cells are wrongly connected i.e., their terminals are connected in reverse of that required for series connection. $(n < \frac{N}{2})$ . Let $E_{0}$ be the emf of resulting battery and $r_{0}$ be its internal resistance. then
A $E_{0} = (N - n) E, r_{0} = (N - n) r$
B $E_{0} = (N - 2 n) E, r_{0} = (N - 2 n) r$
C $E_{0} = (N - 2 n) E, r_{0} = N r$
D $E_{0} = (N - n) E, r_{0} = N r$
$N$ identical cells, each emf $E$ and internal resistance $r$ are joined in series. Out of $N$ cells, $n$ cells are wrongly connected i.e., their terminals are connected in reverse of the required for series connection $(n < \frac{N}{2})$ . Let $E_{0}$ be the emf of resulting battery and $r_{0}$ be its internal resistance. Then
A $E_{0} = (N - n) E, r_{0} = (N - n) r$
B $E_{0} = (N - 2 n) E, r_{0} = (N - 2 n) r$
C $E_{0} = (N - 2 n) E, r_{0} = N r$
D $E_{0} = (N - n) E, r_{0} = N r$
In a arrangement, 3n cells of emf $ε$ and internal resistance r are connected in series. Out of 3n cells polarity of n cells is reversed. Current in the circuit is

A $\frac{2 ε l o}{r}$
B $\frac{2 ε}{3 r}$
C $\frac{ε}{3 r}$
D $\frac{ε}{r}$

The correct Answer is:C