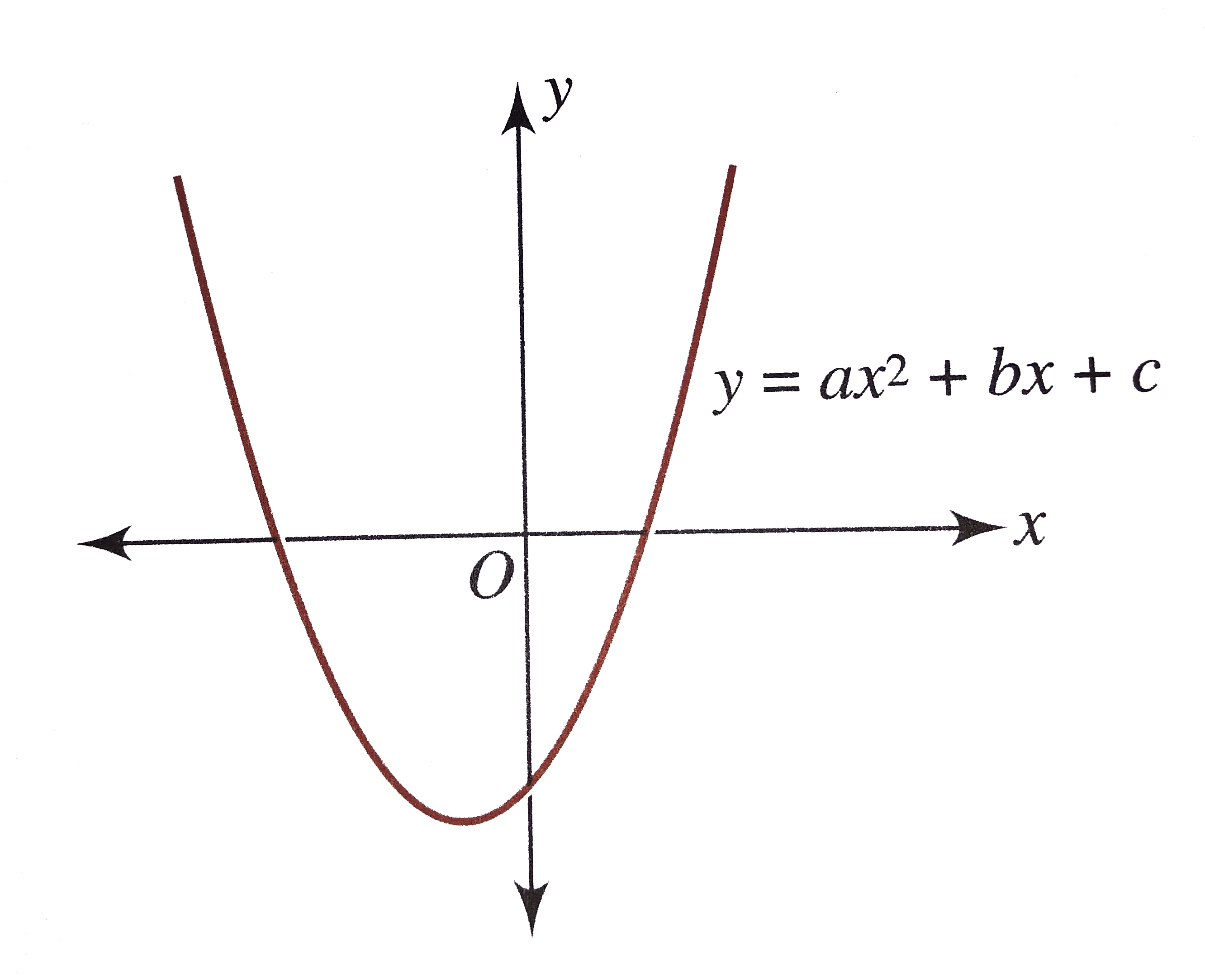The following figure shows the graph of f(x)=ax2+bx+c, find the sign of a,bandc.
Fromt the graph, f(0)=c>0 (as the graph intersects the y-axis on the positive side)
Also the graph is concave downwards, so a<0.
Further, the vertex lies on the right-hand side of y-axis, so the abscissa of the vertex is −b2a>0⇒b> (as a<0).
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise34videos
GRAPHS OF ELEMENTARY FUNCTIONS
- BOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercise13videos
GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONS
- BOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
If f(x)=ax2+bx+c find f1(1).
Aa+2bB2a−bCa−2bD2a+b
If fig shows the graph of f(x)=ax2+bx+c,then Fig ac<0 b. bc>0 c. ab>0 d. abc<0
View SolutionConsider the graph of f(x)=ax2+bx+c in the adjacent figure. We can conclude that
View SolutionIf f(x)=ax2+bx+c,a+b+c=0 then one root is
View SolutionFor following graphs of y=ax2+bx+cwith a,b,c c R, comment on the sign of
View SolutionThe given figure shows the graph of the polynomial f(x)=ax2+bx+c, then
(1) a>0, b<0 and c>0
(2) a>0, b<0 and c<0
(3) a<0, b>0 and c>0
(4) a<0, b>0 and c<0View SolutionWrite the following equations in the form ax2+bx+c=0. Also, find the value of a,b and c.
(ii) x2−3x=−1.View SolutionWrite the following equations in the form ax2+bx+c=0. Also, find the value of a,b and c.
(iii) (x+5)(x−1)=6View Solution