Solve : $x^{2} - | x + 2 | + x > 0$

Video Solution

Text Solution

Verified by Experts

We have $x^{2} - | x + 2 | + x > 0$
or $x^{2} + x > | x + 2 |$ (i)
To solve the inequality, we draw the graph of $y = x^{2} + x and y = | x + 2 |$ as shown in the following figure.

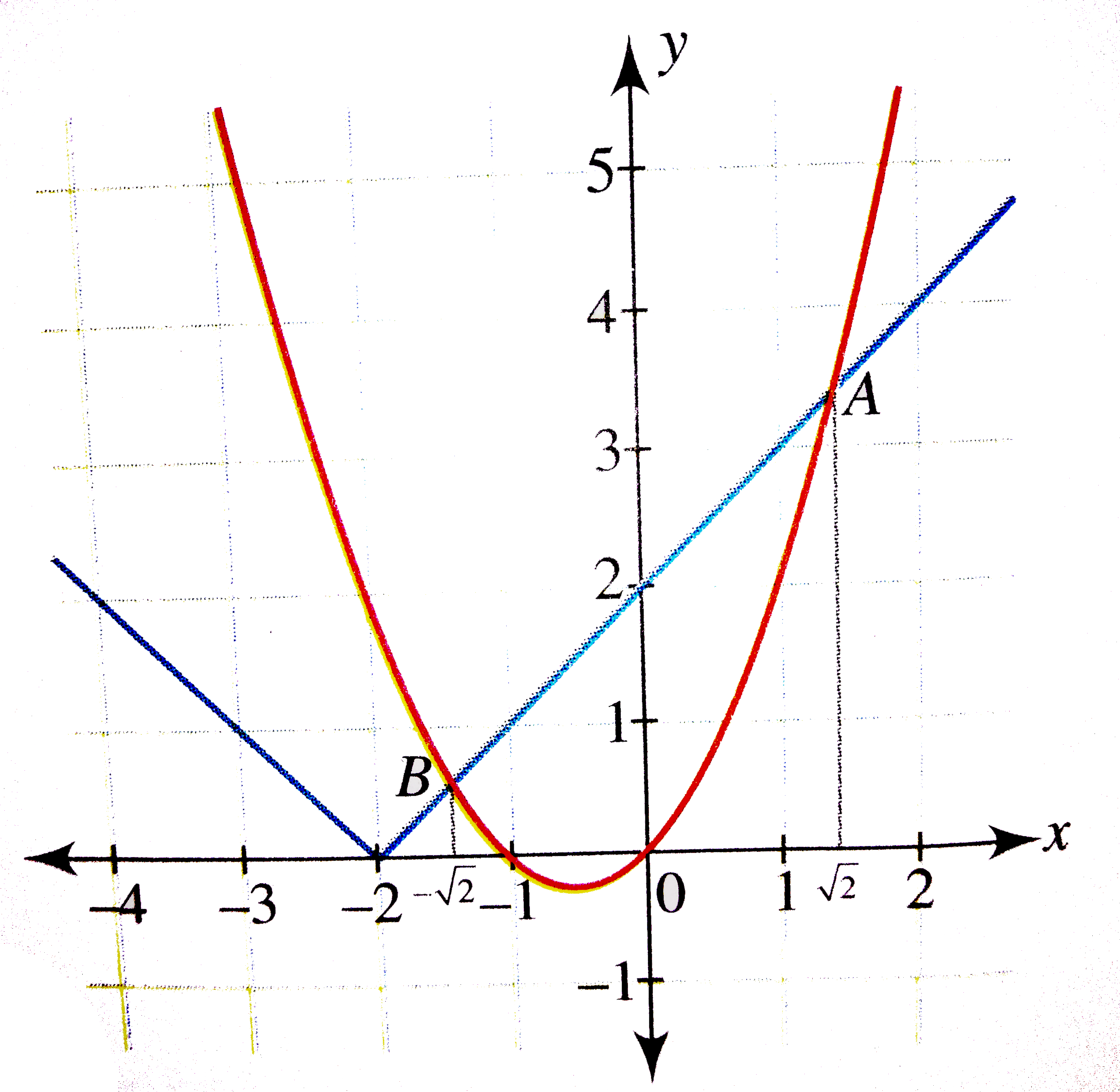
Solving $y = | x + 2 | and y = x^{2} + x$ for their points of intersection A and B, we have $x + 2 = x^{2} + x \Rightarrow x^{2} = 2 \Rightarrow x = \pm \sqrt{2}$
The solution set of (i) is the set of those values of x for which the graph of $y = x^{2} + x$ lies above the graph of $y = x + 2$ .
From the graph, the solution is $(- \infty, - \sqrt{2}) \cup (\sqrt{2}, \infty)$ .

|

Updated on:21/07/2023

Class 12 MATHS FUNDAMENTAL OF MATHEMATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Solve $x^{2} - x - 2 > 0 .$
A $x \in (- \infty, - 1) \cup (2, \infty)$
B $x \in (\infty, 2)$
C $x \notin (- \infty, - 1) \cup (2, \infty)$
D $x \notin (\infty, 2)$

CENGAGE-GRAPHS OF ELEMENTARY FUNCTIONS -Exercise

Home
Ask Doubt
Profile